重心

通常在处理力学和重力时，工程师和科学家会遇到不指向质量的固体。这样的物体尺寸更大，在计算中不能被视为点质量。在这种情况下，假设存在一个物体的所有质量都集中的某个点，并且重力也作用在某个点上。这个假设使我们能够简化复杂的计算。这些点分别称为质心和重心。让我们详细看看这些概念。

重心

理论上，重心被认为是身体重量作用的点。对于具有大尺寸且质量分布通常不均匀的物体，找到这一点非常重要。这一点使我们能够预测这些物体在重力影响下的运动。如果一个物体在这一点上达到平衡，它就是旋转和平移平衡。考虑下图中给出的主体示例，

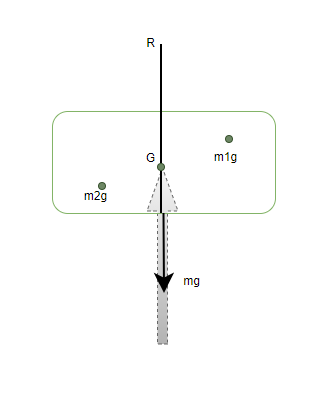
该图显示纸板被放在它下面的铅笔平衡。铅笔的尖端在使纸板保持平移平衡的点上施加反作用力。它也处于旋转平衡状态，可以通过物体没有任何角加速度的事实来验证。让我们假设纸板由非常小的单个点质量 m ₁ 、 m ₂ 、 m ₃ ...

重力作用在所有这些点质量上，在点周围产生扭矩。重心 (CG) 的位置使得由于单个点质量上的力而在 CG 上的总扭矩为零。如果 r _i是第 i 个粒子相对于 CG 的位置矢量，那么由于重力作用在其上的扭矩由下式给出，

$\tau_i = \vec{r_i} \times m_ig$

对于重心点，关于该点的总扭矩必须为零，

$\sum \tau_i =\sum \vec{r_i} \times m_ig = 0$

请注意，在上面的等式中，重力“g”对于每个点都是相同的。它可以作为所有术语的共同因素从等式中出来。

$g\sum \vec{r_i} \times m_i = 0$

因为 g 是常数且非零。可以得出结论，

$\sum \vec{r_i} \times m_i = 0$

质心和重心

它的位置是相对于要计算其质心的对象或对象系统定义的。通常对于统一的形状，它是它们的质心。让我们从简单的形状开始，看看它们的质心在哪里。考虑下图中给出的形状。很容易猜出以下形状的质心。对于他们中的大多数人来说，质心位于他们的质心。

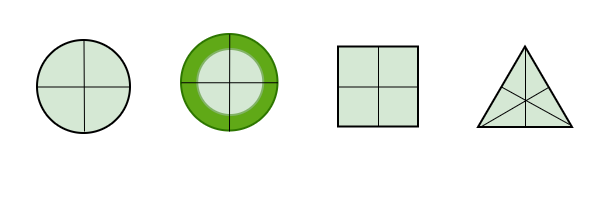
请注意，对于环来说，它的质心位于环内，这意味着物体的质心必须位于物体本身。现在，很明显，均匀对称的物体的质心位于质心。但对于不对称和不均匀的物体，答案并不那么简单。这种物体的质心可以在任何地方。计算复杂物体的质心。取每个身体质量的位置的加权平均值。

Let’s say there is a body consisting of a set of masses “m_i“, each at position r_i relative to the location of the Centre of mass.

⇒ r_cm =  $\sum m_i \times r_i$

请注意，在重力恒定的情况下，质心和重心的位置相同。如果一个物体如此伸展，以至于作用在它不同部位的重力的值是不同的。那么公因数“g”不能取出，质心的位置不同于重心。

示例问题

问题 1：两个点质量 m ₁ = 5Kg 和 m ₂ = 2Kg 分别位于 x = 2 m 和 x = 6 m。找到重心。

解决方案：

The formula for the Centre of gravity is equal to centre of mass when gravity is constant

x_cm =  $\frac{ m_1x_1 + m_2x_2 + ...}{M}$

 m₁ = 5Kg,  m₂ = 2Kg and x = 2 m and x = 6 m. 

M = m₁ + m₂

⇒ M = 5 + 2 = 7

x_cm =  $\frac{ m_1x_1 + m_2x_2 + ...}{M}$

⇒ x_cm =  $\frac{ m_1x_1 + m_2x_2}{M}$

⇒ x_cm =  $\frac{ (5)(2) + (2)(6)}{7}$

⇒ x_cm =  $\frac{22}{7}$

问题 2：两个点质量 m ₁ = 5Kg 和 m ₂ = 2Kg 分别位于 y = 10m 和 y = -5 m。找到重心。

解决方案：

The formula for the Centre of gravity is given by, 

_ycm =  $\frac{ m_1y_1 + m_2y_2 + ...}{M}$

 m₁ = 5Kg,  m₂ = 2Kg and y = 10m and y = -5 m. 

M = m₁ + m₂

⇒ M = 5 + 2 = 7

y_cm =  $\frac{ m_1y_1 + m_2y_2 + ...}{M}$

⇒ y_cm =  $\frac{ m_1y_1 + m_2y_2}{M}$

⇒ y_cm =  $\frac{ (5)(10) + (2)(-5)}{7}$

⇒ y_cm =  $\frac{40}{7}$

问题 3：两个点质量 m ₁ = 1Kg 和 m ₂ = 2Kg 分别位于向量 a = 6i + 4j 和向量 b = -5i + 2j。找到重心。

解决方案：

The formula for the Centre of gravity in the vector notation is given by, 

r_cm =  $\frac{ m_1\vec{r_1} + m_2\vec{r_2} + ...}{M}$

 m₁ = 1Kg,  m₂ = 2Kg and a = 6i + 4j, b = -5i + 2j

M = m₁ + m₂

⇒ M = 1 + 2 = 3

r_cm =  $\frac{ m_1\vec{r_1} + m_2\vec{r_2} + ...}{M}$

⇒ r_cm =  $\frac{ m_1\vec{a} + m_2\vec{b}}{M}$

⇒ r_cm =  $\frac{ (1)(6\hat{i} + 4\hat{j} ) + (2)(-5\hat{i} + 2\hat{j})}{7}$

⇒ r_cm =  $\frac{ 6\hat{i} + 4\hat{j} + -10\hat{i} + 4\hat{j})}{7}$

⇒ r_cm =  $\frac{ -4\hat{i} + 8\hat{j} }{7}$

问题 4：两个点质量 m ₁ = 4Kg 和 m ₂ = 2Kg 分别位于向量 a = i + j 和向量 b = -i + j。找到重心。

解决方案：

The formula for the Centre of gravity in the vector notation is given by, 

r_cm =  $\frac{ m_1\vec{r_1} + m_2\vec{r_2} + ...}{M}$

 m₁ = 4Kg,  m₂ = 2Kg and a = i + j, b = -i + j

M = m₁ + m₂

⇒ M = 4 + 2 = 6

r_cm =  $\frac{ m_1\vec{r_1} + m_2\vec{r_2} + ...}{M}$

⇒ r_cm =  $\frac{ m_1\vec{a} + m_2\vec{b}}{M}$

⇒ r_cm =  $\frac{ (4)(\hat{i} + \hat{j} ) + (2)(\hat{i} -\hat{j})}{7}$

⇒ r_cm =  $\frac{ 4\hat{i} + 4\hat{j} + 2\hat{i} -2\hat{j})}{7}$

⇒ r_cm =  $\frac{ 6\hat{i} + 2\hat{j} }{7}$

问题 5：从质量为 M、半径为 R 的较大圆盘中移除一个半径为 R/2 的圆盘。求重心。

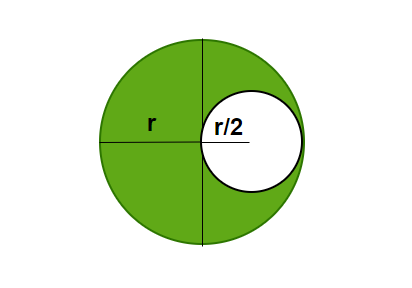
解决方案：

Since the density of the disk is uniform, the weight is uniformly distributed over all the area. 

Mass “m” of the removed disk = 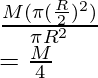$\frac{M (\pi (\frac{R}{2})^2)}{\pi R^2} \\ = \frac{M}{4}$

Let the distance of the center of mass of the remaining portion be “x”. 

$0 = \frac{x\frac{3M}{4} + \frac{R}{2}\frac{M}{4}}{\frac{3M}{4} + \frac{M}{4}} \\ = 0 = x\frac{3M}{4} + \frac{RM}{8} \\ = -\frac{RM}{8} = x\frac{3M}{4} \\ = x = \frac{-R}{6}$

问题 6：半径为 R/4 的圆盘从质量为 M、半径为 R 的较大圆盘中移除，方法与上图相同。找到重心。

解决方案：

Since the density of the disk is uniform, the weight is uniformly distributed over all the area. 

Mass “m” of the removed disk = 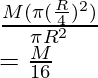$\frac{M (\pi (\frac{R}{4})^2)}{\pi R^2} \\ = \frac{M}{16}$

 Let the distance of the center of gravity of the remaining portion be “x”. 

$0 = \frac{x\frac{15M}{16} + \frac{R}{2}\frac{M}{16}}{\frac{15M}{16} + \frac{M}{16}} \\ = 0 = x\frac{15M}{16} + \frac{RM}{32} \\ = -\frac{RM}{32} = x\frac{15M}{16} \\ = x = \frac{-R}{30}$