点电荷产生的电场
电荷是物质的基本属性,它控制电场或磁场如何影响基本粒子。电荷存在于无法产生或破坏的离散自然单元中。正电荷和负电荷是两种类型的电荷。当具有超过一种电荷的两个项目足够靠近时,它们会相互排斥。当两个带正电和带负电的物体紧密接触时,它们会相互吸引。
许多基本的或亚原子的材料粒子都具有电荷的特性。质子带正电荷,而电子带负电荷。另一方面,中子是不带电或中性粒子。根据实验,每个电子的负电荷和每个质子的正电荷具有相同的大小。电子或质子的电荷是基本物理常数,以自然单位测量。
点电荷产生的电场
考虑原点 O 处的点电荷 Q,它被放置在真空中。库仑定律指出,如果另一个点电荷 q 放置在 OP = r 的位置 P 上,电荷 Q 将对 q 施加一个力。电荷 Q 产生一个在整个环境中延伸的电场。当一个新的电荷 q 在点 P 引入时,那里的场作用在它上面并产生一个力。由电荷 Q 在位置 r 产生的电场可以表示为:
![]()
其中 r = r/r 是从原点到点 r 的单位向量。结果,上述表达式为位置矢量r的每个值指定了电场值。术语“场”是指一些分布量(可以是标量或向量)如何随位置变化。电场的存在与电荷的作用相结合。电荷 Q 对电荷 q 施加的力 F 计算为
![]()
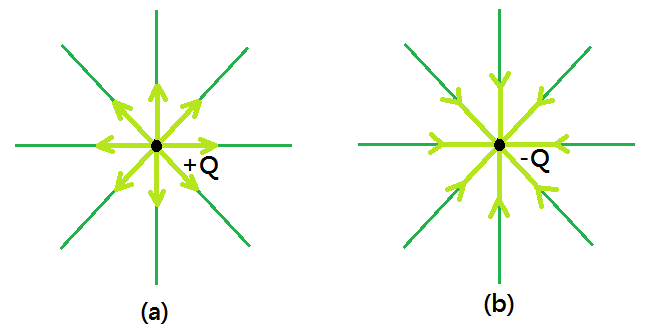
电场(a)由于电荷 Q,(b)由于电荷 -Q。
值得注意的是,电荷 q 对电荷 Q 具有相等且相反的力。
电荷 Q 和 q 之间的静电力可以看作是电荷 q 和 Q 的电场之间的相互作用,反之亦然。如果电荷 q 的位置由矢量 r 表示,则电荷 q 在 q 的位置处感受到的力 F 等于电荷 q 乘以电场 E。
所以,
F(r) = q E(r)
电场的 SI 单位为 N/C。
以下是需要考虑的几个关键点:
- 如果 q 为 1,则电荷 Q 产生的电场在数值上等于它所施加的力。因此,由电荷 Q 在空间中给定位置产生的电场可以描述为如果将单位正电荷放置在那里将受到的力。产生电场的电荷 Q 称为源电荷,而测量源电荷效应的电荷 q 称为测试电荷。重要的是要记住源电荷 Q 必须保持在其原始位置。但是,如果将电荷 q 施加到 Q 周围的任何位置,Q 将受到 q 的影响并开始移动。使 q 可以忽略不计是解决这个问题的一种方法。因此力 F 小到可以忽略不计,但比率 F/q 是有限的,电场定义如下:
![]()
- 值得注意的是,由 Q 引起的电场 E 与 q 无关,尽管它在操作上是根据一些测试电荷 q 来描述的。这是因为 F 与 q 成比例,因此 F/q 比率与 q 无关。电荷 Q 对电荷 q 施加的力 F 由电荷 q 的特定位置决定,该位置可以是电荷 Q 周围区域中的任何值。因此,由 Q 产生的电场 E 同样受空间坐标r。对于跨空间的电荷 q 的不同位置,我们接收到不同的电场 E 值。三维空间中的每个点都包含场。
- 来自正电荷的电场将向外辐射。另一方面,如果源电荷为负,则每个点的电场矢量径向向内。
- 由于电荷 Q 对电荷 q 的作用力 F 的大小仅由电荷 q 与电荷 Q 的距离 r 决定,因此电场 E 的大小也仅由距离 r 决定。结果,电荷 Q 的电场 E 的大小在与它相等的距离处是相同的。因此,在以点电荷为中心的球面上,点电荷产生的电场 E 的大小是相同的;换言之,它具有球对称性。
电荷系统产生的电场

由电荷系统引起的点处的电场是由单个电荷引起的点处的电场的矢量和。
考虑一个电荷系统 q 1 , q 2 ,..., qn ,其位置矢量为 r 1 , r 2 ,..., r n相对于某个原点 O。单元测试电荷在该点处所经受的力,不改变电荷的原始位置 q 1 , q 2 ,…, q n被描述为由于电荷系统而在空间中的一点处的电场,类似于由于单个电荷在空间中的点处的电场。为了确定由位置矢量 r 表示的位置 P 处的该场,我们可以应用库仑定律和叠加原理。
由于 r 1处的 q 1引起的电场 E 1在 r 处可以写成,
![]()
在哪里![]() 是从q 1到P 方向的单位向量,r 1P是q 1和P 之间的距离。
是从q 1到P 方向的单位向量,r 1P是q 1和P 之间的距离。
类似地,由于 r 2处的 q 2引起的电场 E 2在 r 处可以表示为,
![]()
在哪里![]() 是从 q 2到 P 的方向上的单位向量,r 2P是 q 2和 P 之间的距离。由于电荷 q 3 , q 4 , ..., q 的场 E 3 , E 4 , ..., E n的类似表达式ñ 。根据叠加原理,电荷系统在 r 处的电场 E 可以表示为:
是从 q 2到 P 的方向上的单位向量,r 2P是 q 2和 P 之间的距离。由于电荷 q 3 , q 4 , ..., q 的场 E 3 , E 4 , ..., E n的类似表达式ñ 。根据叠加原理,电荷系统在 r 处的电场 E 可以表示为:

E 是一个向量变量,它从空间中的一个位置到另一个位置变化,由源电荷位置决定。
电场的物理意义
The concept of the electric field is useful in electrostatics, but it isn’t absolutely necessary. An elegant approach to describing the electrical environment of a system of charges is to use the term electric field.
如果将单位正测试电荷放置在电荷系统周围空间中的某个位置(不扰乱系统),将受到的力由该点的电场决定。电场是电荷系统的一种性质,与用于计算电场的测试电荷无关。在物理学中,场是在空间的每个点上定义的量,并且可以从一个点到另一个点变化。因为力是矢量,所以电场是矢量场。
然而,当我们超越静电并考虑与时间相关的电磁事件时,电场概念的实际物理相关性就出现了。考虑加速运动中两个远距离电荷 q 1和 q 2之间的力。信号或信息从一个位置传播到另一个位置的速度现在等于 c,即光速。因此,任何 q 1对 q 2的运动都不会产生瞬时影响。在结果(q2 上的力)和原因(q1 的运动)之间,会有一个时间延迟。电场(严格地说,电磁场)的概念在这种情况下是直观且非常有用的。场图如下:电荷 q1 的加速运动产生电磁波,电磁波在 c 处传播,到达 q2,并对 q2 施加一个力。时间延迟可以用场的概念优雅地解释。尽管电场和磁场只能通过它们对电荷的影响(力)来识别,但它们被认为是物理事物而不是数学抽象。它们有自己的动态,即,它们根据自己的一套规则发展。它们还能够传输能量。因此,时间相关的电磁场源会在短时间内打开然后关闭,从而留下传输能量的传播电磁场。法拉第是第一个建立场概念的人,它是当今物理学中最重要的概念之一。
示例问题
问题 1:两个带电荷的粒子 +4 μC 和 -9 μC 保持固定,彼此相距 20 cm。找出由于两点电荷系统而产生的电场为零的点。
解决方案:
The null point is the point at which the resultant electric field owing to the given system of point charges is zero. We know that in a system with two point charges, the null point is on the line connecting the two charges, and that the null point is always closer to the lower magnitude charge. The null point will not be in the space between the charges since the two charges are of opposite nature.

Assume that the electric field at point P is zero. The point P is at a distance of x from the +4C charge. The field vanishes at point P, hence the magnitudes of the separate fields produced by the two point charges at P must be equal (and directions anti-parallel).
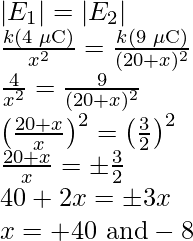
Because there is no chance of a null point in the region between the two charges, the negative value of x should be ignored. As a result, the electric field’s zero point is located on the line connecting the two point charges, 40 cm to the left of the positively charged particle.
问题2:点电荷-2μC位于A(2,2,2)点,求B(1,1,1)点的电场强度矢量。
解决方案:
Electric field intensity vector due to a point charge q at a position r can be expressed as,
![]()
The position vector of the point of calculation of the electric field with regard to the location point of the source point charge is r, and the proper sign is q.

Therefore, electric field at point B is
![]()
Substitute the value in the above formula,
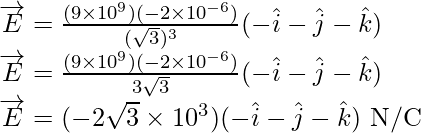
问题 3:两个电荷分别为 2 x 10-7 C 和 3 x 10-7 C 的带电小球在空气中相距 30 厘米时的作用力是多少?
解决方案:
Given,
The magnitude of repulsive force is 6 × 10-3 N.
Charge on the first sphere, q1 is 2 × 10-7 C.
Charge on the second sphere, q2 is 3 × 10-7 C.
Distance between the spheres, r is 30 cm i.e. 0.3 m.
The expression for the electrostatic force between the spheres can be written as,
![]()
Substitute the value in the above formula,
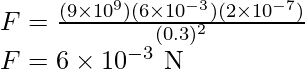
Therefore, force between the two small charged spheres is 6 × 10–3 N. The charges are of same nature. Hence, force between them will be repulsive.
问题 4:解释“物体的电荷被量子化”这句话的含义。
解决方案:
A body’s electric charge is quantized. This means that only an integral number of electrons (1, 2,…, n) can be transported from one substance to another. The transfer of charges is not fractional. As a result, a body can only have total charge in integral multiples of electric charge.
The charges used in macroscopic or large-scale charges are enormous in comparison to the magnitude of electric charge. As a result, on a macroscopic scale, quantization of electric charge is useless. As a result, it is overlooked, and it is assumed that electric charge is constant.
问题 5:当用丝布摩擦玻璃棒时,两者都会出现电荷。在许多其他天体对中也观察到了类似的现象。解释这个观察结果如何与电荷守恒定律相一致。
解决方案:
Because charges are formed in pairs, rubbing produces charges of equal magnitude but opposing nature on the two bodies. Charging by friction is the name for this type of charging. The system of two rubbed bodies has a net charge of zero. This is due to the fact that equal amounts of opposite charges cancel each other out. When rubbing a silk cloth over a glass rod, opposite natured charges develop on both bodies. These phenomena are in accordance with the law of energy conservation. Many other pairs of bodies exhibit a similar phenomena.