图中的悬垂顶点、非悬垂顶点、悬垂边和非悬垂边

先决条件：握手定理。

吊坠顶点

设G是一个图，当且仅当v的度数为 1时， G的顶点v称为悬垂顶点。换句话说，悬垂顶点是度数为 1的顶点，也称为悬垂顶点。

Note: Degree = number of edges connected to a vertex

在树的情况下，悬垂的顶点被称为终端节点或叶节点，或叶，因为它只有1度。请记住，叶节点只有 1 度，因此在树的情况下，下垂顶点称为叶节点。

示例：在给定的图中A和B是悬垂顶点，因为它们每个的度数为1 。

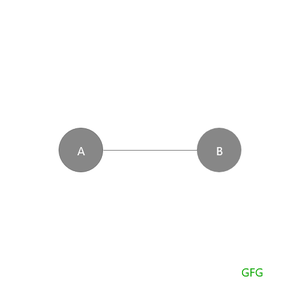
图 1.0

让我们以这个例子来打印图中所有的悬垂顶点。

C++

// C++ program for the above approach
#include 
using namespace std;
 
// Function to print all the pendant
// vertices
void printPendantVertices(map > graph)
{
 
    // All the vectors which contain only 1
    // vertex i.e, size 1 has only 1 edge
    // hence a pendant vertex.
    for (auto x : graph) {
        if (x.second.size() == 1) {
            cout << x.first << " ";
        }
    }
}
 
// Driver Code
int main()
{
 
    map > graph;
    graph['A'].push_back('B');
    graph['B'].push_back('A');
    graph['C'].push_back('B');
    graph['B'].push_back('C');
 
    printPendantVertices(graph);
 
    return 0;
}

Javascript

// Javascript program for the above approach
 
// Function to print all the pendant
// vertices
function printPendantVertices(graph) {
  // All the vectors which contain only 1
  // vertex i.e, size 1 has only 1 edge
  // hence a pendant vertex.
  for (x of graph) {
    if (x[1].length == 1) {
      document.write(x[0] + " ");
    }
  }
}
 
// Driver Code
 
let graph = new Map();
graph.set("A", ["B"]);
graph.set("B", ["A"]);
graph.set("C", ["B"]);
graph.set("B", [...graph.get("B")].push("A"));
 
printPendantVertices(graph);
 
// This code is contributed by saurabh_jaiswal.

输出

A C 

非悬垂顶点

非悬垂顶点是度数不是1的顶点。在树的情况下，非悬垂顶点是非叶节点，因为它没有度数1 （叶节点的度数为1 ）。

示例：在给定的图中A和C是悬垂顶点，因为A和C的度数为1 ， B 是非悬垂顶点，因为它包含的度数不是1 ，即2 。

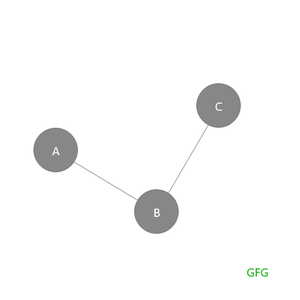
图 2.0

吊坠边缘

当且仅当其顶点之一是悬垂顶点时，图的边被称为悬垂边。

示例：在给定的图中AB是一个悬垂边，因为它包含悬垂顶点作为其顶点之一。

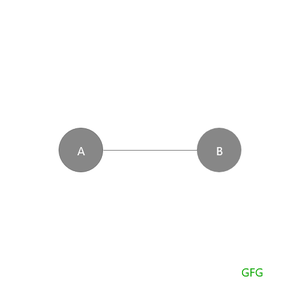
图 3.0

非吊边

如果图的边不包含作为其顶点之一的悬垂顶点，则称该边为非悬垂边。

示例：在给定的图中AB是一个悬垂边，因为它具有悬垂顶点 (A) 作为其顶点之一。 BD、BC、DC是非悬垂顶点。

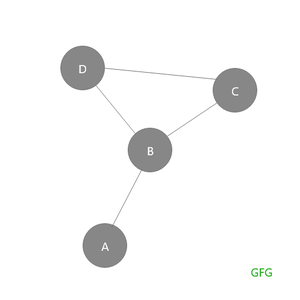
图 4.0

例子：

让我们看一些基于上述主题的示例：

Q1。假设一棵树 T 有 2 个度数为 2 的顶点、4 个度数为 3 的顶点和 3 个度数为 4 的顶点。求 T 中的悬垂顶点数。

Finding number pendant vertices is nothing but finding the number of leaf nodes.
         Let’s use the Handshaking Theorem formula 

         Sum of all degrees  = 2 * (Sum of Edges)

(2 vertices) * (2 degrees) + (4 vertices) * (3 degrees) + (3 vertices) * (4 degrees) + (k vertices) * (1 degree) = (2 * edges)

where 
          k is pendant vertices or leaf nodes which have degree 1
          e is total number of edges in the tree

        2*2 + 4*3 + 3*4 + k*1 = 2*e      ——-(1)

remember : number of edges = number of vertices – 1 

        e=(2+4+3+k)-1

        e=9+k-1

        e=8+k                                         ——-(2)

        putting equation 2 in 1 gives

        4 + 12 + 12 + k = 2(8+k)

        28 + k = 16 + 2k

       -2k + k = 16 – 28

       -k = -12

        k = 12

        so total number of pendant vertices are 12

Q2。如果一棵树 T 有 4 个 2 度的顶点、1 个 3 度的顶点和 2 个 4 度的顶点和 1 个 5 度的顶点。求 T 中的下垂顶点数。

Finding number pendant vertices is nothing but finding the number of leaf nodes.

        Let’s use the Handshaking Theorem formula

        Sum of all degrees  = 2 * (Sum of Edges)

(4 vertices)*(2 degrees) + (1 vertex)*(3 degrees) + (2 vertices)*(4 degrees) + (1 vertex)*(5 degree) + (k vertices)*(1 degree) = (2 * edges)

where
          k is pendant vertices or leaf nodes which have degree 1
          e is total number of edges in the tree

        4*2 + 1*3 + 2*4 + 1*5 + k*1 = 2*e      ——-(1)

        remember number of edges = number of vertices – 1

        e=(4+1+2+1+k)-1

        e=8+k-1

        e=7+k                                         ——-(2)

        putting equation 2 in 1 gives

        8 + 3 + 8 + 5 + k = 2(7+k)

       24 + k = 14 + 2k

       -2k + k = 14 – 24

       -k = -10

        k = 10

       so total number of pendant vertices are 10