LC 振荡

直流电和交流电之间的区别在于，直流电（DC）仅沿一个方向传播，而交流电（AC）是一种有时会改变方向并随时间连续改变其幅度的电流。交流电是供应给企业和住宅的电力类型，也是消费者将厨房电器、电视、风扇和电灯插入墙壁插座时使用的能源类型。

然而，手电筒的电池是典型的直流电源。在修改电流或电压时，缩写词 AC 和 DC 经常用于表示仅交流和直流。在大多数电力电路中，最常见的交流波形是正弦波，其正半周期与正电流方向相关，反之亦然。不同的波形，如三角波或方波，用于特定应用，如吉他放大器。交流电还包括通过电缆发送的音频和无线电信号。声音（音频）或图片（视频）等信息有时通过以这些交流电形式调制 AC 载波信号来传送。这些电流的频率通常高于电力传输电流的频率。

什么是 LC 振荡？

The LC Oscillator employs a tank circuit (comprising an inductor and a capacitor) to provide the necessary positive feedback to keep oscillations in a circuit going. 

充电电容器 (C) 连接到该电路中未充电的电感器，如名称所示 (L)。

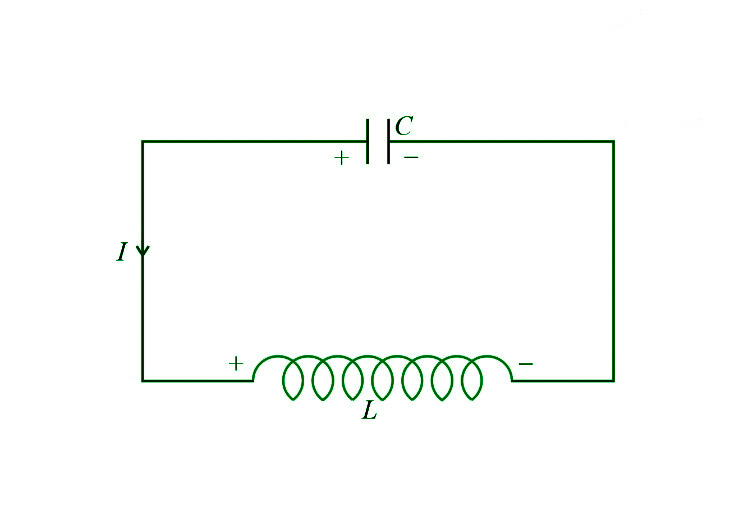
LC振荡器

该电路将使用一个完全充电的电容器和一个完全断电的电感器。该电感器的电阻必须尽可能低（理想情况下为零）。

考虑一下：如果将充电的电容器连接到电阻器，则电阻将消耗电容器的能量，电流最终将停止。

然而，在这种情况下，电容器（存储电能）与低电阻电感器（存储磁能）相连。结果，当电感器开始从电容器吸收能量时，它会被充电，并且其能量会上升，从而导致电容器放电。当电感器完全充电时，电容器失去所有能量，电感器开始使用其存储的能量为电容器充电。能量从电容器传递到电感器，然后返回到电容器。 LC 振荡是从一个设备到另一个设备的持续能量流动。

Equations of Energy Stored in a Capacitor and an Inductor

• Energy Stored in a Capacitor

The capacitor is an electric charge and energy storage device. The voltage difference (V) applied between the ends of a capacitor is exactly proportional to the quantity of charge stored (q). As a result, the charge stored in a capacitor has the following equation:

q = CV

The capacitance of the capacitor is denoted by C.

The change in electric potential energy is now calculated as follows:

dU = q (dV)

dU = CV dV

Integrating the equation as,

∫^U₀ dU = ∫^V₀ CVdV 

U = 1/2 CV² = 1/2 qV = q² / 2C

These are the formulas for calculating the amount of energy stored in a capacitor.

• Energy Stored in an Inductor

The inductor is a device that stores energy in a magnetic field when a current is passed through it. The change in electric current with respect to time is exactly proportional to the potential difference (V) across the ends of an inductor (di/dt).

V = –L di/dt

The inductor’s inductance is denoted by the letter L. The potential energy stored in the inductor will now shift as follows:

dU = V i(dt)

dU = L i(di)

Integrating the equation as, 

∫^U₀ dU = ∫ⁱ₀ L idi

U = 1/2 L i²

This is the formula for calculating the energy stored in an inductor.

LC振荡器的工作

当完全供电的电容器连接到断电的电感器时，电容器接收电路的所有能量，而电感器不接收任何能量。我们将存储在电容器中的能量（电能）称为 U _E和存储在电感器中的磁能（U _B ）。

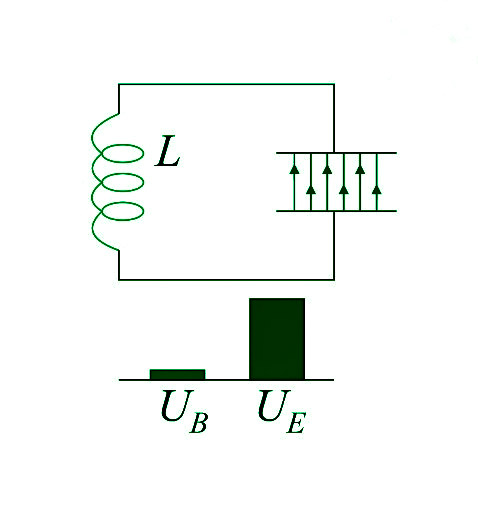
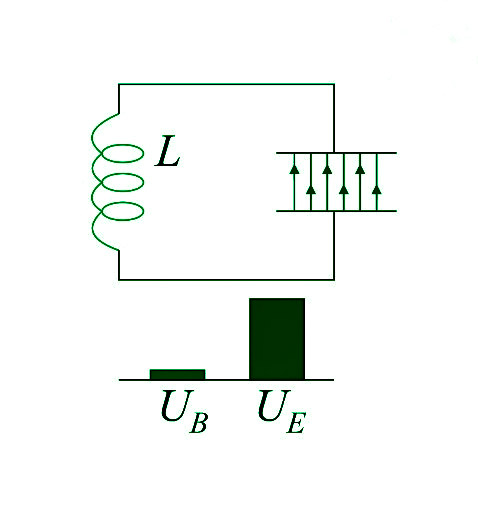
• 电流从电容器流向电感器，使电感器通电并使电容器放电。电感器的能量开始上升，而电容器的能量开始下降。该图描绘了电路的当前状态。电路图下方的条形图显示，此时电感中存储的一半能量相当于电容器中存储的一半能量，表明电容器已将一半的能量转移到电感中。

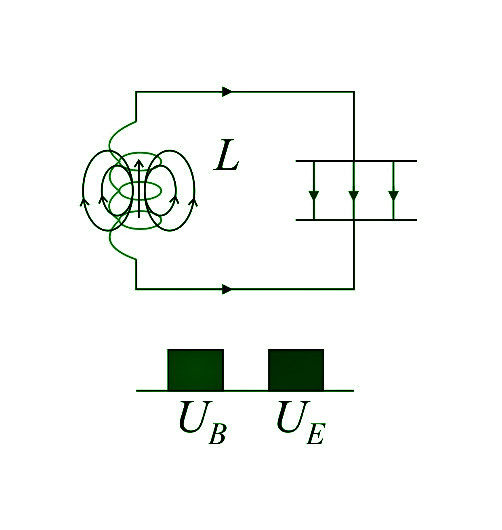
• 一旦电容器完全耗尽，所有电容器的能量现在都将传输到电感器。结果，大量电能转化为磁能。

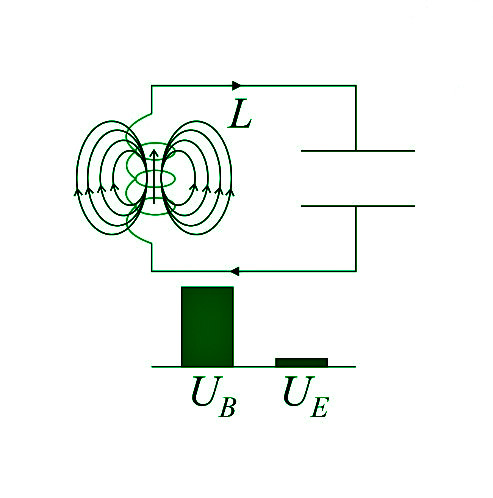
• 因为电容器已经完全耗尽并且电感器已经完全通电，所以电感器现在将开始以与电流相同的方向对电容器充电。电路的当前状态在第四张图中描述。结果，电感器的一半能量已转移到电容器。

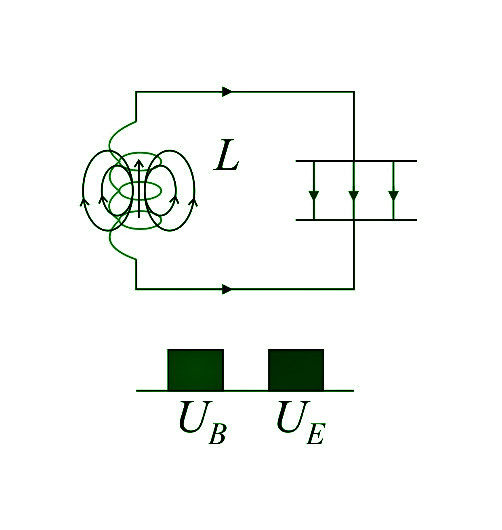
• 最后，电容器将再次充满电，电感器将被完全激活。另一方面，电容器的极性现在将反转。结果，如果电路中的电流再次从电容器中流出，它将以相反的方向流动。我们可以说交流周期的前半部分已经结束，而后半部分已经开始，因为电路中的电流现在具有相反的电流流动。

结果，电容器和电感器都将在循环期间完全充电两次。

Differential Equation of LC Oscillations

We may claim that the total potential differences across capacitor and inductor in the tank circuit will be zero if Kirchhoff’s Voltage Law is applied.

V_L + V_C = 0

–L di / dt + q / C=0

But, i = −dq / dt, since the charge on the capacitor diminishes as time passes:

L d²q / dt² + q/C = 0

⇒ d²q / dt² = –q /LC

The differential equation for LC Oscillations is this equation. The angular frequency of LC oscillations may be written as follows using this equation:

ω = 1 / √LC

Therefore, its frequency will be:

f = 1 / 2π√LC

The LC Oscillation differential equation will have the following solution:

q = q_m sin (ωt+ϕ)

The maximum charge on the capacitor is denoted by q_m. We derive the equation of current by differentiating this equation with regard to time.

i = dq/dt

i = d/dt [(q_m sin(ωt+ϕ))]

⇒ i= q_mωcos(ωt+ϕ)

However, the current flowing through the circuit will be zero at time t=0. Hence,

cos(ϕ) = 0

⇒ ϕ = π/2

Thus, the equation of charge will be;

q = q_m sin(ωt+π/2)

⇒ q=q_m cos(ωt)

Total Energy of LC Oscillations

In LC Oscillations, the charge equation is as follows:

q = q_m cos(ωt)

We derive the present equation by differentiating this equation:

i = dq/dt

⇒ i = –q_m ωsin(ωt)

The formula for calculating the energy stored in a capacitor is:

U_E = q²/2C

Substituting the equation for a given time interval t;

U_E = q_m²/2C × (ωt)

The formula for calculating the energy stored in an inductor is:

U_B = 1/2 L i²

Substituting the capacitor’s equation for the same amount of time;

U_B = 1/2 L q_m² ω² (ωt)

Since the angular frequency, ω=1/ √LC

⇒ U_B = q_m²/2C × (ωt)

As a result, the LC Oscillations’ total energy will be;

U = U_E + U_C

U = q_m²/2C × (ωt)+q_m²/2C × (ωt)

⇒ U = q_m² / 2C

LC 振荡的应用

许多电气设备，例如发射机、无线电设备、滤波器、混频器、电视、射频发生器等，都采用 LC 振荡。

1. 为了将直流信号转换为交流信号，使用了此设备。
2. 该谐振电路可用于升压。
3. 它用于通过调整电感和电容水平来创建具有指定频率的信号。
4. 它们适用于感应加热。

示例问题

问题 1：LC 振荡会发生什么？

回答：

LC Oscillations are caused by the continuous flow of energy from the capacitor (C) to the inductor (L). The capacitor is completely charged at first, then discharged, then charged again, and so on.

问题2：LC电路为什么会振荡？

回答：

When a charged capacitor is connected to a de-energized inductor, the capacitor will begin charging the inductor while discharging itself, and the inductor will do the same when fully energised and the capacitor is fully discharged. As a result, the short current oscillation occurs here.

问题 3：LC 振荡器有什么用？

回答：

The majority of the time, LC oscillators are employed to create a certain frequency.

问题4：LC振荡的频率是多少？

回答：

The frequency of LC oscillation is calculated as follows: 

f = 1/2π √LC

As a result, it is dependent on the capacitance (C) and inductance (L) values.

问题 5：为什么 LC 振荡不现实？

回答：

Because the inductor and capacitor are obviously non-ideal, they will have some resistance in them in real life. As a result, some energy is wasted in the resistance with each cycle of LC oscillation, and it can’t carry on indefinitely.

问题 6：一个 27 mH 电感器连接到一个已充电的 30μF 电容器。电路自由振荡的角频率是多少？

解决方案：

Free oscillations’ angular frequency.

The resonance frequency is calculated as follows:

ω_r = 1/ √LC

     ​= 1/ √27 × 30 × 10^-9

     =1.11 × 10³ rad/s.