振荡和周期性运动

日常生活中会遇到许多类型的运动——直线运动，弹丸的运动就是这样的一个例子。这两个动作都是非重复的。这意味着该对象不会再次回到同一个地方。有些动作本质上是重复的。例如，行星运动。所有的行星每年都围绕太阳运行并完成公转。这种类型的运动是周期性运动。周期性运动的研究是物理学的重要组成部分，了解如何分析此类运动至关重要。让我们详细看看这些动作。

周期性和振荡运动

考虑昆虫试图爬墙的运动。昆虫试图爬上一堵垂直的墙，它达到了一定的高度，然后又掉了下来。昆虫后退，但再次尝试爬墙。昆虫高度随时间变化的图表如下所示。在这样的图表中，请注意昆虫一次又一次地回到零。这种图形也可以在弹跳球中观察到。球也一次又一次地回到它在地面上的位置。

在上图中，运动会在一段时间后重复。这种运动称为周期运动。现在，由于物体正在改变它的速度，这意味着必须有一个力作用在物体上，使它回到某个位置。该特定位置称为平均位置。当身体处于这个位置时，没有任何力作用在它上面。所以，这个位置称为平衡位置。

试图使身体达到平衡位置的力会导致运动中的重复行为。这种重复行为也称为振荡或振动。

Note: There is not really much difference between the oscillations and vibrations. When the frequency is small, they are called oscillations. Else, they are called vibrations. 

周期性运动

考虑时钟、时针、秒针和分针的运动。这些指针覆盖整个时钟并回到相同的位置。在周期性运动中，物体从其平衡位置开始并以相等的时间间隔返回。圆周运动是周期性运动的一个例子。

周期性运动不同于振荡运动。例如，圆周运动是周期性运动，但不是振荡运动。下面给出了一些周期性运动的例子——

1. 一把摇椅
2. 一个弹跳球
3. 圆周运动

振荡运动

摆动运动是物体围绕平衡位置来回运动。摆锤是振荡运动的一个例子。在实践中，由于摩擦或气压等外力引起的阻尼，产生振荡运动的物体最终会停止。但是这种运动可以通过使用保持相等时间间隔的外力来保持。下面给出了一些振荡运动的例子：

1. 附在弹簧上的质量执行振荡。
2. 执行振动的音叉。
3. 一个简单的钟摆。

简谐运动是最简单的一种振荡运动。

Simple Harmonic Motion (SHM) is a kind of oscillatory motion where the net force on the system is restoring force which brings it back to an equilibrium position. If the restoring force is given by F, and the displacement from the mean position is x 

$F \propto x$

周期和频率

由于振荡和周期性运动在相等的时间间隔后重复。有一些可测量的量使我们能够以数学方式描述任何此类运动。这些量还允许我们描述任何物体在周期性运动中的状态。

The smallest interval after a body repeats its motion is called its Time Period. It is denoted by T. Its SI unit is seconds. The reciprocal of the time period (T) is called frequency. It is denoted by f or v and its SI unit is Hz. It denotes the number of repetitions per second. 

v = 1 / T

位移可以定义为振动体距平衡位置的距离。

一个简单的周期函数可以写成，

f(t) = Asin(ωt)

参数 ωt 由下式给出，

T = 2π / ω

因此，函数f(t) 与 T 是周期性的，

f(t + T) = f(t)

示例问题

问题 1：如果身体的时间段是 0.1 秒，则找出身体在 HM 中的频率。

解决方案：

The frequency of a body is given by, 

v = 1 / T

where v = frequency and T = time period. 

Given: T = 0.1 s

Plugging the value into the equation, 

v = 1 / 0.1 s

   = 10 Hz. 

问题 2：如果物体的频率为 250Hz，则在 SHM 中找出物体的时间段。

解决方案：

The frequency of a body is given by, 

v = 1 / T

where, v = frequency and T = time period. 

Given: v = 250 Hz

Plugging the value into the equation, 

v = 1 / T

250 Hz = 1 / T

or

T = 0.004 s

问题 3：一个人的心跳测量结果是每分钟 80 次。找出时间段和频率。

解决方案：

The frequency of a body is given by the number of times heart beats per second. 

v = 80 / 60

v = 1.33 beats per second

The frequency is given by,

v = 1 / T

where v = frequency and T = time period. 

Given: v = 1.33 Hz

Plugging the value into the equation, 

$v = \frac{1}{T} \\ =\frac{4}{3} = \frac{1}{T} \\ =T = \frac{3}{4}\\ = T = 0.75 \text{ seconds}$

问题 4：执行 SHM 的物体由以下等式控制：A = 5sin(44t)。找出时间段。

解决方案：

A standard equation of an SHM is given by, 

$f(t) = A sin(\omega t)$

where T = 2π / ω

In this case, A = 5sin(44t)

Comparing the two equations, we find that 

ω = 44

Plugging the value into the equation for a time period. 

$T = \frac{2\pi}{\omega} \\ = T = \frac{2\pi}{44} \\ = T = \frac{2 \times 22}{7 \times 44}\\ = T = \frac{1}{7}$

问题 5：执行 SHM 的主体由以下等式控制：A = 3sin(t)。找出时间段。

解决方案：

A standard equation of an SHM is given by, 

$f(t) = A sin(\omega t)$

Here, $T = \frac{2\pi}{\omega}$

In this case, A = 3sin(t)

Comparing the two equations, we find that 

$\omega = 1$

Plugging the value into the equation for a time period. 

$T = \frac{2\pi}{\omega} \\ = T = \frac{2\pi}{1} \\ = T = 2\pi$

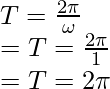
问题 6：执行 SHM 的物体由以下等式控制：A = sin(t) + cos(t)。找出时间段。

解决方案：

A standard equation of an SHM is given by, 

$f(t) = A sin(\omega t)$

Here, $T = \frac{2\pi}{\omega}$

In this case, A = sin(t) + cos(t)

This equation can be rewritten as, 

$A = \sqrt{2}sin(t + \frac{\pi}{4})$

Comparing the two equations, we find that 

$\omega = 1$

Plugging the value into the equation for a time period. 

$T = \frac{2\pi}{\omega} \\ = T = \frac{2\pi}{1} \\ = T = 2\pi$