重要数字

为了找到不同尺寸的值并进行比较，使用了测量。量东西不仅是一个概念，而且在日常生活中也有实际应用，例如，送牛奶的人在卖牛奶之前会量牛奶，以确保送达正确的数量，裁缝在制作西装之前总是先量身体的大小，A鞋匠在制作/给鞋底之前测量脚的大小，我们周围还有很多其他的例子来证明测量的重要性。

测量

测量是将要测量的数量与可用的标准数量进行比较。测量是一个主题，不仅在数学中教授，而且在物理和化学中也教授，因为每个学科都需要了解测量，以便测量数量。

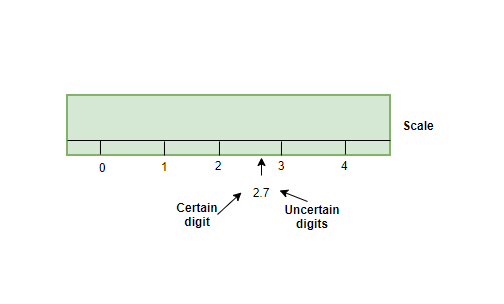
测量数量并不总是能给出完全准确的答案。只有理想的测量仪器才能提供完全准确的答案。实际上，测量会导致答案的两个部分，称为可靠数字和不确定数字。

重要数字

有效数字或有效数字被定义为包含所有特定数字和第一个不确定数字的数字。例如，一个物体的长度被测量为123.5m，这里1、2和3是确定的数字，5是不确定的数字，因此，在这种情况下有效数字是4。

如果一个数量的值超过分配给该值的有效数字，则该数量的值肯定是有缺陷和误导性的。这就是教导错误的原因。有效数字被称为测量的分辨率。

确定重要数字的规则：

• 所有非零数字都被视为有效数字。

 For example, 67812 has 5 significant figures.

• 位于两个非零数字之间的所有零也被认为是重要的，而与值中的小数位无关。

 For example, 1.03 have 3 significant figures.

• 如果零出现在非十进制值的最右侧，则零将不被视为有效数字。

 For example, 25000 has 2 significant figures. 

• 如果数字小于 1，这里需要注意两件事。首先，出现在值末尾的零不被视为有效数字。其次，非零数字左侧的零被认为是重要的。

 For example, 0.067 (here 2 zeroes are present on the left side, are considered significant in nature)

• 如果该值本质上是小数，则尾随零被视为有效数字。

 For example, 2.30 has 3 significant figures.

对有效数字应用算术运算时的规则：

两个值在进行算术运算时，得到的最终值总是会得到更多的有效数字。比如说，为了确定电路中的电流，电阻和电压分别为 6.77 欧姆和 12.559 伏。得到的电流是1.8550960118168，电流值比电压值和电阻值有很多有效数字，但是众所周知，当对两个包含误差的量进行一些运算时，最终的结果肯定有比给定数量更多的错误。因此，在进行任何算术运算时，都会制定一些规则来理解有效数字，

• 在进行除法或乘法运算时，得到的最终值的有效数字必须与输入值中的数字相同（有效数字较低的输入值）。

For example, Take the previous example where R= 6.77ohm and V= 12.559volts, the current is described as,

I = V/R

I = 12.559/6.77

I = 1.850960118168amperes

According to the rule, I should have 3 significant digits, that is, I= 1.85 amperes.

• 在加法或减法时，获得的最终结果应具有与输入值中包含的最低有效数字一样多的有效数字。

For example, Take Inputs as 4.556 and 7.9864. If addition is done on these two inputs, the result is 12.5424. However, the result should be considered as 12.54.

确定有效数字时要牢记的要点：

• 值单位的变化不会改变有效数字。

For example: 23m = 2300cm = 230000 mm, They all have 2 significant digits.

• 在表示测量值时最好使用科学计数法。符号必须是 a × 10 ^b 。

For example, Take the above example, 23m = 2.3 × 10¹ m= 2.3 ×10³ cm= 2.3 × 10⁵ mm. In all the case, significant digit will come out to be 2.

• 如果确切的数字被除或相乘，它将给出无限的有效数字。

示例问题

问题1：确定以下数量的有效数字，

1. 232
2. 1.500
3. 0.0899
4. 5.6 × 10 ³
5. 85633.98

回答：

Based on the rules provided for determining significant digits, 

1. 232= 3 significant figures
2. 1.500= 2 significant figures
3. 0.0899= 5 significant figures
4. 5.6 × 103 = 2 Significant figures
5. 85633.98 = 7 Significant figures.

问题 2： 33. 689 和 44. 23 两个数字相加。在得到的结果值中找到有效数字。

回答：

Adding 33.689 and 44.23, this will give 77.919. But the input value with the least significant digit is 44. 23. Therefore, the answer is 77.91.

问题3：一个物体的质量是50.9kgs，体积是2.34m ³ 。根据有效数字规则找到对象的密度。

解决方案：

Density of an object is defined as,

$Density= \frac{mass}{volume}\\=\frac{50.9}{2.34}\\=21.752136752136kg/m^3$

Since, the least significant value in the input is 3.

Hence, the density of the object is 21.7kg/m³.

问题4：两个值相乘得到某个值作为结果。输入值为 3.99 和 1.5789，求得到的结果值的有效数字。

回答：

The input values do not even needed to be added in order to find the significant figure of the resultant value as there is an easier way. Just by looking as the rules, it can be seen that the first rule of arithmetic operations of significant figures says that the resultant value has the same significant digits as found in the input with the least significant digit.

Therefore, The result will have 3 significant digits.

问题5：0.06700和6.700有什么区别？

回答：

The difference is due to the fact that 0.06700 is a number less than 1 and hence, the zeros on the left are significant, but the zeroes on the rightmost side are insignificant (rule 4). The significant digits are 4.

While, the number 6.700 is a number greater than 1 and also a decimal, therefore, the zeroes on the rightmost side are significant (rule 5). The significant digits are 4.