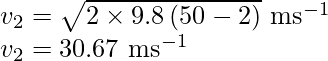伯努利原理

我们周围到处都可以找到液体。地球被空气包围，水覆盖了其表面的三分之二。水不仅对我们的生存至关重要；它对于每个动物物种的生存也是必不可少的。每个哺乳动物的身体主要由水构成。流体不同于固体，它没有自己独特的形状。气体覆盖其容器的全部容量，而固体和液体具有固定的体积。由于外部压力的变化，体积可以改变，在固体或液体中相当小，即固体和液体的可压缩性远低于气体。

剪切应力可以改变固体的几何形状，同时保持其体积。流体有一个关键特性：它们对剪切应力的抵抗力极低；当施加适量的剪切应力时，它们的形状会发生变化。流体的剪切应力比固体低一百万倍。

伯努利原理

由丹尼尔·伯努利介绍的伯努利原理指出，随着流动流体（液体或气体）的速度增加，流体内的压力也会增加。尽管伯努利建立了这个规则，但莱昂哈德·欧拉在 1752 年以标准形式发展了伯努利方程。

伯努利原理指出：

The net mechanical energy of the flowing fluid, which includes gravitational potential energy of elevation, fluid pressure energy, and kinetic energy of fluid motion, stays constant.

流体流动是一个棘手的概念，但是，能量守恒用于获得一些有用的特征，以实现稳定或流线型流动。伯努利原理的表达起源于能量守恒定律。当将该理论应用于理想状态的流体时，密度和压力都成反比。结果，以较慢速率运动的流体将比以较快速率运动的流体施加更大的力。

Bernoulli’s Principle Formula

Bernoulli’s equation formula is a relationship between a fluid’s pressure, kinetic energy, and gravitational potential energy in a container.

Bernoulli’s principle is defined as follows:

$P+\frac{1}{2}\rho v^2+\rho gh=\text{Constant}$

where P is the pressure exerted, v is the velocity, ρ is the density of the fluid and h is the height of the container.

伯努利方程的推导

考虑流经具有变化横截面积的管道的流体和以恒定速率运行的不可压缩的流体。如图所示，管道应处于不同的高度。根据连续性方程，它的速度一定是波动的。为了达到这个加速力是需要的，它是由它周围的流体产生的，而且不同地方的压力必须不同。

伯努利方程是一个通用公式，它将速度变化（即动能变化）和高度变化（即势能变化）与管道中两个位置之间的压力差联系起来。

理想流体在不同横截面的管道中的流动。

考虑两个区域 BC 和 DE 的流动。看看两个不同地区的流量：BC 和 DE。考虑存在于 B 和 D 之间的流体将以无限小的时间间隔 Δt 传播。如果 v ₁是 B 处的速度并且 v ₂是 D 处的速度，则 B 处的流体已经行进了 v ₁ Δt 到 C 的距离，即

dx ₁ = v ₁ Δt

在相同的间隔 Δt 中，最初在 D 处的流体移动到 E，距离等于 v ₂ Δt，即

dx ₂ = v ₂ Δt

区域A ₁和A ₂具有压力P ₁和P ₂ 。在 BC 对流体所做的工作是，

W ₁ = P ₁ ​A ₁ ​⋅ v ₁ Δt

= P ₁ ΔV

其中 ΔV 是通过区域 BC 的体积。

由于相同的体积ΔV通过区域 BC 和 DE。因此，流体在另一端 DE 所做的功的表达式为，

W ₂ = P ₂ ​A ₂ ​⋅ v ₂ Δt

= P ₂ ΔV

所以对流体所做的总功是，

W ₁ – W ₂ = P ₁ ​ΔV – P ₂ ​ΔV

= ΔV (P ₁ – P ₂ )

设流体的密度为 ρ，因此表达式：

Δm = ρΔV 是在时间 Δt 中通过管道的质量。那么引力势能的变化是，

$\Delta{U} =\rho g\Delta{V}\left(h_{2}-h_{1}\right)$

其动能的变化由下式给出：

$\Delta{K}=\frac{1}{2}\rho\Delta{V}\left(v^2_{2}-v^2_{1}\right)$

根据功——能量定理'

$\left(P_{1}-P_{2}\right)\Delta{V}=\frac{1}{2}\rho \Delta{V}\left(v^2_{2}-v^2_{1}\right)+\rho g\Delta{V}\left(h_{2}-h_{1}\right)\\ \left(P_{1}-P_{2}\right)=\frac{1}{2}\rho\left(v^2_{2}-v^2_{1}\right)+\rho g\left(h_{2}-h_{1}\right)$

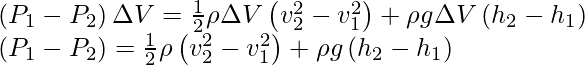
重新排列上面的表达式，

$P_{1}+\frac{1}{2}\rho v^2_{1}+\rho gh_{1}=P_{2}+\frac{1}{2}\rho v^2_{2}+\rho gh_{2}$

这是伯努利方程，可以写成一般表达式，

$P+\frac{1}{2}\rho v^2+\rho gh=\text{Constant}$

伯努利原理的应用

• 文丘里仪表：文丘里仪表是一种通过管道测量液体流速的设备，它基于伯努利定理。
• 飞机：伯努利定理支配着飞机的运行。飞机的机翼有一定的形状。当飞机移动时，尽管飞机的假发表面很低，但空气仍以高速流过它。由于伯努利原理，机翼上方和下方的空气流动存在变化。由于机翼上表面的空气流动，这个概念会导致压力发生变化。如果力大于飞机的质量，飞机就会上升。
• 马格努斯效应：一个旋转的球一旦被抛出，就会在飞行中偏离其正常路线。马格努斯效应是这种现象的名称。这种影响在板球、足球和网球等运动中很重要。

伯努利原理的局限性

以下是伯努利原理的局限性：

• 由于摩擦，管中部的流体粒子速度朝着管的方向逐渐减小。液体粒子的速度不是恒定的，因此必须使用液体的平均速度。
• 这个伯努利方程可用于简化液体供应。它在湍流或非稳态流动中是无效的。
• 液体流动会受到液体外力的影响。
• 该定理优选适用于非粘性流体。
• 需要不可压缩的流体。
• 当流体沿弯曲路径行进时，必须考虑离心力产生的能量。
• 液体流量应随时间保持恒定。
• 在不稳定的流动中，少量的动能可以转化为热能，而在稠密的流动中，由于剪切应力会损失一些能量。因此，必须忽略这些损失。
• 粘性的影响必须可以忽略不计。

示例问题

问题 1：水以 2m3s 的速度流过直径为 1 m 的管子。如果此时的压力是80 kPa，管子缩窄到0.5m直径后水的压力是多少？ ρ_水=1.0 kgl ^-1

解决方案：

According to Bernoulli’s expression:

$P_{1}+\frac{1}{2}\rho v^2_{1}+\rho gh_{1}=P_{2}+\frac{1}{2}\rho v^2_{2}+\rho gh_{2}$

The height is same, so the expression can be written as

$P_{2}=P_{1}+\frac{1}{2}\rho \left(v^2_{1}-v^2_{2}\right)$

The expression for the cross-sectional area is

$A_{1}=\pi\frac{ d^2}{4}\\ A_{1}=\pi\frac{ 1^2}{4}\text{ m}^2\\ A_{1}=\frac{\pi}{4}\text{ m}^2\\$

Similarly

 $A_{2}=\pi\frac{ d^2}{4}\\ A_{2}=\pi\frac{ 0.5^2}{4}\text{ m}^2\\ A_{2}=\frac{\pi}{16}\text{ m}^2\\$

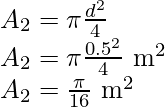
The expression for the velocity for each diameter is 

$v_1=\frac{V}{A}\\ v_1=\frac{2}{\frac{\pi}{4}}\frac{\text{m}}{s}\\ v_1=\frac{8}{\pi}\frac{\text{m}}{s}$

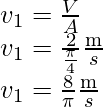
Similarly,

$v_2=\frac{2}{\frac{\pi}{16}}\frac{\text{m}}{s}\\ v_2=\frac{32}{\pi}\frac{\text{m}}{s}$

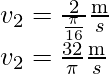
Substitute the value in Bernoulli’s expression:

$P_{2}=80000\text{ Pa}+\frac{1}{2}\times1000\frac{\text{kg}}{\text{m}^3}\times\left(\frac{8}{\pi}-\frac{32}{\pi}\right)\frac{\text{m}}{s}\\P_{2}=76.2\text{ kPa}$

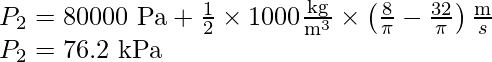
问题2：解释为什么？

(a) 人类脚部的血压高于脑部的血压。

(b) 尽管大气高度超过 100 公里，但在约 6 公里高度处的大气压力降至海平面值的近一半。

(c) 静水压力是一个标量，尽管压力是力除以面积。

解决方案：

(a) The height of the blood column in the foot is greater than that in the brain. As a result, human blood pressure is higher in the feet than in the brain.

(b) The relationship between air density and height is not linear. As a result, pressure does not decrease linearly with height. P = P₀e^–αh gives the air pressure at a height h, where P₀ is the pressure of air at sea level and α is a constant.

(c) When a force is applied to a liquid, the pressure is distributed evenly throughout the liquid. As a result, the pressure due to liquid has no definite direction. As a result, hydrostatic pressure is a scalar value.

问题 3：驱动伯努利原理的表达式。

解决方案：

The flow of an ideal fluid in a pipe of varying cross-section. The fluid in a section of length v₁∆t moves to the section of length v₂∆t in time ∆t

Consider the flow at two regions BC and DE. Take a look at the flow in two different regions: BC and DE. Consider the fluid that present between B and D would traveled in an infinitesimal time interval ∆t. If v₁ is the speed at B and v₂ is the speed at D, the fluid at B has traveled a distance of v₁∆t to C i.e.

$dx_{1}=v_{1}\Delta{t}$

In the same interval ∆t the fluid initially at D moves to E, a distance equal to v2∆t i.e.

$dx_{2}=v_{2}\Delta{t}$

The areas A₁ and A₂  has  the pressures P₁ and P₂. The work done on the fluid at BC is

$W_{1}=P_{1}A_{1}\cdot v_{1}\Delta{t}\\ W_{1}=P_{1}\Delta{V}$

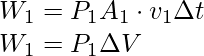
where  ∆V is the volume passes through region BC.

Since the same volume ∆V passes through both the regions BC and DE. therefore the expression for the work done by the fluid at the another end DE is

$W_{2}=P_{2}A_{2}\cdot v_{2}\Delta{t}\\ W_{2}=P_{2}\Delta{V}$

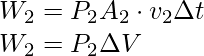
So the total work done on the fluid is$P+\frac{1}{2}\rho v^2+\rho gv^2=contant$

$W_{1}-W_{2}=P_{1}\Delta{V}-P_{2}\Delta{V}\\ W_{1}-W_{2}=\left(P_{1}-P_{2}\right)\Delta{V}$

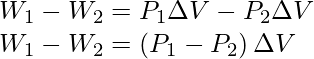
Let the density  of the fluid is ρ therefore  the expression ∆m = ρ∆V is the  mass passing through the pipe in time ∆t, then  change in gravitational potential energy is

$\Delta{U} =\rho g\Delta{V}\left(h_{2}-h_{1}\right)$

The change in its kinetic energy is given by

$\Delta{K}=\frac{1}{2}\rho\Delta{V}\left(v^2_{2}-v^2_{1}\right)$

According to he work – energy theorem’

$\left(P_{1}-P_{2}\right)\Delta{V}=\frac{1}{2}\rho \Delta{V}\left(v^2_{2}-v^2_{1}\right)+\rho g\Delta{V}\left(h_{2}-h_{1}\right)\\ \left(P_{1}-P_{2}\right)=\frac{1}{2}\rho\left(v^2_{2}-v^2_{1}\right)+\rho g\left(h_{2}-h_{1}\right)$

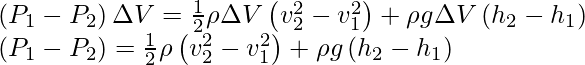
Rearrange the above expression,

$P_{1}+\frac{1}{2}\rho v^2_{1}+\rho gh_{1}=P_{2}+\frac{1}{2}\rho v^2_{2}+\rho gh_{2}$

This is Bernoulli’s equation and this can written as general expression.

This is Bernoulli’s equation and this can written as general expression,

$P+\frac{1}{2}\rho v^2+\rho gh=contant$

问题4：伯努利原理的局限性是什么。

解决方案：

Following are the limitations of Bernoulli’s principle:

• Because of friction, the fluid particle velocity in the middle of a tube  gradually decreases toward the tube’s direction. the velocity of the particles of the liquid are not constant, therefore the liquid’s mean velocity must be used.
• This Bernoulli’s equation can be used to streamline liquid supply. It is ineffective in turbulent or non-steady flow.
• The liquid flow will be affected by the liquid’s external force.
• This theorem preferably applies to non-viscosity fluids.
• Incompressible fluid is required.
• When a fluid is traveling in a curved path, the energy generated by centrifugal forces must be taken into account.
• The liquid flow should remain constant over time.
• A small amount of kinetic energy can be converted to heat energy in an unstable flow, and some energy can be lost due to shear stress in a thick flow. As a result, these losses must be overlooked.
• The effect of viscous must be negligible

问题 5：假设一个 50m 高、装满水的巨大水箱向大气敞开，子弹穿过水箱一侧，使水流出。该孔距地面2m。如果孔与水箱的尺寸相比非常小，水从水箱中流出的速度有多快？

解决方案：

According to Bernoulli’s expression:

$P_{1}+\frac{1}{2}\rho v^2_{1}+\rho gh_{1}=P_{2}+\frac{1}{2}\rho v^2_{2}+\rho gh_{2}$

According to question it is assumed that the top of the container as point 1, and the hole where water is flowing out as point 2. Both points are open to the atmosphere. Therefore, the pressure on each side of the above equation is equal to 1 atm, and thus it got cancel. The size of the hole on the side of the tank is so small compared to the rest of the tank, the velocity of the water at point 1 is nearly equal to 0. Hence, we can cancel out the $\frac{1}{2}ρv^2_1$ term on the left side of the equation. The expression can rewrite as,

$\rho gh_1=\frac{1}{2}\rho v^2_{2}+\rho gh_{2}\\ gh_1=\frac{1}{2}v^2_{2}+gh_{2}\\v^2_{2}=2\left(gh_1-gh_2\right)\\v_{2}=\sqrt{2g\left(h_1-h_2\right)}\\$

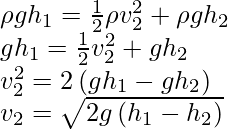
Substitute the values in the above expression,

$v_{2}=\sqrt{2\times9.8\left(50-2\right)}\text{ ms}^{-1}\\v_{2}=30.67\text{ ms}^{-1}$