切线 3 Theta 公式

三角学是研究直角三角形的边的比率与其角度之间的联系。各种三角比，如正弦、余弦、正切、余切、割线和余割线都用于检查该研究领域。三角学由名称“Trigonon”和“Metron”组成，分别代表三角形和测量值。它是研究直角三角形的边和角之间的连接。因此，它通过使用基于此关系的方程和恒等式来帮助计算直角三角形的未知尺寸。

正切三角比

任何两个直角三角形边的比率称为三角比。切线比定义为角的对边的长度除以相邻边的长度的比值。

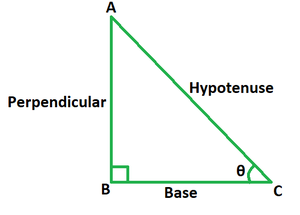
如果 θ 是直角三角形的底边和斜边所形成的角度，那么，

tan θ = Perpendicular/Base = sin θ/ cos θ

Here, perpendicular is the side opposite to the angle and base is the side adjacent to it.

Tangent 3 Theta (Tan 3 θ ) 公式

Tan3θ 是三角学中的三重角恒等式。它是一个关键的三角恒等式，用于解决各种三角和积分问题。它是一个函数，返回三角的 tan函数值。它也可以写成 tan3θ = sin 3θ/cos 3θ，因为正切函数是正弦函数和余弦函数的比率。 tan3θ 的值在每 π/3 弧度后重复一次，tan3θ = tan (3θ + π/3)。它的图比 tan θ 的图更薄。

$\tan3\theta=\frac{3\tan\theta-\tan^3\theta}{1-3\tan^2\theta}$

推导

The formula for Tangent 3 theta is derived by using the sum angle formula for Tangent theta and Tangent 2 theta ratios.

To demonstrate that tan 3θ = (3 tan θ – tan³θ) / (1 – 3 tan²θ), we write 3θ as (2θ + θ).

L.H.S. = tan 3θ 

= tan (2θ + θ)

Use the formula tan (x + y) = (tan x + tan y) / (1 – tan x tan y)

= (tan 2θ + tan θ)/ (1 – tan 2θ tan θ)

Use the formula tan 2x = (2 tan x) / (1 – tan²x) for tan 2θ.

= [(2 tan θ / (1 – tan²θ)) + tan θ] / [1 – (2 tan θ / (1 – tan²θ)) tan θ]

= (tan θ – tan³θ + 2 tan θ) / (1 – tan²θ – 2 tan²θ)

= (3 tan θ – tan³θ) / (1 – 3 tan²θ) 

= R.H.S.

This derives the formula for tangent 3 theta ratio.

示例问题

问题 1. 如果 tan θ = 3/4，使用公式求 tan 3θ 的值。

解决方案：

We have, tan θ = 3/4.

Using the formula we get,

tan 3θ = (3 tan θ – tan³θ) / (1 – 3 tan²θ)

= (3 (3/4) – (3/4)³) / (1 – 3 (3/4)²)

= (9/4 – 27/64) / (1 – 3 (9/16))

= (117/64) / (-11/16)

= -117/44

问题 2. 如果 tan θ = 12/5，使用公式求 tan 3θ 的值。

解决方案：

We have, tan θ = 12/5.

Using the formula we get,

tan 3θ = (3 tan θ – tan³θ) / (1 – 3 tan²θ)

= (3 (12/5) – (12/5)³) / (1 – 3 (12/5)²)

= (36/5 – 1728/125) / (1 – 3 (144/25))

= (-828/125) / (-407/25)

= 828/2035

问题 3. 如果 sin θ = 4/5，使用公式求 tan 3θ 的值。

解决方案：

We have, sin θ = 4/5.

Clearly cos θ = 3/5. Hence we have, tan θ = 4/3.

Using the formula we get,

tan 3θ = (3 tan θ – tan³θ) / (1 – 3 tan²θ)

= (3 (4/3) – (4/3)³) / (1 – 3 (4/3)²)

= (4 – 64/27) / (1 – 3 (16/9))

= (44/27) / (-13/3)

= -44/117

问题 4. 如果 cos θ = 12/13，使用公式找到 tan 3θ 的值。

解决方案：

We have, cos θ = 12/13.

Clearly sin θ = 5/13. Hence we have, tan θ = 5/12.

Using the formula we get,

tan 3θ = (3 tan θ – tan³θ) / (1 – 3 tan²θ)

= (3 (5/12) – (5/12)³) / (1 – 3 (5/12)²)

= (5/4 – 125/1728) / (1 – 3 (25/144))

= (2035/1728) / (19/144)

= 2035/228

问题 5. 如果 sec θ = 17/8，使用公式找到 tan 3θ 的值。

解决方案：

We have, sec θ = 17/8.

Find the value of tan θ using the formula sec² θ = 1 + tan² θ.

tan θ = √((289/64) – 1)

= √(225/64)

= 15/8

Using the formula we get,

tan 3θ = (3 tan θ – tan³θ) / (1 – 3 tan²θ)

= (3 (15/8) – (15/8)³) / (1 – 3 (15/8)²)

= (45/8 – 3375/1728) / (1 – 3 (225/64))

= (72/25) / (64/675)

= 243/8

问题 6. 使用 tan 3x 公式求 tan 135° 的值。

解决方案：

We have to find the value of tan 135°.

Let us take 3x = 135

=> x = 135/3

=> x = 45°

We know, tan 45° = 1.

Using the tan 3x formula, we get

tan 135° = (3 tan 45° – tan³45°) / (1- 3 tan²45°)

= (3(1) – 1³) / (1 – 3 (1²))

= (3 – 1) / (1 – 3)

= 2 / (-2)

= -1

问题 7. 使用 tan 3x 公式求 tan 75° 的值。

解决方案：

We have to find the value of tan 75°.

Let us take 3x = 75

=> x = 75/3

=> x = 25°

We know, tan 25° = 0.47.

Using the tan 3x formula, we get

tan 75° = (3 tan 25° – tan³25°) / (1- 3 tan²25°)

= (3(0.47) – (0.47)³) / (1 – 3 (0.47)²)

= (1.41 – 0.10) / (1 – 3 (0.22))

= (1.31) / (0.34)

= 3.85