查找具有给定顶点和尺寸的所有可能矩形的顶点坐标

给定两个整数L和B代表一个矩形的长和宽，一个坐标(X, Y)代表笛卡尔平面上的一个点，任务是找到所有以(X, Y)为顶点的矩形的坐标给定尺寸。

例子：

Input: X=9, Y=9, L=5, B=3
Ouput:
(9, 9), (14, 9), (9, 12), (14, 12)
(4, 9), (9, 9), (4, 12), (9, 12)
(9, 6), (14, 6), (9, 9), (14, 9)
(4, 6), (9, 6), (4, 9), (9, 9)
(9, 9), (12, 9), (9, 14), (12, 14)
(6, 9), (9, 9), (6, 14), (9, 14)
(9, 4), (12, 4), (9, 9), (12, 9)
(6, 4), (9, 4), (6, 9), (9, 9)
Explanation: There are 8 possible rectangles such that one of their vertex is (9, 9) and the length and breadth is 5 and 3 respectively as mentioned above.

Input: X=2, Y=3, L=4, B=1
Ouput:
(2, 3), (6, 3), (2, 4), (6, 4)
(-2, 3), (2, 3), (-2, 4), (2, 4)
(2, 2), (6, 2), (2, 3), (6, 3)
(-2, 2), (2, 2), (-2, 3), (2, 3)
(2, 3), (3, 3), (2, 7), (3, 7)
(1, 3), (2, 3), (1, 7), (2, 7)
(2, -1), (3, -1), (2, 3), (3, 3)
(1, -1), (2, -1), (1, 3), (2, 3)

方法：可以观察到，对于给定的长度和宽度以及顶点(X, Y) ，可能有八个矩形，如下图所示：

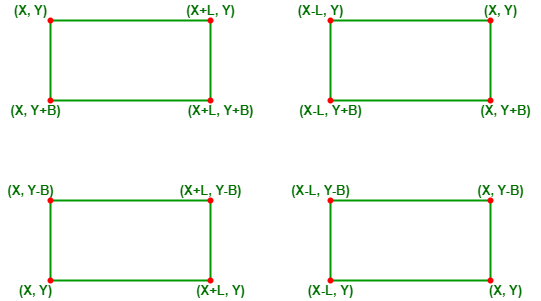
如果矩形的给定长度和宽度相等，则水平和垂直矩形都将表示相同的坐标。因此，图 1 或图 2 中仅可能显示 4 个独特的正方形。

下面是上述方法的实现：

C++

// C++ code for the above approach
#include 
using namespace std;
 
void printHorizontal(int X, int Y, int L, int B)
{
    cout << '(' << X << ", " << Y << "), ";
    cout << '(' << X + L << ", " << Y << "), ";
    cout << '(' << X << ", " << Y + B << "), ";
    cout << '(' << X + L << ", " << Y + B << ")"
         << endl;
}
 
void printVertical(int X, int Y, int L, int B)
{
    cout << '(' << X << ", " << Y << "), ";
    cout << '(' << X + B << ", " << Y << "), ";
    cout << '(' << X << ", " << Y + L << "), ";
    cout << '(' << X + B << ", " << Y + L << ")"
         << endl;
}
 
// Function to find all possible rectangles
void findAllRectangles(int L, int B, int X, int Y)
{
 
    // First four Rectangles
    printHorizontal(X, Y, L, B);
    printHorizontal(X - L, Y, L, B);
    printHorizontal(X, Y - B, L, B);
    printHorizontal(X - L, Y - B, L, B);
 
    // If length and breadth are same
    // i.e, it is a square
    if (L == B)
        return;
 
    // Next four Rectangles
    printVertical(X, Y, L, B);
    printVertical(X - B, Y, L, B);
    printVertical(X, Y - L, L, B);
    printVertical(X - B, Y - L, L, B);
}
 
// Driver Code
int main()
{
    int L = 5, B = 3;
    int X = 9, Y = 9;
 
    findAllRectangles(L, B, X, Y);
}

Java

// Java code for the above approach
class GFG{
 
static void printHorizontal(int X, int Y, int L, int B)
{
    System.out.print("("+ X+ ", " +  Y+ "), ");
    System.out.print("("+ (X + L)+ ", " +  Y+ "), ");
    System.out.print("("+ X+ ", " +  (Y + B)+ "), ");
    System.out.print("("+ (X + L)+ ", " +  (Y + B)+ ")"
         +"\n");
}
 
static void printVertical(int X, int Y, int L, int B)
{
    System.out.print("("+ X+ ", " +  Y+ "), ");
    System.out.print("("+ (X + B)+ ", " +  Y+ "), ");
    System.out.print("("+ X+ ", " +  (Y + L)+ "), ");
    System.out.print("("+ (X + B)+ ", " +  (Y + L)+ ")"
         +"\n");
}
 
// Function to find all possible rectangles
static void findAllRectangles(int L, int B, int X, int Y)
{
 
    // First four Rectangles
    printHorizontal(X, Y, L, B);
    printHorizontal(X - L, Y, L, B);
    printHorizontal(X, Y - B, L, B);
    printHorizontal(X - L, Y - B, L, B);
 
    // If length and breadth are same
    // i.e, it is a square
    if (L == B)
        return;
 
    // Next four Rectangles
    printVertical(X, Y, L, B);
    printVertical(X - B, Y, L, B);
    printVertical(X, Y - L, L, B);
    printVertical(X - B, Y - L, L, B);
}
 
// Driver Code
public static void main(String[] args)
{
    int L = 5, B = 3;
    int X = 9, Y = 9;
 
    findAllRectangles(L, B, X, Y);
}
}
 
// This code is contributed by shikhasingrajput

Python3

# python code for the above approach
def printHorizontal(X, Y, L, B):
 
    print(f"({X}, {Y}), ", end="")
    print(f"({X + L}, {Y}), ", end="")
    print(f"('{X}, {Y + B}), ", end="")
    print(f"({X + L}, {Y + B})")
 
def printVertical(X, Y, L, B):
 
    print(f"({X}, {Y}), ", end="")
    print(f"({X + B}, {Y}), ", end="")
    print(f"({X}, {Y + L}), ", end="")
    print(f"({X + B}, {Y + L})")
 
# Function to find all possible rectangles
def findAllRectangles(L, B, X, Y):
 
    # First four Rectangles
    printHorizontal(X, Y, L, B)
    printHorizontal(X - L, Y, L, B)
    printHorizontal(X, Y - B, L, B)
    printHorizontal(X - L, Y - B, L, B)
 
    # If length and breadth are same
    # i.e, it is a square
    if (L == B):
        return
 
    # Next four Rectangles
    printVertical(X, Y, L, B)
    printVertical(X - B, Y, L, B)
    printVertical(X, Y - L, L, B)
    printVertical(X - B, Y - L, L, B)
 
# Driver Code
if __name__ == "__main__":
 
    L = 5
    B = 3
 
    X = 9
    Y = 9
 
    findAllRectangles(L, B, X, Y)
 
    # This code is contributed by rakeshsahni

C#

// C# code for the above approach
using System;
 
class GFG{
 
static void printHorizontal(int X, int Y, int L, int B)
{
    Console.Write("(" + X + ", " +  Y + "), ");
    Console.Write("(" + (X + L) + ", " +  Y + "), ");
    Console.Write("(" + X + ", " +  (Y + B) + "), ");
    Console.Write("(" + (X + L) + ", " +  (Y + B) + ")" + "\n");
}
 
static void printVertical(int X, int Y, int L, int B)
{
    Console.Write("(" + X + ", " +  Y + "), ");
    Console.Write("(" + (X + B) + ", " +  Y + "), ");
    Console.Write("(" + X + ", " +  (Y + L) + "), ");
    Console.Write("(" + (X + B) + ", " +  (Y + L) + ")" + "\n");
}
 
// Function to find all possible rectangles
static void findAllRectangles(int L, int B, int X, int Y)
{
     
    // First four Rectangles
    printHorizontal(X, Y, L, B);
    printHorizontal(X - L, Y, L, B);
    printHorizontal(X, Y - B, L, B);
    printHorizontal(X - L, Y - B, L, B);
 
    // If length and breadth are same
    // i.e, it is a square
    if (L == B)
        return;
 
    // Next four Rectangles
    printVertical(X, Y, L, B);
    printVertical(X - B, Y, L, B);
    printVertical(X, Y - L, L, B);
    printVertical(X - B, Y - L, L, B);
}
 
// Driver Code
public static void Main(String[] args)
{
    int L = 5, B = 3;
    int X = 9, Y = 9;
 
    findAllRectangles(L, B, X, Y);
}
}
 
// This code is contributed by shikhasingrajput

Javascript

 
// JavaScript code for the above approach
 
function printHorizontal(X, Y, L, B)
{
    document.write('(' + X + ", " + Y + "), ");
    document.write('(' + (X + L) + ", " + Y + "), ");
    document.write('(' + X + ", " + (Y + B) + "), ");
    document.write('(' + (X + L) + ", " +
                         (Y + B) + ")" + '<br>');
}
 
function printVertical(X, Y, L, B)
{
    document.write('(' + X + ", " + Y + "), ");
    document.write('(' + (X + B) + ", " + Y + "), ");
    document.write('(' + X + ", " + (Y + L) + "), ");
    document.write('(' + (X + B) + ", " +
                         (Y + L) + ")" + '<br>');
}
 
// Function to find all possible rectangles
function findAllRectangles(L, B, X, Y)
{
     
    // First four Rectangles
    printHorizontal(X, Y, L, B);
    printHorizontal(X - L, Y, L, B);
    printHorizontal(X, Y - B, L, B);
    printHorizontal(X - L, Y - B, L, B);
 
    // If length and breadth are same
    // i.e, it is a square
    if (L == B)
        return;
 
    // Next four Rectangles
    printVertical(X, Y, L, B);
    printVertical(X - B, Y, L, B);
    printVertical(X, Y - L, L, B);
    printVertical(X - B, Y - L, L, B);
}
 
// Driver Code
let L = 5, B = 3;
let X = 9, Y = 9;
 
findAllRectangles(L, B, X, Y);
 
// This code is contributed by Potta Lokesh
 

输出：

(9, 9), (14, 9), (9, 12), (14, 12)
(4, 9), (9, 9), (4, 12), (9, 12)
(9, 6), (14, 6), (9, 9), (14, 9)
(4, 6), (9, 6), (4, 9), (9, 9)
(9, 9), (12, 9), (9, 14), (12, 14)
(6, 9), (9, 9), (6, 14), (9, 14)
(9, 4), (12, 4), (9, 9), (12, 9)
(6, 4), (9, 4), (6, 9), (9, 9)

时间复杂度： O(1)
辅助空间： O(1)