在数轴上代表√3

数轴是用于表示特定间隔的数字的线，用于简单的数值运算。自然数、整数、整数、有理数和无理数都是数字的类型。这些都是实数或实数行的一部分。所有这些数字都可以用数字线直观地表示吗？用于计数的零之后的正整数称为自然数。请记住，它们用符号“N ”表示。现在向这个集合添加零。您现在拥有由符号“W”表示的整数集合。现在零之前的大量负整数和整数的集合是所有整数的集合，它用符号'Z'表示。你可能想知道，为什么是 Z？ Z来自德语单词“Zahlen”，意思是“数字”。数字行上是否还留有任何数字？是的，当然，有许多不同的数字，例如 1/3、-6352/371、25/6。如果所有这些数字都包含在整数集合中，那么它现在将是有理数集合。回想一下，有理数用'Q'表示。为什么问？ Rational 来自“比率”这个词，Q 来自“商”这个词。有人可能想知道，无理数在这条数轴上的什么位置？不是有理数的数被归类为无理数。毕达哥拉斯学派，著名数学家和希腊哲学家毕达哥拉斯的追随者，是第一个发现无理数的人。

数字类型

有不同类型的数字。上面提到了整数、自然数、整数、有理数、无理数等。我们来看看关于这些具体类型的几点，

自然数

• 这些是用于计数的数字。
• 1、2、3、4……以此类推。
• 它们用符号“N”表示。

整数

• 整数是一组自然数，包括零 (0)。
• 例如。 9、35、100、0 等
• 它们用符号“W”表示。

整数：

• 整数是一组正数和负数，包括零。它们没有小数或小数部分。
• 例如。 24、-18、0、-1、8 等
• 它们用符号“Z”表示。

有理数

• 一组可以用 p/q 形式表示的数，其中 p 和 q 是整数，q 不等于 0，称为有理数。
• 例如。 25/5。 17/8，-3/8。
• 它们用符号“Q”表示。

无理数

• 不能以 p/q 形式表示的一组数，其中 p 和 q 是整数且 q 不等于 0，称为无理数。
• 例如。 Pi (π)、黄金比例 (1.618033….)、√2、√3 等。
• 它们用符号“P”表示。

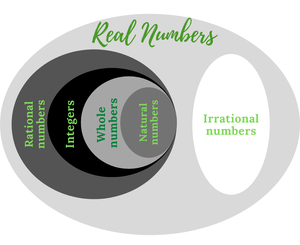
实数饼图

有理数和无理数的区别

首先要记住的是，有理数的小数位要么是终止的，要么是循环的。而无理数的小数位是非终止和非循环的。

Rational numbers	Irrational numbers
1. The decimal places are Terminating or recurring	1. The decimal places are Non-terminating and Non-recurring
2. Can be represented in the form p/q where p is an integer and q is not equal to zero	2. Cannot be represented in the for p/q, where p is an integer and q is not equal to zero.
3. Eg: 1.2, 3.567,-24.92, -18	3. Eg: √8, √11, √50, √3, √5

在数轴上代表√3

方法一

Representation of √2 on the number line 

Step 1: Draw a line segment of 1cm anywhere on a number line.

Step 2: Mark the line segment A and B

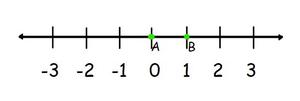
Step 3: Draw a line segment of 1cm perpendicular to AB, and mark the point C.

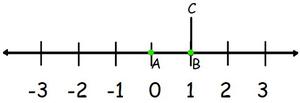
Step 4: Draw a line connecting A and C.

                

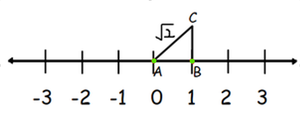
Step 5: AC is √(1² + 1²) = √2

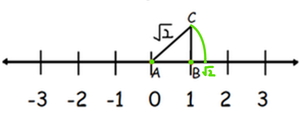
Representation of √3 on the number line

Step 6: Draw a line segment of 1cm perpendicular to AC. Mark the point D

Step 7: Draw line connecting A and D.

Step 8: AD is √ {(√2)² + (1)²}= √3

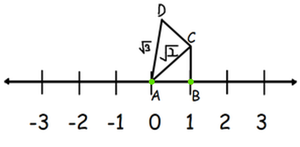
Step 9: Take a compass and take the radius to the length of AD.

Step 10: Extend the arc and mark where it touches the number line as E

Step 11: AE is √3 

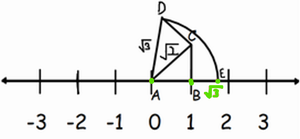
Similarly, locate √n for any positive integer n, after √n-1 has been located. Continue doing this for √4, √5, √6, etc. Square root spiral is obtained. 

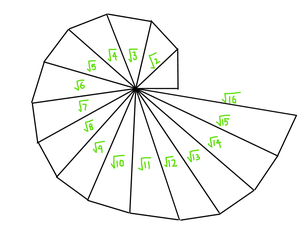
Square root spiral

方法二

Representation of √2 on the number line.

Method 1 is tedious and time-consuming. It is easy to use for smaller numbers. Therefore there is another easy way to represent irrational numbers on the number line which is less time-consuming and can also be used for larger numbers. 

Step 1: Draw a line segment of n cm. In this case, it’s 2 cm, and mark them A and B.

Step 2: Extend B to C to form a line segment of 1cm.

Step 3: Take a compass, and mark an arch, placing one end of the compass in A. With the same radius, draw another arch placing one end of the compass in B. 

Step 4: Mark the point of intersection between the arches as D. 

Step 5: Draw a perpendicular line joining D and AC. Mark this point as E.

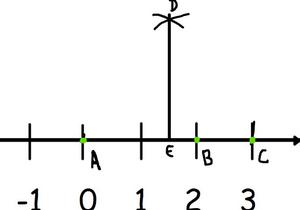
Step 6: Take AE as radius, and draw a semicircle.

Step 7: Draw a perpendicular line joining B and the semicircle, and mark the point F. 

Step 8: BF is √2

Step 9: Take radius AF on the compass on extending it till the number line, and mark the point G.

Step 10: BG is √2 = 1.41421…

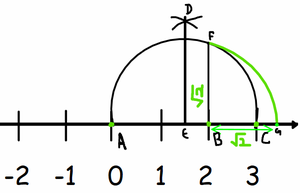
Using the same steps you can represent √n on the number line, by taking AB as n cm.

Representation of √3 on the number line

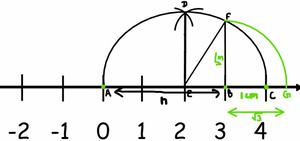
AB = n units, BC = 1 unit.

In this case, n = 3.

BF is = √n

We can prove this by using the Pythagoras Theorem. Note that △EBF is a right-angled triangle and the radius of the circle is (n + 1)/2 units. Therefore, EC = EF = EA = (n + 1)/2 units.

OB = n – (n + 1/2) = (n – 1)/2.

Applying the Pythagoras theorem, 

(BF)² – (EF)² – (EB)² = {(n + 1)/2}² = (4n)/4 = n.

This proves that BF = √n.

示例问题

问题 1：数轴上 √4 的表示。

解决方案：

问题 2：数轴上 √5 的表示。

解决方案：

This construction gives us a visual, and geometric way of showing that √n exists for all real number n>0.