曲率半径公式

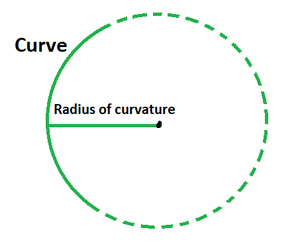
曲率是曲线从平坦到曲线以及从曲线回到直线的标量值。曲率的倒数是曲率半径，它是一个想象的圆，而不是一个真正的形式或图形。曲率半径是特定位置的近似圆的半径。它由曲率矢量长度表示，并用符号 R 表示。它被定义为顶点与曲率中心之间的距离。随着曲线的前进，半径发生变化。

曲率半径公式

曲线的曲率半径定义为圆在任何给定点的近似半径或曲率的矢量长度。它存在于任何具有方程 y = f(x) 且 x 作为其参数的曲线。

Radius of curvature, R = $\frac{\left(1+(\frac{dy}{dx})^2\right)^\frac{3}{2}}{\left|\frac{d^2y}{dx^2}\right|}$

where,

dy/dx = first derivative of the function y = f(x),

d²y/dx² = second derivative of the function y = f(x).

在极坐标 r=r(θ) 的情况下，曲率半径由下式给出

$\rho=\frac{1}{K}\frac{\left[r^2+(\frac{dr}{d\theta})^2\right]^{\frac{3}{2}}}{\left|r^2+2(\frac{dr}{d\theta})^2-r\frac{d^2r}{d\theta^2}\right|}$

where,

K is the tangent vector function and curvature of the curve given by dT/ds,

r is the radius of curvature.

示例问题

问题 1. 求 f(x) = 4x ² + 3x – 7 在 x = 4 处的曲率半径。

解决方案：

We have, y = 4x² + 3x – 7 and x = 4.

dy/dx = 16x + 3

d²y/dx² = 16

Using the radius of curvature formula, we get

R = (1 + (16x + 3)²)^3/2/16

= (1 + 256x² + 9 + 36x)^3/2/16

= (256x² + 36x + 10)^3/2/16

Substitute the value x = 4.

R = (256 (4)² + 36 (4) + 10)^3/2/16

= (4096 + 144 + 10)^3/2/16 

= 27066/16

= 1691.625 units

问题 2. 求 f(x) = 3x ² + 3x – 2 在 x = 1 处的曲率半径。

解决方案：

We have, y = 3x² + 3x – 2 and x = 1.

dy/dx = 6x + 3

d²y/dx² = 6

Using the radius of curvature formula, we get

R = (1 + (6x + 3)²)^3/2/6

= (1 + 36x² + 9 + 36x)^3/2/6

= (36x² + 36x + 10)^3/2/6

Substitute the value x = 1.

R = (36 (1)² + 36 (1) + 10)^3/2/6

= (36 + 36 + 10)^3/2/6

= 742.54/6

= 123.75 units

问题 3. 求 f(x) = 3x ³ – 2x + 7 在 x = 2 处的曲率半径。

解决方案：

We have, y = 3x³ – 2x – 2 and x = 2.

dy/dx = 9x² – 2

d²y/dx² = 18x

Using the radius of curvature formula, we get

R = (1 + (9x² – 2)²)^3/2/18x

= (1 + 81x⁴ + 4 – 36x²)^3/2/18x

= (81x⁴ – 36x² + 5)^3/2/18x

Substitute the value x = 2.

R = (81 (2)⁴ – 36 (2) + 5)^3/2/18 (2)

= (576 – 72 + 5)^3/2/36

= 12305.26/36

= 512.71 units

问题 4. 求 f(x) = 5x ³ – 3x ² + x 在 x = 1 处的曲率半径。

解决方案：

We have, y = 5x³ – 3x² + x and x = 1.

dy/dx = 15x² – 6x + 1

d²y/dx² = 30x – 6

Using the radius of curvature formula, we get

R = (1 + (15x² – 6)²)^3/2/(30x – 6)

= (1 + 225x⁴ + 36 – 180x²)^3/2/(30x – 6)

= (225x⁴ – 180x² + 37)^3/2/(30x – 6)

Substitute the value x = 1.

R = (225(1)⁴ – 180(1)² + 37)^3/2/(30 (1) – 6)

= (225 – 180 + 37)^3/2/24

= 742.54/24

= 30.93 units

问题 5. 求曲线 f(x) = x ²的曲率半径。

解决方案：

We have the curve, y = x²

dy/dx = 2x

d²y/dx² = 2

Using the radius of curvature formula, we get

R = (1 + (2x)²)^3/2/2

= (1 + 4x²)^3/2/2

= (1 + 4y)^3/2/2

问题 6. 求曲线 f(x) = sin x 的曲率半径。

解决方案：

We have the curve, y = sin x.

dy/dx = cos x

d²y/dx² = – sin x

Using the radius of curvature formula, we get

R = – (1 + (cos x)²)^3/2/sin x

= (1 + cos² x)^3/2/sin x

= (1 + cos² x)^3/2/y

问题 7. 求曲线 f(x) = e ^x的曲率半径。

解决方案：

We have the curve, y = e^x.

dy/dx = e^x

d²y/dx² = e^x

Using the radius of curvature formula, we get

R = – (1 + (e^x)²)^3/2/e^x

= (1 + e^2x)^3/2/e^x

= (1 +y²)^3/2/y