两个向量之间的角度公式

向量是那些具有大小和方向的物理量。当两个向量作用于一个粒子时，对粒子的合成作用将取决于这些向量之间的角度。因此，了解它们之间的角度很重要。

Vector用于角度计算的一些性质

向量由与向量方向平行的箭头表示。

• 如果矢量与自身平行传输，则矢量保持不变。
• 具有相同方向的两个向量是平行向量。
• 具有相反方向的两个向量是反平行向量。
• 具有相同大小和方向的两个向量是相等的向量。
• 具有相同大小和相反方向的两个向量称为负向量。

点积

它也被称为向量的标量积。它只有大小，没有方向。

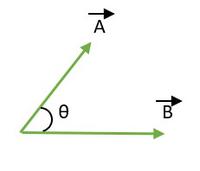
两个向量 A 和 B

然后 A 和 B 的点积由下式给出，

$\vec{A}.\vec{B}$ = |A| |B| cosθ。

特别案例

• 当向量之间的角度为 0 度时。

即 θ = 0°

⇒ |A| |B| cosθ。

⇒ |A| |B| cos0°

⇒ |A| |B| [cos0° = 1]

• 当向量之间的角度为 180 度时。

⇒ |A| |B| cosθ。

⇒ |A| |B| cos180°

⇒ – |A| |B| [cos180° = -1]

• 当向量之间的角度为 90 度时。

⇒ |A| |B| cosθ。

⇒ |A| |B| cos90°

⇒ |A| |B| × 0 [cos90° = 0]

⇒ 0。

两个向量之间的角度公式

The cosine of the angle between two vectors is equal to the sum of the product of the individual constituents of the two vectors, divided by the product of the magnitude of the two vectors.

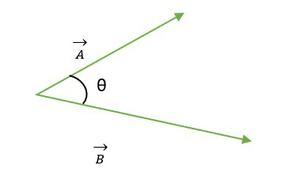
Two Vectors A and B

$\vec{A}.\vec{B}$ =| A | | B | cosθ.

cosθ= $\frac{\vec{A}.\vec{B}}{|A|.|B|}$

θ= cos^-1 $\frac{\vec{A}.\vec{B}}{|A|.|B|}$

In cartesian Form,

A = A_xi + A_yj + A_zk

B= B_xi + B_yj + B_zk 

cosθ = 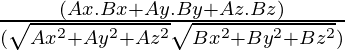$\frac{(Ax.Bx+Ay.By+Az.Bz)}{(\sqrt{Ax^2+Ay^2+Az^2}×\sqrt{Bx^2+By^2+Bz^2})}$

点积的性质

• 点积是可交换的。

$\vec{A}.\vec{B}=\vec{B}.\vec{A}$

• 点积是分布式的。

$\vec{A}.(\vec{B}+\vec{C})=(\vec{A}.\vec{B}+\vec{A}.\vec{C})$

在对流物理学中，两个向量之间的角度介于 0 ≤ θ ≤ 180 之间。当两个向量的尾部或头部重合时，计算向量之间的角度。

尾部重合

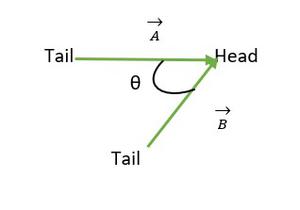
头部重合

示例问题

问题1：求向量之间的角度（如果它们形成等边三角形）

• a 和 b 矢量图
• b 和 c 矢量图
• a 和 c 矢量图

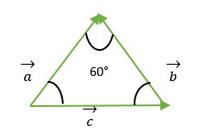
由 a, b, c 向量组成的等边三角形

解决方案：

• a and b vectors

For vector a and b, head of both the vectors coincide with each other, hence angle between a and b vector is same as the angle between two sides of equilateral triangle = 60°.

• b and c vectors:

From the above figure, we see that head or tail of the b and c vector does not coincide with each other.

So, by using the property- A vector remains unchanged if it is transmitted parallel to itself.

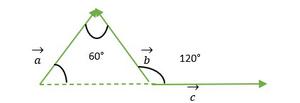
Vector c is shifted parallel to itself

Now we see the tail of vectors b and c are coincide with each other, therefore is the same as the exterior angle make with an equilateral triangle = 120°.

• a and c vectors

The tail of a and c coincide

For vectors a and c, the tail of both the vectors coincide with each other, hence the angle between the a and c vector is the same as the angle between two sides of the equilateral triangle = 60°.

问题2：如果向量形成等腰直角三角形，则求它们之间的角度。

• a 和 b 向量
• b 和 c 向量
• a 和 c 矢量图

解决方案：

• a and b vector

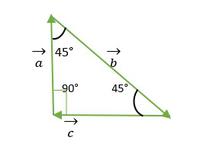
Right angle Isosceles Triangle

From the above figure, we see that head or tail of a and b vector does not coincide with each other. So, by using the property- A vector remains unchanged if it is transmitted parallel to itself.

a vector is shifted parallel to itself

Now, a and b vectors tails coincide with each other and make an angle the same as the exterior angle of a right angle isosceles triangle = 135°.

• b and c vector

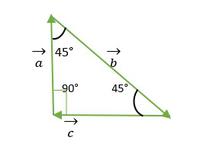
Right angle Isosceles Triangle

From the above figure, b and c vector head or tails does not coincide with each other. So, by using the property, a vector remains unchanged if it is transmitted parallel to itself.

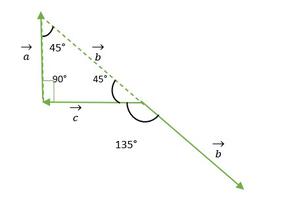
b vector is shifted parallel to itself

Now, b and c vectors tails coincide with each other and make an angle the same as the exterior angle of a right angle isosceles triangle = 135°.

• a and c vectors

Right angle Isosceles Triangle

From the above figure, a and c vector head or tails do not coincide with each other. So, by using the property- A vector remains unchanged if it is transmitted parallel to itself.

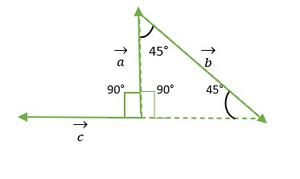
c vector is moved parallel to itself

Now, a and c vectors tails coincide with each other and make an angle the same as the right angle of isosceles triangle = 90°.

问题 3：求向量 A = i + j + k 和向量 B = -2i – 2j – 2k 之间的角度。

解决方案：

From the formula,

A = A_xi + A_yj + A_zk

B= B_xi + B_yj + B_zk       

cosθ= 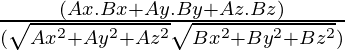$\frac{(Ax.Bx+Ay.By+Az.Bz)}{(\sqrt{Ax^2+Ay^2+Az^2}×\sqrt{Bx^2+By^2+Bz^2})}$

Here in the Given question, 

A= i + j + k.

B= -2i -2j -2k.

Substituting the values in the formula

⇒ cosθ = 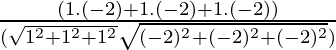$\frac{(1.(-2)+1.(-2)+1.(-2))}{(\sqrt{1^2+1^2+1^2}×\sqrt{(-2)^2+(-2)^2+(-2)^2})}$

⇒ cosθ = $\frac{(-2-2-2)}{(\sqrt{1+1+1}×\sqrt{4+4+4})}$

⇒ cosθ = $\frac{-6}{(\sqrt{3}×\sqrt{12})}$

⇒ cosθ = $\frac{-6}{(\sqrt{36})}$

⇒ cosθ = -6/6

⇒ cosθ= -1

⇒ θ = 180°.

问题 4：求向量 A = 3i + 4j 和 B = 2i + j 之间的角度

解决方案：

A = A_xi + A_yj + A_zk

B = B_xi + B_yj + B_zk      

cosθ = $\frac{(Ax.Bx+Ay.By+Az.Bz)}{(\sqrt{Ax^2+Ay^2+Az^2}×\sqrt{Bx^2+By^2+Bz^2})}$

Here Given,

A= 3i + 4j + 0k

B= 2i + j + 0k

Substituting the values in the formula,

⇒ cosθ = $\frac{(3.2+4.1+0.0)}{(\sqrt{3^2+4^2+0^2}×\sqrt{2^2+1^2+0^2})}$

⇒ cosθ = $\frac{(6+4+0)}{(\sqrt{9+16+0}×\sqrt{4+1+0})}$ 

⇒ cosθ = $\frac{(10)}{(\sqrt{25}×\sqrt{5})}$

⇒ cosθ = $\frac{(10)}{(\sqrt{125})}$

⇒ θ = cos^-1 ($\frac{(10)}{5.(\sqrt{5})}$)

⇒ θ = cos^-1 ($\frac{2}{(\sqrt{5})}$)

问题 5：求向量 A = i + j 和向量 B = j + k 之间的角度。

解决方案：

From the formula,

A = A_xi + A_yj + A_zk

B = B_xi + B_yj + B_zk      

cosθ = 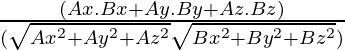$\frac{(Ax.Bx+Ay.By+Az.Bz)}{(\sqrt{Ax^2+Ay^2+Az^2}×\sqrt{Bx^2+By^2+Bz^2})}$  

Here in the Given question, 

⇒ A = i + j 

⇒ B = j + k

⇒ cosθ = $\frac{(1.0+1.1+0.1)}{(\sqrt{1^2+1^2+0^2}×\sqrt{0^2+1^2+1^2})}$

⇒ cosθ = $\frac{(1)}{(\sqrt{1+1+0}×\sqrt{0+1+1})}$

⇒ cosθ =$\frac{1}{(\sqrt{2}×\sqrt{2})}$.

⇒ θ = cos^-1 (1/2)

⇒ θ = 60°.