U-替代整合

求积分基本上是一个反向微分过程。这就是为什么积分也被称为反导数。通常，这些功能是可以轻松集成的简单且标准的功能。使用不定积分的性质更容易求解这些函数的组合。有时函数是由两个函数组成的，这些问题用传统的方法很难解决。在这种情况下，U 替代规则就会发挥作用。直观地说，它只是链式法则的反面。让我们详细看看这个规则。

不定积分

考虑一个在区间内定义和可微的导函数f'(x)。不定积分或反导数使我们能够反转微分过程并计算函数F(x)，其导数已提供给我们。任何给定的导数都有无穷多个反导数。数学积分用符号∫表示

F(x)= ∫ƒ(x) + C

其中 C 是任意常数。下表显示了集成中使用的一些约定和短语及其含义。

Symbol/Term/Meaning	Meaning
∫f(x)dx	Integral of f with respect to x
f(x) in ∫f(x)dx	Integrand
x in ∫f(x)dx	Variable of integration
Integral of f(x)	A function such that F'(x) = f(x)

必须牢记一些标准函数的积分，以便积分变得更简单、更省时。一些标准函数的积分如下所示。

Function	Integral
xn	$\frac{x^{n+1}}{n +1}$
sin(x)	-cos(x)
cos(x)	sin(x)
ex	ex
sec2(x)	tan(x)
$\frac{1}{x}$	ln(x)

积分的性质

Property 1: ∫kf(x)dx= k∫f(x)dx    

Property 2: ∫f(x)± g(x)dx= ∫f(x)dx± ∫g(x)dx     

Property 3: ∫(f1(x)dx± f2(x)dx± f3(x)dx….)= ∫f1(x)dx± ∫f2(x)± ∫f3(x)dx…      

U-替代规则

大多数情况下，可以使用上面提到的属性和公式计算积分。它们允许我们计算更简单的积分，其中被积函数通常是一些简单函数和标准函数的组合。例如，考虑函数f(x) = cos(x) + 5，这个函数的积分很容易，并且可以使用上述属性轻松计算。但现在考虑另一个函数f(x) = sin(3x + 5)。这个函数是两个不同函数的组合，这个函数的积分不像前一个那么容易。此类积分使用 U 代换法求解。

∫f(g(x))g'(x)dx= ∫f(u)du

Here, u= g(x)  

考虑一个例子来理解这个规则。

f(x)= ∫2xcosx ² dx

注意 cos(x ² ) 部分是一个复合函数。 2x 是内部 x ²的导数。因此，假设 h(x) = x ²和 w(x) = cos(x)。因此，我们可以假设 u(x) = x ²和 w(x) = cos(x)，

$\overbrace{2x}^{u'}cos(\overbrace{x^2}^{u}) = u'(x)w(u(x))$

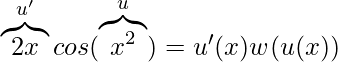
这是替代的表述。考虑 u = x ²

$u' = 2x \\ \frac{du}{dx} = 2x \\ du = 2xdx$

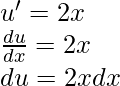
因此，函数变为，

f(x) = ∫cosdu

这可以使用标准公式来解决，

f(x) = ∫cosu du = f(x) = 正弦 + C = f(x) = sinx ² + C

让我们看看这个规则的一些问题，

示例问题

问题 1：求以下函数f(x) 的积分，

f(x)= ∫10x(5x ² )dx,

解决方案：

Given f(x)= ∫10x(5x²)dx, 

Let F(x) be the integral of the function f(x), 

F(x)= ∫f(x)dx= F(x)= ∫10x(5x²)dx

Notice that the function is also a composite function, 

Let u = 5x². Differentiating the expression, 

$\frac{du}{dx} = \frac{d}{dx}(5x^2) \\ = \frac{du}{dx} = 10x \\ = du = 10xdx$

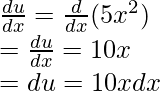
Using these results in the original integral equation, 

F(x)= ∫f(x)dx= F(x)= ∫udu

$\\ = F(x) = \frac{u^2}{2} + C$

Substituting the value of “u” in the above equation, 

F(x) = $\frac{(5x^2)^2}{2} + C$

问题 2：求以下函数f(x) 的积分，

f(x) = 6x(3x ² + 5)

解决方案：

Given f(x) = 6x(3x²+ 5), 

Let F(x) be the integral of the function f(x), 

F(x)= ∫f(x)dx= F(x)= ∫F(x)= ∫6x(3x²+ 5) dx

Notice that the function is also a composite function, 

Let u = 3x² + 5. Differentiating the expression, 

$\frac{du}{dx} = \frac{d}{dx}(3x^2 + 5 ) \\ = \frac{du}{dx} = \frac{d}{dx}(3x^2) + \frac{d}{dx}(5)\\ = \frac{du}{dx} = 6x \\ = du = 6x$

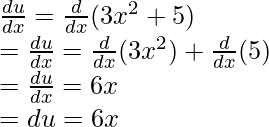
Using these results in the original integral equation, 

F(x)= ∫f(x)dx= F(x)= udu  

$\\ = F(x) = \frac{u^2}{2} + C$

Substituting the value of “u” in the above equation, 

F(x) = $\frac{(3x^2 + 5)^2}{2} + C$

问题 3：求以下函数f(x) 的积分，

f(x)= ∫ (3x ² + 6x)(x ³ + 3×2+ 5)

解决方案：

Given f(x)= ∫ (3x²+ 6x)(x³+ 3×2+ 5), 

Let F(x) be the integral of the function f(x), 

F(x)= ∫f(x)dx= F(x)= ∫ (3x²+ 6x)(x³+ 3×2+ 5) dx

Notice that the function is also a composite function, 

Let u = x³ + 3x² + 5. Differentiating the expression, 

$\frac{du}{dx} = \frac{d}{dx}(x^3 + 3x^2 + 5 ) \\ = \frac{du}{dx} = \frac{d}{dx}(x^3) + \frac{d}{dx}(3x^2) + \frac{d}{dx}(5)\\ = \frac{du}{dx} = 3x^2 + 6x \\ = du = (3x^2+6x)dx$

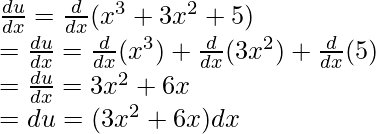
Using these results in the original integral equation, 

F(x)= ∫f(x)dx= F(x)= ∫udu

$\\ = F(x) = \frac{u^2}{2} + C$

Substituting the value of “u” in the above equation, 

F(x) = $\frac{(x^3 + 3x^2 + 5)^2}{2} + C$

问题 4：求以下函数f(x) 的积分，

f(x) = sin(x)cos(x)

解决方案：

Given f(x) = sinxcosx, 

Let F(x) be the integral of the function f(x), 

F(x)= ∫f(x)dx= F(x)= ∫ sinxcosxdx

Notice that the function is also a composite function, 

Let u = cos(x). Differentiating the expression, 

$\frac{du}{dx} = \frac{d}{dx}(cos(x)) \\ = \frac{du}{dx} = -sin(x) \\ = du = -sin(x)dx$

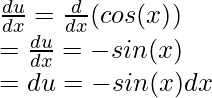
Using these results in the original integral equation, 

F(x)= ∫f(x)dx= F(x)= ∫ sin(x)cos(x)dx

F(x)= ∫-cos(x)(-sin(x))dx= F(x)= ∫-udu

$F(x) = \frac{-u^2}{2} + C$

Substituting the value of “u” in the above equation, 

F(x) = 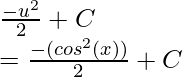$\frac{-u^2}{2} + C \\ = \frac{-(cos^2(x))}{2} + C$

问题 5：求以下函数f(x) 的积分，

f(x)= ∫ 3x ² cosx ³

解决方案：

Given f(x)= ∫ 3x²cosx³, 

Let F(x) be the integral of the function f(x), 

F(x)= ∫f(x)dx= F(x)= ∫ 3x²cosx³dx

Notice that the function is also a composite function, 

Let u = x^3. Differentiating the expression, 

$\frac{du}{dx} = \frac{d}{dx}(x^3) \\ = \frac{du}{dx} = 3x^2 \\ = du = 3x^2dx$

Using these results in the original integral equation, 

F(x)= ∫f(x)dx

F(x)= ∫3x2cos(x)dx

F(x)= ∫cosu du

F(x) = sinu +C

Substituting the value of “u” in the above equation, 

F(x) = sin(u) + C

⇒F(x) = sin(x³) + C

问题 6：求以下函数f(x) 的积分，

f(x) = ∫3x ² cosx ³

解决方案：

Given f(x)= ∫3x²cosx³, 

Let F(x) be the integral of the function f(x), 

F(x)= ∫f(x)dx

F(x)= ∫3x²cosx³dx

Notice that the function is also a composite function, 

Let u = x^3. Differentiating the expression, 

$\frac{du}{dx} = \frac{d}{dx}(x^3) \\ = \frac{du}{dx} = 3x^2 \\ = du = 3x^2dx$

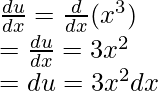
Using these results in the original integral equation, 

F(x)= ∫f(x)dx

F(x)= ∫3x²cos(x)dx

F(x)= ∫cosu du

F(x)= sin(u)+ C

Substituting the value of “u” in the above equation, 

F(x) = sin(u) + C

⇒F(x) = sin(x³) + C