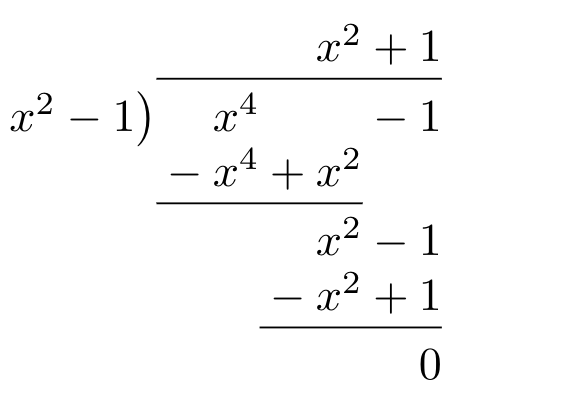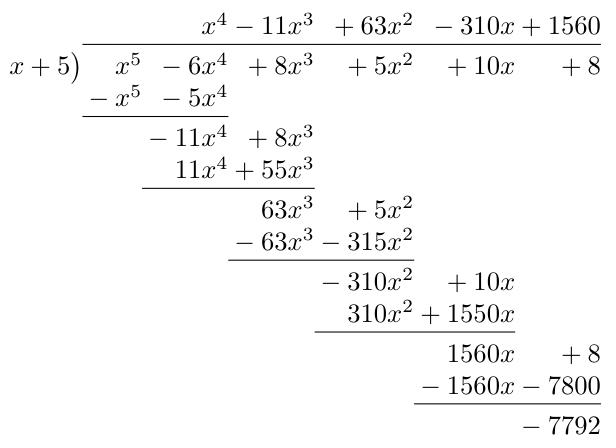多项式由不同程度的代数表达式组成。一阶多项式称为线性多项式,二阶多项式称为二次多项式,三阶多项式称为三次多项式。这些多项式的零是这些多项式变为零的点。有时候,我们有多项式的零点,我们需要找到其他零点。例如,假设多项式p(x)= x 3 – 3x 2 – x + 3,并且我们知道零为1。那么x – 1必须是该多项式的因数。目标是找到其他两个零。在这种情况下,除法算法可以为我们提供帮助。
多项式的除法算法
让我们有两个多项式p(x)和g(x),以及g(x)≠0。现在我们可以找到两个多项式q(x)和r(x),
p(x)= q(x)xg(x)+ r(x),
在此,r(x)= 0或r(x)的度 Dividend = Quotient x Divisor + Remainder 现在让我们来看一个例子,如何将两个多项式相除, 假设我们有p(x)= 2x 2 + 4x + 1和g(x)= x + 1。 长除法的步骤: Step 1: We will stop this process when the remainder becomes zero, or its degree becomes less than divisor. Step 2: Quotients’ first term is obtained by dividing the highest order term of dividend with the highest degree term of the divisor. Step 3: For the second term, divide the highest degree term of the new dividend obtained as remainder by the highest degree term of the divisor. Step 4: Continue the steps until the condition mentioned in step 1 is met. 请注意,在此示例中,q(x)= 2x + 2和r(x)= -1。 让我们看看这个算法的一些问题。 问题1:给定多项式p(x)= x 2 + x +5和g(x)= x +2。找出q(x)和r(x)的值。 解决方案: Using the steps mentioned above. On dividing p(x) with g(x) we get, q(x) = x – 1 and r(x) = 7. 问题2:给定多项式p(x)= x 3 + x + 6x 2 + 4和g(x)= x 2 +1。求出q(x)和r(x)的值。 解决方案: Using the steps mentioned above. On dividing p(x) with g(x) we get, So, here q(x) = x + 6, and r(x) = -2. 问题3:给定多项式p(x)= x 4 + x + 6x 2 + 4和g(x)= x 2 +1。求出q(x)和r(x)的值。 解决方案: Using the steps mentioned above. On dividing p(x) with g(x) we get, So, here q(x) = x2 + 5, and r(x) = x -1. 问题4:给定多项式x 4 –1。我们知道两个根为-1,1。找到另外两个根(如果存在)。 解决方案: We know that the two roots are -1 and 1. So, x -1 and x + 1 are the factors of the given polynomial. Then, (x – 1)(x +1) is also a factor of the polynomial. (x – 1) (x + 1) = x2 – 1 We see that quotient is x2 + 1. The roots of this polynomial will be the roots of the equation. x2 + 1 = 0 ⇒ x2 = -1 This equation cannot have real roots, thus roots don’t exist for this polynomial. 问题5:给定多项式p(x)= x 5 + 8x 3 – 6x 4 + 5x 2 + 10x + 8和g(x)= x 2 + 10x -5。找出q(x)和r(x)。 解决方案: Using the same steps as done in previous questions, q(x) = x3 – 16x2 + 173x – 1805 r(x) = 18295x – 9017 问题6:对于给定的多项式,相同的多项式p(x)= x 5 + 8x 3 – 6x 4 + 5x 2 + 10x + 8和g(x)= x +5。找到q(x)和r(x)。 解决方案: Here, q(x) = x4 – 11x3 + 63x2 – 310x + 1560 r(x) = -7792 问题7:对于相同的给定多项式p(x)= x 5 – 6x 4 + 5x 2 + 8和g(x)= x +2。找到q(x)和r(x)。 解决方案: q(x) = x4 – 8x3 + 16x2 -27x + 54 g(x) = -100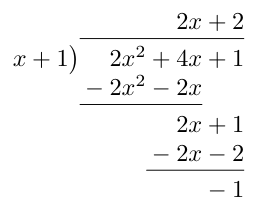
样本问题