在统计中,图形起着重要作用。借助这些图形,我们可以轻松理解数据。因此,在本文中,我们将学习如何以图形方式表示累积频率分布。
累积频率
频率是事件在给定情况下发生的次数,累积频率是所有先前频率到当前频率的总和。换句话说,一个类别的累积频率是通过将给定类别之前的所有类别的频率相加而计算出的频率。例如:
| Interval | Frequency |
| 0-10 | 2 |
| 10-20 | 4 |
| 20-30 | 5 |
在上表中,我们有间隔和频率,现在我们要通过将所有以前的频率与当前频率相加来找到累积频率。
| Interval | Frequency | Cumulative frequency |
| 0-10 | 2 | 2 |
| 10-20 | 4 | 6 |
| 20-30 | 5 | 11 |
因此,这里的interval(0-10)的累积频率为2,因为这是第一个频率。同样,间隔(10-20)的累积频率是6,因为它是2(先前频率)+ 4(当前频率)的总和,间隔(20-30)的累积频率是11,因为它是总和2 + 4(先前频率)+ 5(当前频率)。这种类型的表称为累积频率表。
累积频率曲线
让我们考虑将分组频率分布给予我们。拿一张方格纸,在x轴上标记上限,并在y轴上标记相应的累积频率。通过平滑曲线依次将这些点连接起来,我们将得到一条曲线,该曲线称为累积频率曲线。换句话说,累积频率分布的图形表示称为累积频率曲线。它也被称为ogive,它是表示数据的最有效方法。累积频率曲线有两种类型:
(1)小于累积频率曲线
众所周知,累积频率曲线是使用累积频率创建的,因此,在小于累积频率曲线的情况下,所有先前类别或间隔的频率都将添加到当前类别或间隔频率中。您可以通过将一级频率添加到二级频率,等等来创建多个累积频率。例如:
| Interval | Frequency |
| 5-10 | 2 |
| 10-15 | 4 |
| 15-20 | 5 |
在上表中,我们有间隔和频率,现在我们将所有以前的频率加到当前频率上,以找到小于累积频率的频率。
| Interval | Frequency | Cumulative frequency | Upper Limit |
| 5-10 | 2 | 2 | 10 |
| 10-15 | 4 | 2 + 4 = 6 | 15 |
| 15-20 | 5 | 2 + 4 + 5 = 11 | 20 |
如何绘制小于累积频率的曲线:
在这种情况下,我们使用类别的上限来绘制曲线。现在,逐步绘制小于累积频率曲线的过程:
- 拿一张方格纸,在x轴上标记上限,并在y轴上标记相应的累积频率。
- 通过线段依次连接这些点,我们将得到一个多边形,称为累积频率多边形。
- 通过平滑曲线依次将这些点连接起来,我们将得到一条曲线,称为累积频率图。
- 在y轴上取一个点A(0,N / 2)并绘制AP || x轴,在P点处切割上述曲线。在x轴上绘制PM⊥,在M处切割x轴。
- 然后,是OM的中值长度。

(2)超过累积频率曲线
众所周知,累积频率曲线是使用累积频率创建的,因此,在累加频率曲线之外,还可以将后继类别或间隔的频率添加到当前类别或间隔频率中。您可以通过从第一类中减去第二类的频率来创建多个累加频率,依此类推。例如:
| Interval | Frequency |
| 5-10 | 20 |
| 10-15 | 4 |
| 15-20 | 5 |
在上表中,我们有间隔和频率,现在我们将发现比累积频率更多的东西:
| Interval | Frequency | Cumulative frequency | Lower Limit |
| 5-10 | 20 | 20 | 5 |
| 10-15 | 4 | 20 – 4 = 16 | 10 |
| 15-20 | 5 | 16 – 5 = 11 | 15 |
如何绘制多于累积频率的曲线:
在这种情况下,我们使用类别的下限绘制曲线。现在,逐步绘制一条“累积频率”曲线以上的过程:
- 拿一张方格纸,在x轴上标记下限,在y轴上标记相应的累积频率。
- 通过线段依次连接这些点,我们将得到一个多边形,称为累积频率多边形。
- 通过平滑曲线依次将这些点连接起来,我们将得到一条曲线,称为累积频率图。
- 我们假定P是小于和大于曲线的交点。将PM⊥绘制到y轴,在M处切割x轴。
- 然后,中值= OM的长度。

样本问题
问题1.以下是小组学生的年龄分布。现在,绘制小于type的累积频率曲线并找到中值。
|
Age (in years) |
Frequency |
|---|---|
|
4-5 |
36 |
|
5-6 |
42 |
|
6-7 |
52 |
|
7-8 |
60 |
|
8-9 |
68 |
|
9-10 |
84 |
|
10-11 |
96 |
|
11-12 |
82 |
|
12-13 |
66 |
|
13-14 |
48 |
|
14-15 |
50 |
|
15-16 |
16 |
解决方案:
For the given table, we have to prepare the more than series as shown below:
On a graph paper, take the scale
Along the x-axis: 5 small div. = 1.
Along the y-axis: 1 small div. = 10.
And, plot all the points A(5, 36), B(6, 78), C(7, 130), D(8, 190), E(9, 258), F(10, 342), G(11, 438),
H(12, 520), I(13, 586), J(14, 634), K(15, 684) and L(16, 700).
Join these points successively with a freehand, we will get the cumulative frequency curve or an ogive.
Here, N = 700 ⇒ N/2 = 350

Take a point P(0, 350) on the y-axis and draw PQ|| x-axis, meeting the curve at Q. Draw QM 1 x-axis, intersecting x-axis at M. Then, OM = 10 units.
Hence, median = 10.
问题2。对于给定的频率分布,绘制一个大于类型的累积频率图,并找到中值。
|
Age (in years) |
c.f. |
|---|---|
|
Less than 5 |
36 |
|
Less than 6 |
78 |
|
Less than 7 |
130 |
|
Less than 8 |
190 |
|
Less than 9 |
258 |
|
Less than 10 |
342 |
|
Less than 11 |
438 |
|
Less than 12 |
520 |
|
Less than 13 |
586 |
|
Less than 14 |
634 |
|
Less than 15 |
684 |
|
Less than 16 |
700 |
解决方案:
For the given table, we have to prepare the more than series as shown below:
Scale: Along the x-axis, 10 small div. = 5.
Along the y-axis, 1 small div.= 1.
Plot all the points A(5, 100), B(10, 95), C(20, 80), D(30, 60), E(40, 37), F(50, 20) and G(60, 9).
Join AB, BC, CD, DE, EF and FG with a freehand, and we will get the required curve, as shown in below figure.
Here, N = 100
⇒ N/2 = 50

From P(0, 50) draw PQ || x-axis, meeting the curve at Q. Draw QM ⊥ OZ, meeting x-axis at M. Clearly, OM = 35 units
Hence, median = 35.
问题3.下表列出了一个村庄的100个农场的大米产量:
|
Class Interval |
0-10 |
10-20 |
20-30 |
30-40 |
40-50 |
50-60 |
60-70 |
|---|---|---|---|---|---|---|---|
|
Frequency |
5 |
15 |
20 |
23 |
17 |
11 |
9 |
绘制一个大于类型的累积频率图。
解决方案:
For the given table, we have to prepare the more than series as shown below:
|
More than 65 |
24 |
|
More than 60 |
54 |
|
More than 55 |
74 |
|
More than 50 |
90 |
|
More than 45 |
96 |
|
More than 40 |
100 |
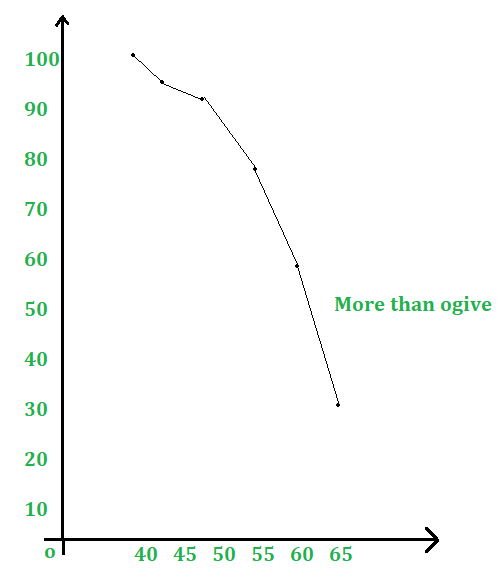
Scale: Along the x-axis, 1 small div.= 1
Along the y-axis, 1 small div. = 1
On a graph paper, plot all the points A(40, 100), B(45, 96), C(50, 90), D(55, 74), E(60, 54) and F(65, 24).
Join AB, BC, CD, DE and EF with a free hand, and we will get a More Than Ogive.
问题4.在对一所大学的35名学生进行身体检查时,他们的体重记录如下:
|
More than 60 |
9 |
|
More than 50 |
20 |
|
More than 40 |
37 |
|
More than 30 |
60 |
|
More than 20 |
80 |
|
More than 10 |
95 |
|
More than 5 |
100 |
从给定的数据中得出小于和大于类型的输入。因此,从图表中找到体重的中位数。
解决方案:
(i) Less than Series:
For the given table, we have to prepare the less than series as shown below:
|
Weight (in kg) |
Number of Students |
|---|---|
|
Less than 40 |
3 |
|
Less than 42 |
5 |
|
Less than 44 |
9 |
|
Less than 46 |
14 |
|
Less than 48 |
28 |
|
Less than 50 |
32 |
|
Less than 52 |
35 |
Scale: Along the x-axis, 5 small div. = 1 kg.
Along the y-axis, 10 small div.= 5 kg.
Plot all the points A(40, 3), B(42, 5), C(44, 9), D(46, 14), E(48, 28), F(50, 32) and G(52, 35).
Join AB, BC, CD, DE, EF and FG with a free hand to get the curve ‘Less Than Series’.
(ii) More than Series:
For the given table, we have to prepare the more than series as shown below:
|
Weight (in kg) |
Number of Students |
|---|---|
|
More than 38 |
35 |
|
More than 40 |
32 |
|
More than 42 |
30 |
|
More than 44 |
26 |
|
More than 46 |
21 |
|
More than 48 |
7 |
|
More than 50 |
3 |
Now plot the points on the same graph: P(38,35), Q(40, 32), R(42, 30), S(44, 26), T(46, 21), U(48, 7) and V(50,3)
and join PQ, QR, RS, ST, TU and UV with a free hand to get ‘More Than Series’.

The two curves intersect at the point L. Draw LM ⊥ OX.
Hence, median weight = OM = 46.5 kg.