3D空间中的坐标轴和坐标平面

在平面上，我们知道我们需要两条相互垂直的线来定位点的位置。这些线称为平面的坐标轴，而该平面通常称为笛卡尔平面。但是在现实生活中，我们没有这样的飞机。在现实生活中，要定位物体，我们需要一些额外的信息，例如高度。因此，现在我们需要三件事来定位现实生活中的一个点-x，y及其高度，通常用z表示。这些被称为关于三维空间的坐标。

坐标轴和坐标平面

在图中，我们可以看到三个平面相交。这些平面彼此垂直。线XOX’，Y’OY和Z’OZ表示所有平面彼此的交点。这些线分别称为x轴，y轴和z轴，它们构成3D直角坐标系。

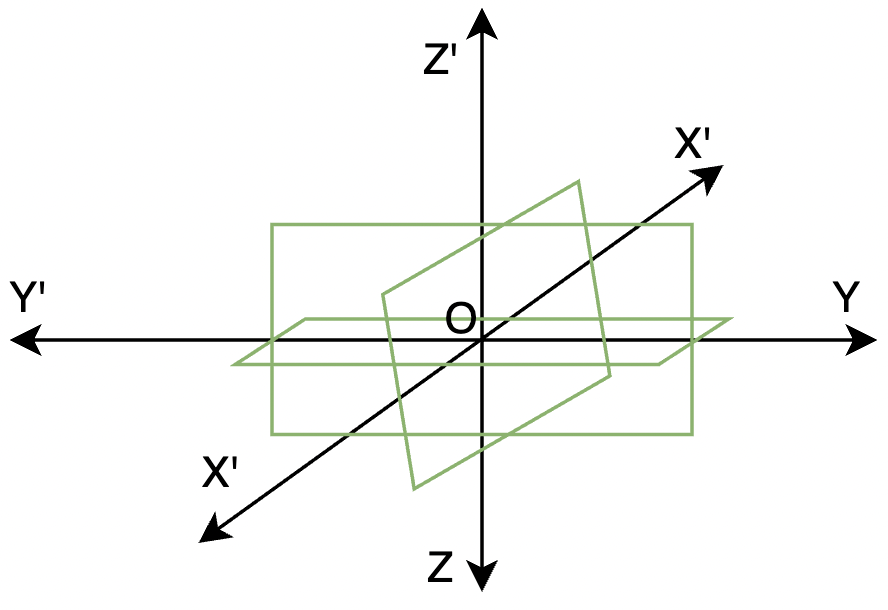
座标平面

平面XOY，YOZ和ZOX分别称为XY平面，YZ平面和ZX平面。所有平面的交点称为原点。这些平面将3-D空间划分为8个八分圆。

空间点的坐标

假定3D空间中的任何点都具有三个坐标，分别表示x，y和z坐标的值。在下图中，我们得到了一个位于空间中的点A(x，y，z)。我们从A到xy平面到点M的垂线放下。AM的长度为坐标z的值。在图中，LM和OL给出了y和x坐标的值。

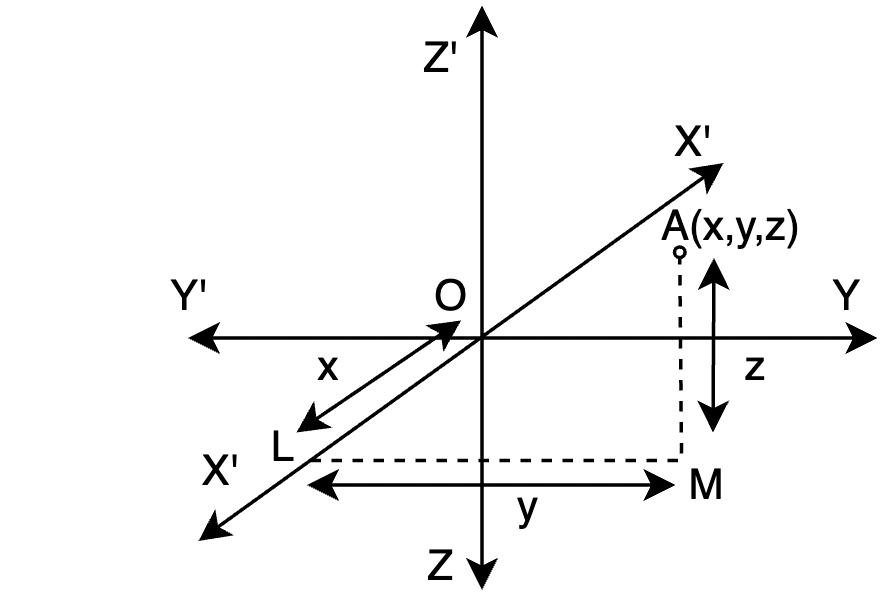
因此，对于空间中存在的任何点，都存在一个有序三元组(x，y，z)，该三元组给出了该点在空间中的位置。

Coordinates of the origin are (0, 0, 0). A point on x-axis is of the form (x, 0, 0), same goes for the points on y-axis and z-axis. 

两点之间的距离

假设我们在3-D空间中有两个点(x ₁ ，y ₁ ，z ₁ )和(x ₂ ，y ₂ ，z _2)。计算3-D空间中两点之间的距离的公式类似于我们针对2-D空间研究的距离的Euclid公式。该公式是对Euclid给出的原始公式的略微修改。

The distance between two points (x₁, y₁, z₁) and (x₂, y₂, z₂) is given by, 

$\sqrt{(x_1 - y_1)^2 + (x_2 - y_2)^2 + (x_3 - y_3)^2}$

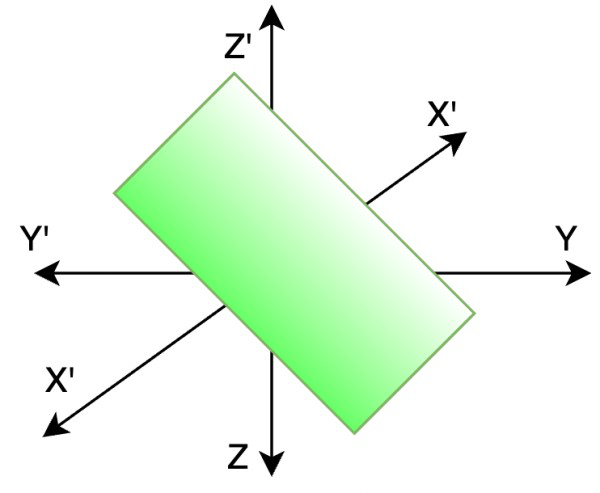
飞机

平面是无限延伸的二维平面。它是3维的，类似于直线2维和一维空间中的一个点。绘制平面很难，如果我们在纸上写东西，那也是平面。我们在飞机上写作。下图显示了3维空间中的平面。

Let’s say we have a plane, it intersects the x-axis at “a”, y-axis at “b” and the z-axis at “c”. Its equation is given by, 

$\frac{x}{a} + \frac{y}{b} + \frac{z}{c} = 1$

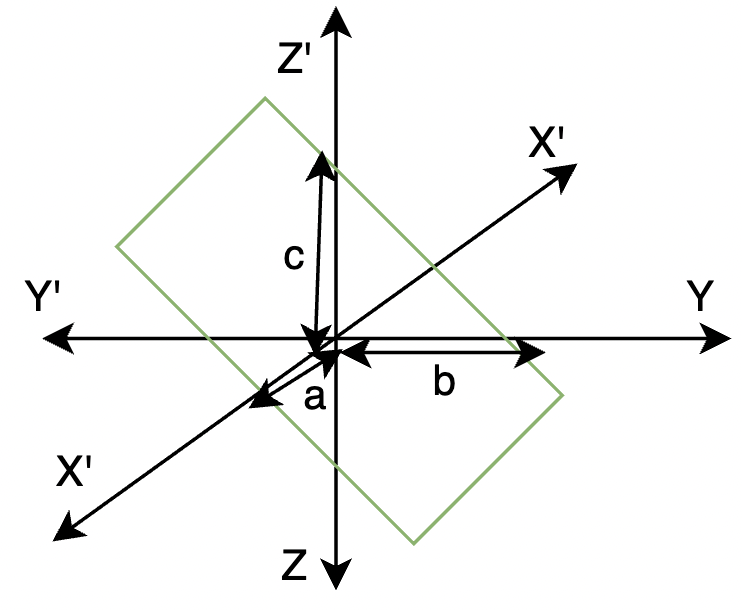
This is called intercept form of the equation of plane. 

样本问题

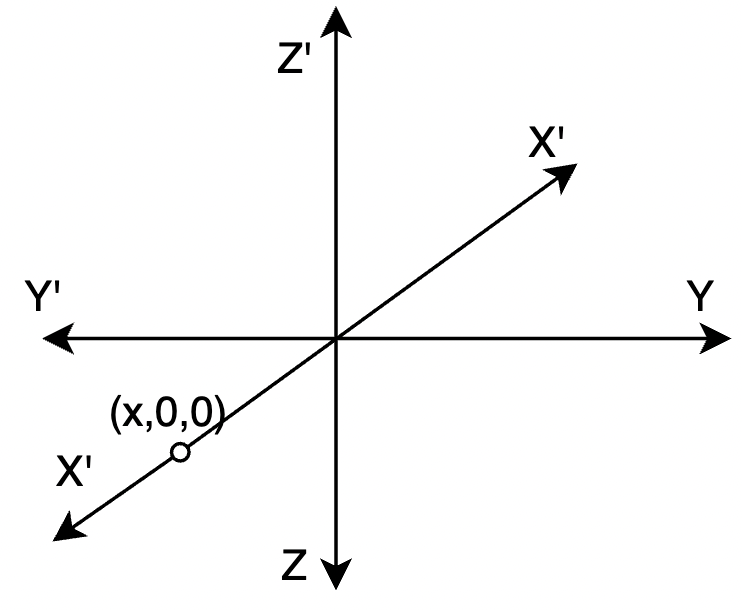
问题1：假设我们在x轴上有一个点，它的y坐标和z坐标是什么?

解决方案：

In the figure, the point lies on x-axis. It can be noticed that it’s coordinates for y and z are equal to zero. 

问题2：填空：

1. X和Y轴共同构成_____平面。
2. 所有坐标平面将3d空间划分为_______个八分圆。

回答：

1. X and Y axis together make XY plane. 

2. All the coordinates planes divide the 3-D space into eight octants. 

问题3：计算(0,0,0)与(5,4,3)之间的距离。

解决方案：

For the points (x₁, y₁, z₁) and (x₂, y₂, z₂) 

$\sqrt{(x_1 - y_1)^2 + (x_2 - y_2)^2 + (x_3 - y_3)^2}$

Here, (x₁, y₁, z₁) = (0,0,0) and (x₂, y₂, z₂) = (5,,4,3). Let the distance be “l” 

l = $\sqrt{(x_1 - y_1)^2 + (x_2 - y_2)^2 + (x_3 - y_3)^2}$

= $\sqrt{(5 - 0)^2 + (4 - 0)^2 + (3 - 0)^2}$

= $\sqrt{25 + 16 + 9}$

= $\sqrt{50}$

= 5√2 

问题4：计算(0,0,0)与(1,2,3)之间的距离。

解决方案：

For the points (x₁, y₁, z₁) and (x₂, y₂, z₂) 

$\sqrt{(x_1 - y_1)^2 + (x_2 - y_2)^2 + (x_3 - y_3)^2}$

Here, (x₁, y₁, z₁) = (0,0,0) and (x₂, y₂, z₂) = (1,2,3). Let the distance be “l” 

l = $\sqrt{(x_1 - y_1)^2 + (x_2 - y_2)^2 + (x_3 - y_3)^2}$

= $\sqrt{1 + 4 + 9}$

= $\sqrt{1 + 9 + 4}$

= $\sqrt{14}$

问题5：计算(1,1,1)与(2,4,3)之间的距离。

解决方案：

For the points  (x₁, y₁, z₁) and (x₂, y₂, z₂) 

$\sqrt{(x_1 - y_1)^2 + (x_2 - y_2)^2 + (x_3 - y_3)^2}$

Here, (x₁, y₁, z₁) = (1,1,1) and (x₂, y₂, z₂) = (2,4,3). Let the distance be “l” 

l = $\sqrt{(x_1 - y_1)^2 + (x_2 - y_2)^2 + (x_3 - y_3)^2}$

= $\sqrt{(2 - 1)^2 + (4 - 1)^2 + (3 - 1)^2}$

= $\sqrt{1^2 + 3^2 + 2^2}$

= $\sqrt{14}$ 

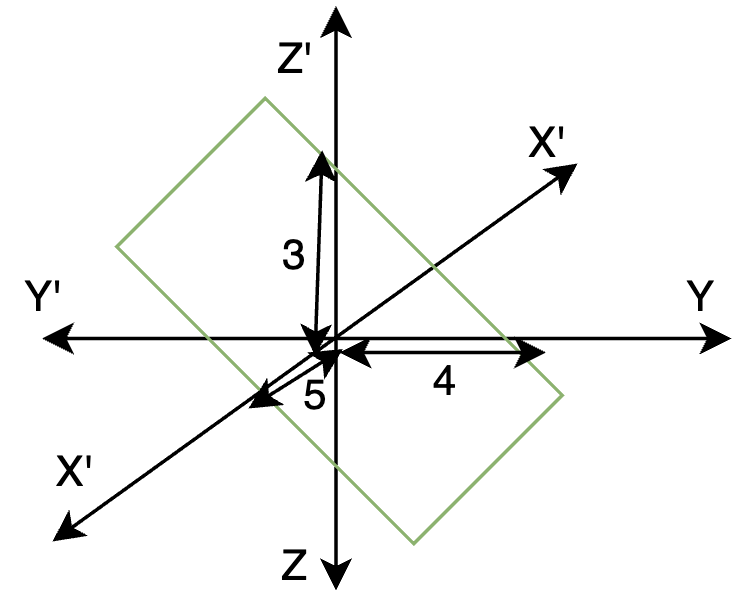
问题6：在下图中，找到平面方程。

解决方案：

We know the intercept form of an equation of a plane. Let’s say we have a plane, it intersects the x-axis at “a”, y-axis at “b” and the z-axis at “c”. It’s equation is given by, 

$\frac{x}{a} + \frac{y}{b} + \frac{z}{c} = 1$

Notice in the figure, a = 5, b = 4 and c = 3 

So, the equation of the plane becomes, 

$\frac{x}{5} + \frac{y}{4} + \frac{z}{3} = 1$

= $\frac{12x + 15y + 20z}{60} = 1$

= $12x + 15y + 20z = 60$