📌  相关文章
📜  价差量度–范围，方差和标准偏差

📅  最后修改于: 2021-06-23 04:21:27             🧑  作者: Mango

收集数据并以表格，图表和其他分布形式表示对我们来说至关重要。但是，同样重要的是，我们必须对数据的分布方式，分散程度以及数据的含义有一个公正的认识。均值的度量还不足以描述数据及其性质。我们还需要针对数据的不同统计量来测量数据中的离散度。例如，我们需要能够回答以下问题：周围数据的均值或其中位数的分散程度是多少。这些值使我们可以更好地描述数据。让我们来看一些

分散度(也称为分散度)是根据选定的中心趋势的类型和我们可用的观测值进行测量的。这些度量告诉我们观察值有多少不同或彼此相似。有许多方法可以测量数据的离散度，以下是一些主要的方法来测量散度：

  1. 范围
  2. 方差
  3. 标准偏差

范围

数据范围以数据中观测值的最大值和最小值之差给出。举例来说，假设我们有一周内在商店中走访的顾客数量的数据。

10、14、8、10、15、4、7

数据最小值= 7

数据中的最大值= 15

范围=数据中的最大值–数据中的最小值

= 15 – 7

= 8

现在我们可以说数据的范围是8。这使我们对数据的分布有一个了解，但没有说明数据的分布方式。

方差

通过测量观测值与分布平均值的距离来给出数据的方差。在这里，我们与点的距离的符号无关，而对幅度更感兴趣。因此，我们采用距均值的距离的平方。假设我们有x 1 ，x 2 ，x 3 …。 x n作为n个观测值，并且\bar{x}是卑鄙的。

(x_1 - \bar{x})^2 + (x_2 - \bar{x})^2 + (x_3 - \bar{x})^2 .... (x_n - \bar{x})^2 = \sum^{n}_{0}(x_i - \bar{x})^2

如果该总和为零，则每个项必须为零，这意味着数据中没有散射。如果很小，则意味着对于大的方差值，数据集中在平均值上，反之亦然。

但是，此度量仍然取决于数据中的观察次数。也就是说，如果有很多观察，则该值将变大。因此，我们取数据的平均值，

\text{Variance} = \frac{\sum^{n}_{0}(x_i - \bar{x})^2}{n}\\ \sigma^2 = \frac{\sum^{n}_{0}(x_i - \bar{x})^2}{n}

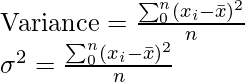
标准偏差

在计算方差时，请注意方差的单位和观察值的单位不相同。因此，为消除此问题，我们定义了标准偏差。表示为\sigma

\sigma = \sqrt{\frac{\sum^{n}_{0}(x_i - \bar{x})^2}{n}}

让我们看看如何在某些问题中计算这些度量，

样本问题

问题1：找出以下数据的范围：

-4  5 -10 6 9

解决方案：

问题2：找出相同数据的均值和中位数：

-4  5 -10 6 9

解决方案：

问题3：假设我们有以下数据，

-4 -2 0 -2 6 4 6 0 -6 4

计算数据的范围，方差和标准偏差。

解决方案：

问题4：假设我们有以下数据，

-3 -3 -3 -3 0 3 3 3 3

计算数据的范围，方差和标准偏差。

解决方案：

问题5：假设我们有以下有关消费电子商店一周内销售的电视数量的数据，

Monday  4
Tuesday 5
Wednesday 3
Thursday 4
Friday 5
Saturday 5
Sunday 3

计算数据的范围，方差和标准偏差。

解决方案：