零件集成

在微积分中，对于每种函数，都有一个公式可以直接评估它们的积分。但是，如果要求我们找出两个函数的乘积的整数，该怎么办?部件集成是解决此问题的方法。零件积分的公式为：

∫uv dx = u∫v dx − ∫((du/dx)∫v dx) dx

Where:

• u is the first function of x: u(x)
• v is the second function of x: v(x)

顾名思义，在“部分集成”中，我们首先检查哪种两种功能组成了要集成的给定表达式。然后，我们根据称为ILATE规则的简单规则检查其优先级。此规则有助于我们确定哪个函数应被视为x的第一个函数u(x)，哪个函数应被称为第二个函数v(x)。

ILATE规则

ILATE是下面列出的函数类型的首字母缩写。在对给定的两种功能，这是上在分级结构中的函数比较被视为第一函数，而另一个作为第二函数。

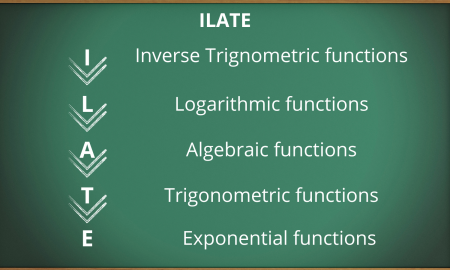
找到u(x)和v(x)之后的步骤

1. 相对于x区分u(x)，即评估du / dx。
2. 将v(x)相对于x积分，即评估∫vdx。
3. 在公式中使用在步骤1和2中获得的结果： ∫uvdx =u∫vdx −∫((du / dx)∫vdx)dx

例子

使用“按零件”规则查找以下表达式的集成：

问题1.∫e ^x x dx

解决方案：

Choosing u and v, 

u = x and v = e^x

Differentiating u:

u'(x) = d(u)/dx

       = d(x)/dx

      = 1

Using the formula,

∫ e^x x dx = x ∫e^x dx − ∫1 (∫ e^x dx) dx

         = xe^x − e^x + C

         = e^x(x − 1) + C

问题2.∫x sin x dx

解决方案：

Choosing u and v,

u = x and v = sin x

Differentiating u:

u'(x) = d(u)/dx

       = d(x)/dx

       = 1

Using the formula, 

∫ x sin x dx = x ∫sin x dx − ∫1 ∫(sin x dx) dx

                  = − x cos x − ∫−cos x dx

                  = − x cos x + sin x + C

问题3.∫ln x dx

解决方案：

Since here is only one function, thus we need to assume the other function so that the integration by parts’ formula can be applied to it. Also, the questions having the logarithmic functions can be solved only by parts.

Choosing u and v,

u = ln x and v = 1 

Differentiating u:

u'(x) = d(u)/dx

       = d(ln x)/dx

      = 1/x

Using the formula, 

∫ ln x dx = ln x∫ 1 dx − ∫ 1/x ∫(1 dx) dx

              = x ln x −  ∫(1/x) x  dx       [since, ∫ 1 dx = x ]

              = x ln x − ∫ 1 dx

              = x ln x − x + C

              = x (ln x − 1) + C

问题4.∫sin ^-1 x dx

解决方案：

Since here is only one function, thus we need to assume the other function so that the integration by parts’ formula can be applied to it.

Choosing u and v,

u = sin⁻¹ x  and  v = 1

Differentiating u:

u'(x) = d(u)/dx

        = d(sin⁻¹ x )/dx 

        = 1/√(1 − x ²) 

Using the formula, 

∫ sin⁻¹ x dx = sin⁻¹ x ∫ 1 dx  − ∫ 1/√(1 − x ²)  ∫(1 dx)  dx

                   = x sin⁻¹ x  − ∫( x/√(1 − x ² ) )dx

                  Here, assuming t = 1 − x ² and on differentiating both sides,

                                         dt = −2x dx

                                        −dt/2 = x dx

∫ sin⁻¹ x dx =  x sin⁻¹ x  − ∫−(1/2√t ) dt

                   = x sin⁻¹ x  + 1/2∫t^−1/2 dt

                   = x sin⁻¹ x + t^1/2 + C

                   = x sin⁻¹ x + √(1 − x² ) + C