11类RD Sharma解决方案–第8章转换公式–练习8.2 |套装2

问题11。如果secsec A + sec A = secsec B + sec B，则证明tan A tan B = 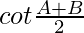$cot\frac{A+B}{2}$ 。

解决方案：

We have, cosec A + sec A = cosec B + sec B

=> sec A − sec B = cosec B − cosec A

=>$\frac{1}{cosA}-\frac{1}{cosB}=\frac{1}{sinB}-\frac{1}{sinA}$

=>$\frac{cosB-cosA}{cosAcosB}=\frac{sinA-sinB}{sinAsinB}$

=>$\frac{sinAsinB}{cosAcosB}=\frac{sinA-sinB}{cosB-cosA}$

=> tan A tan B =$\frac{2cos\frac{A+B}{2}sin\frac{A-B}{2}}{2sin\frac{A+B}{2}sin\frac{A-B}{2}}$

=> tan A tan B =$cot\frac{A+B}{2}$

Hence proved.

问题12.如果sin 2A =λsin 2B，则证明$\frac{tan(A+B)}{tan(A-B)}=\frac{λ+1}{λ-1}$ 。

解决方案：

We are given, sin 2A = λ sin 2B

=>$\frac{sin2A}{sin2B}=λ$

On applying Componendo and Dividendo, we get,

=>$\frac{sin2A+sin2B}{sin2A-sin2B}=\frac{λ+1}{λ-1}$

=>$\frac{2sin\frac{2A+2B}{2}cos\frac{2A-2B}{2}}{2cos\frac{2A+2B}{2}sin\frac{2A-2B}{2}}=\frac{λ+1}{λ-1}$

=>$\frac{sin(A+B)cos(A-B)}{cos(A+B)sin(A-B)}=\frac{λ+1}{λ-1}$

=>$tan(A+B)cot(A-B)=\frac{λ+1}{λ-1}$

=>$\frac{tan(A+B)}{tan(A-B)}=\frac{λ+1}{λ-1}$

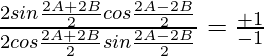
Hence proved.

问题13：证明：

(一世) 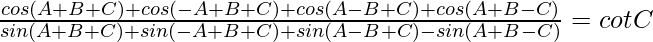$\frac{cos(A+B+C)+cos(-A+B+C)+cos(A-B+C)+cos(A+B-C)}{sin(A+B+C)+sin(-A+B+C)+sin(A-B+C)-sin(A+B-C)}=cotC$

解决方案：

We have,

L.H.S. =$\frac{cos(A+B+C)+cos(-A+B+C)+cos(A-B+C)+cos(A+B-C)}{sin(A+B+C)+sin(-A+B+C)+sin(A-B+C)-sin(A+B-C)}$

=$\frac{2cos\frac{A+B+C-A+B+C}{2}cos\frac{A+B+C+A-B-C}{2}+2cos\frac{A-B+C+A+B-C}{2}cos\frac{A-B+C-A-B+C}{2}}{2sin\frac{A+B+C-A+B+C}{2}cos\frac{A+B+C+A-B-C}{2}+2cos\frac{A-B+C+A+B-C}{2}sin\frac{A-B+C-A-B+C}{2}}$

=$\frac{2cos(B+C)cosA+2cosAcos(C-B)}{2sin(B+C)cosA+2cosAsin(C-B)}$

=$\frac{2cosA(cos(B+C)+cos(C-B))}{2cosA(sin(B+C)+sin(C-B))}$

=$\frac{cos(B+C)+cos(C-B)}{sin(B+C)+sin(C-B)}$

=$\frac{2cos\frac{B+C+C-B}{2}cos\frac{B+C-C+B}{2}}{2sin\frac{B+C+C-B}{2}cos\frac{B+C-C+B}{2}}$

=$\frac{cosCcosB}{sinCcosB}$

=$\frac{cosC}{sinC}$

= cot C

= R.H.S.

Hence proved.

(ii)sin(BC)cos(AD)+ sin(CA)cos(BD)+ sin(AB)cos(CD)= 0

解决方案：

We have, L.H.S. = sin (B−C) cos (A−D) + sin (C−A) cos (B−D) + sin (A−B) cos (C−D)

=$\frac{1}{2}[2sin (B−C) cos (A−D) + 2sin (C−A) cos (B−D) + 2sin (A−B) cos (C−D)]$

=$\frac{1}{2}[sin(B−C+A-D)+sin(B−C-A+D)+sin(C−A+B-D)+sin(C-A-B+D)+sin(A−B+C-D)+sin(A-B-C+D)]$

=$\frac{1}{2}[sin(A+B−C-D)-sin(A+C-B-D)-sin(A+D-B-C)-sin(A+B-C-D)+sin(A+C−B-D)+sin(A+D-B-C)]$

=$\frac{1}{2}[0]$

= 0

= R.H.S.

Hence proved.

问题14.如果$\frac{cos(A-B)}{cos(A+B)}+\frac{cos(C+D)}{cos(C-D)}=0$ ，证明tan A tan B tan C tan D = -1。

解决方案：

We have,

=>$\frac{cos(A-B)}{cos(A+B)}+\frac{cos(C+D)}{cos(C-D)}=0$

=>$\frac{cos(A-B)}{cos(A+B)}=-\frac{cos(C+D)}{cos(C-D)}$

=>$\frac{cos(A-B)}{cos(A+B)}+1=-\frac{cos(C+D)}{cos(C-D)}+1$

=>$\frac{cos(A-B)+cos(A+B)}{cos(A+B)}=\frac{-cos(C+D)+cos(C-D)}{cos(C-D)}$

=>$\frac{cos(A-B)+cos(A+B)}{cos(A+B)}=\frac{-[cos(C+D)-cos(C-D)]}{cos(C-D)}$

=>$\frac{2cos\frac{A+B+A-B}{2}cos\frac{A+B-A+B}{2}}{cos(A+B)}=\frac{-[-2sin\frac{C+D+C-D}{2}sin\frac{C+D-C+D}{2}]}{cos(C-D)}$

=>$\frac{2cosAcosB}{cos(A+B)}=\frac{2sinCsinD}{cos(C-D)}$

=>$\frac{cosAcosB}{cos(A+B)}=\frac{sinCsinD}{cos(C-D)}$. . . . (1)

Also,$\frac{cos(A-B)}{cos(A+B)}=-\frac{cos(C+D)}{cos(C-D)}$

=>$\frac{cos(A-B)}{cos(A+B)}-1=-\frac{cos(C+D)}{cos(C-D)}-1$

=>$\frac{cos(A-B)-cos(A+B)}{cos(A+B)}=\frac{-[cos(C+D)+cos(C-D)]}{cos(C-D)}$

=>$\frac{-2sin\frac{A+B+A-B}{2}sin\frac{A+B-A+B}{2}}{cos(A+B)}=\frac{2cos\frac{C+D+C-D}{2}cos\frac{C+D-C+D}{2}}{cos(C-D)}$

=>$\frac{-2sinAsinB}{cos(A+B)}=\frac{2cosCcosD}{cos(C-D)}$

=>$\frac{-sinAsinB}{cos(A+B)}=\frac{cosCcosD}{cos(C-D)}$. . . . (2)

Dividing (1) by (2), we get,

=>$\frac{\frac{cosAcosB}{cos(A+B)}}{\frac{-sinAsinB}{cos(A+B)}}=\frac{\frac{sinCsinD}{cos(C-D)}}{\frac{cosCcosD}{cos(C-D)}}$

=>$\frac{cosAcosB}{-sinAsinB}=\frac{sinCsinD}{cosCcosD}$

=>$\frac{-1}{tanAtanB}=tanCtanD$

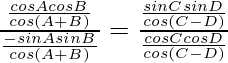
=> tan A tan B tan C tan D = −1

Hence proved.

问题15.如果cos( α+β)sin(γ+δ)= cos(α-β)sin(γ-δ)，则证明cotαcotβcotγ= cotδ。

解决方案：

We have, cos (α+β) sin(γ+δ) = cos (α−β) sin(γ−δ)

=>$\frac{cos(α+β)}{cos(α−β)}=\frac{sin(γ-δ)}{sin(γ+δ)}$

=>$\frac{cos(α+β)}{cos(α−β)}+1=\frac{sin(γ-δ)}{sin(γ+δ)}+1$

=>$\frac{cos(α+β)+cos(α−β)}{cos(α−β)}=\frac{sin(γ-δ)+sin(γ+δ)}{sin(γ+δ)}$

=>$\frac{2cosαcosβ}{cos(α−β)}=\frac{2sinγcosδ}{sin(γ+δ)}$

=>$\frac{cosαcosβ}{cos(α−β)}=\frac{sinγcosδ}{sin(γ+δ)}$. . . . (1)

Also,$\frac{cos(α+β)}{cos(α−β)}=\frac{sin(γ-δ)}{sin(γ+δ)}$

=>$\frac{cos(α+β)}{cos(α−β)}-1=\frac{sin(γ-δ)}{sin(γ+δ)}-1$

=>$\frac{cos(α+β)-cos(α−β)}{cos(α−β)}=\frac{sin(γ-δ)-sin(γ+δ)}{sin(γ+δ)}$

=>$\frac{-2sinαcosβ}{cos(α−β)}=\frac{-2cosγsinδ}{sin(γ+δ)}$

=>$\frac{sinαsinβ}{cos(α−β)}=\frac{cosγsinδ}{sin(γ+δ)}$. . . . (2)

Dividing (1) by (2), we get,

=>$\frac{\frac{cosαcosβ}{cos(α−β)}}{\frac{sinαsinβ}{cos(α−β)}}=\frac{\frac{sinγcosδ}{sin(γ+δ)}}{\frac{cosγsinδ}{sin(γ+δ)}}$

=>$\frac{cosαcosβ}{sinαsinβ}=\frac{sinγcosδ}{cosγsinδ}$

=> cot α cot β = tan γ cot δ

=> cot α cot β cot γ = cot δ

Hence proved.

问题16。如果y sinØ= x sin(2θ +Ø)，则证明(x + y)cot( θ +Ø)=(y − x)cotθ 。

解决方案：

Given, y sin Ø = x sin (2θ + Ø)

=>$\frac{sinØ}{sin (2θ + Ø)}=\frac{x}{y}$

On applying Componendo and Dividendo, we get,

=>$\frac{sinØ+sin(2θ+Ø)}{sinØ-sin(2θ+Ø))}=\frac{x+y}{x-y}$

=>$\frac{2sin\frac{Ø+2θ+Ø}{2}cos\frac{Ø-2θ-Ø}{2}}{2cos\frac{Ø+2θ+Ø}{2}sin\frac{Ø-2θ-Ø}{2}}=\frac{x+y}{x-y}$

=>$\frac{2sin(Ø+θ)cosθ}{-2cos(Ø+θ)sinθ}=\frac{x+y}{x-y}$

=>$\frac{sin(Ø+θ)cosθ}{cos(Ø+θ)sinθ}=\frac{x+y}{y-x}$

=> tan (Ø+θ) cot θ =$\frac{x+y}{y-x}$

=>$\frac{cotθ}{cot(Ø+θ)}=\frac{x+y}{y-x}$

=> (y − x) cot θ = (x + y) cot (θ + Ø)

=> (x + y) cot (θ + Ø) = (y − x) cot θ

Hence proved.

问题17.如果cos(A + B)sin(C − D)= cos(AB)sin(C + D)，则证明tan A tan B tan C + tan D = 0。

解决方案：

We are given, cos (A+B) sin (C−D) = cos (A−B) sin (C+D)

=>$\frac{cos(A+B)}{cos (A−B)}=\frac{sin(C+D)}{sin(C-D)}$

On applying Componendo and Dividendo, we get,

=>$\frac{cos(A+B)+cos(A−B)}{cos(A+B)-cos(A−B)}=\frac{sin(C+D)+sin(C-D)}{sin(C+D)-sin(C-D)}$

=>$\frac{2cosAcosB}{-2sinAsinB}=\frac{2sinCcosD}{2cosCsinD}$

=>$\frac{-cosAcosB}{sinAsinB}=\frac{sinCcosD}{cosCsinD}$

=>$\frac{-1}{tanAtanB}=\frac{tanC}{tanD}$

=> −tan D = tan A tan B tan C

=> tan A tan B tan C + tan D = 0

Hence proved.

问题18 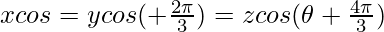$xcosθ=ycos(θ+\frac{2\pi}{3})=zcos(\theta+\frac{4\pi}{3})$ ，证明xy + yz + zx = 0。

解决方案：

We have,$xcosθ=ycos(θ+\frac{2\pi}{3})=zcos(\theta+\frac{4\pi}{3})$= k (say)

x =$\frac{k}{cos\theta}$

y =$\frac{k}{cos(\theta+\frac{2\pi}{3})}$

z =$\frac{k}{cos(\theta+\frac{4\pi}{3})}$

So, L.H.S. = xy + yz + zx

=$k^2\left[\frac{1}{cos\theta cos(\theta+\frac{2\pi}{3})}+\frac{1}{cos(\theta+\frac{2\pi}{3}) cos(\theta+\frac{4\pi}{3})}+\frac{1}{cos\theta cos(\theta+\frac{4\pi}{3})}\right]$

=$k^2\left[\frac{cos(\theta+\frac{4\pi}{3})+cos\theta +cos(\theta+\frac{2\pi}{3})}{cos\theta cos(\theta+\frac{2\pi}{3})cos(\theta+\frac{4\pi}{3})}\right]$

=$k^2\left[\frac{cos\theta cos\frac{4\pi}{3}-sin\theta sin\frac{4\pi}{3}+cos\theta +cos\theta cos\frac{2\pi}{3}-sin\theta sin\frac{2\pi}{3}}{cos\theta cos(\theta+\frac{2\pi}{3})cos(\theta+\frac{4\pi}{3})}\right]$

=$k^2\left[\frac{cos\theta(\frac{-1}{2})-sin\theta(\frac{-\sqrt{3}}{2})+cos\theta +cos\theta (\frac{-1}{2})-sin\theta (\frac{\sqrt{3}}{2})}{cos\theta cos(\theta+\frac{2\pi}{3})cos(\theta+\frac{4\pi}{3})}\right]$

=$k^2\left[\frac{-cos\theta+sin\theta(\frac{\sqrt{3}}{2})+cos\theta -sin\theta (\frac{\sqrt{3}}{2})}{cos\theta cos(\theta+\frac{2\pi}{3})cos(\theta+\frac{4\pi}{3})}\right]$

=$k^2\left[\frac{0}{cos\theta cos(\theta+\frac{2\pi}{3})cos(\theta+\frac{4\pi}{3})}\right]$

= 0

= R.H.S.

Hence proved.

问题19.如果m sinθ = n sin( θ + 2a)，则证明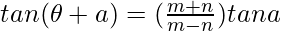$tan(\theta+a)=(\frac{m+n}{m-n})tana$ 。

解决方案：

We are given, m sin θ = n sin (θ + 2a)

=>$\frac{sin(θ+2a)}{sin θ}=\frac{m}{n}$

On applying Componendo and Dividendo, we get,

=>$\frac{sin(θ+2a)+sinθ}{sin(θ+2a)-sinθ}=\frac{m+n}{m-n}$

=>$\frac{2sin\frac{θ+2a+θ}{2}cos\frac{θ+2a-θ}{2}}{2cos\frac{θ+2a+θ}{2}sin\frac{θ+2a-θ}{2}}=\frac{m+n}{m-n}$

=>$\frac{2sin(θ+a)cosa}{2cos(θ+a)sina}=\frac{m+n}{m-n}$

=>$\frac{tan(θ+a)}{tana}=\frac{m+n}{m-n}$

=>$tan(\theta+a)=(\frac{m+n}{m-n})tana$

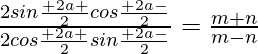
Hence, proved.