简单曲线下的面积

我们知道如何计算一些标准曲线的面积，例如矩形，正方形，梯形等。每个图都有面积公式，但是在现实生活中，这些图并不总是完美的。有时可能会遇到一个看起来像正方形但实际上不是一个完美正方形的图形，或者可能会遇到一个完全不同的图形，我们必须为其计算面积。在这种情况下，积分可以帮助我们。积分使我们能够根据给定的方程式来计算任意曲线的面积。让我们详细了解该过程。

简单曲线下面积的公式

积分可以看作是一个和，而定积分可以通过微积分的基本定理求值。在下图中，我们可以看到任意条带，其长度为“ y”，宽度为“ dx”。 “ ydx”可以近似为阴影矩形的面积。该区域称为基本区域。当我们从a移到b时，我们可以继续添加这些区域，然后得到ABCD区域以下的区域。

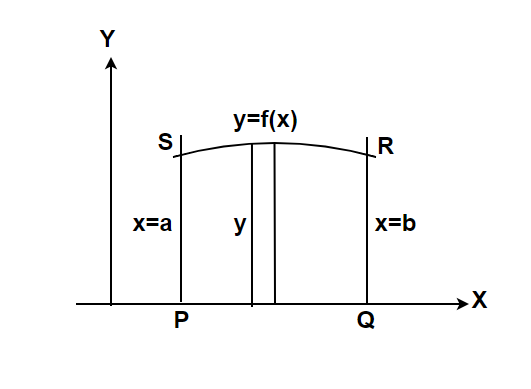
因此，由x = a和x = b之间的曲线f(x)界定的ABCD区域的面积为：

$\int^{b}_{a}f(x)dx$

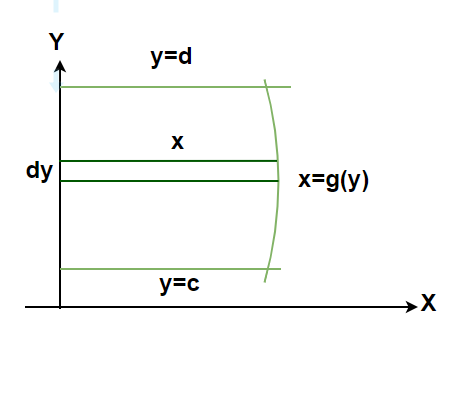
类似地，如果我们有一个函数g(y)来限制y = a和y = b之间的区域。它的面积由

$\int^{b}_{a}g(y)dy$

Note: If the function is considered to be in the negative interval, then the area comes out to be negative. But we don’t worry about the negative area, it is only numerical value of the area that is taken into consideration. 

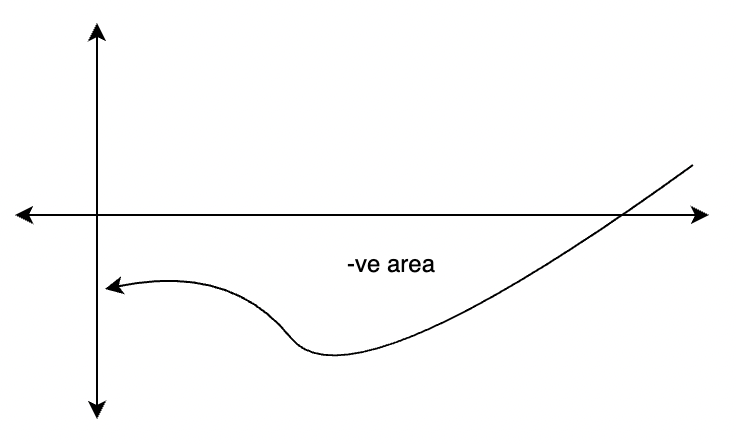
Now it might also happen that some part of the area is negative and some is positive as seen in the figure below. 

The area A1 is negative and A2 is positive. In such cases, 

A = |A₁| + A2

让我们看看与此概念有关的一些问题

样本问题

问题1：通过f(x)= e ^x找出x = 0到x = 5之间的区域。

解决方案：

The area enclosed by the line = $\int^{5}_{3}x$

                                                   = $[e^x]^5_3$

                                                   = $e^5 - e^3$

问题2：通过y = x线找到x = 3和x = 5之间的区域。

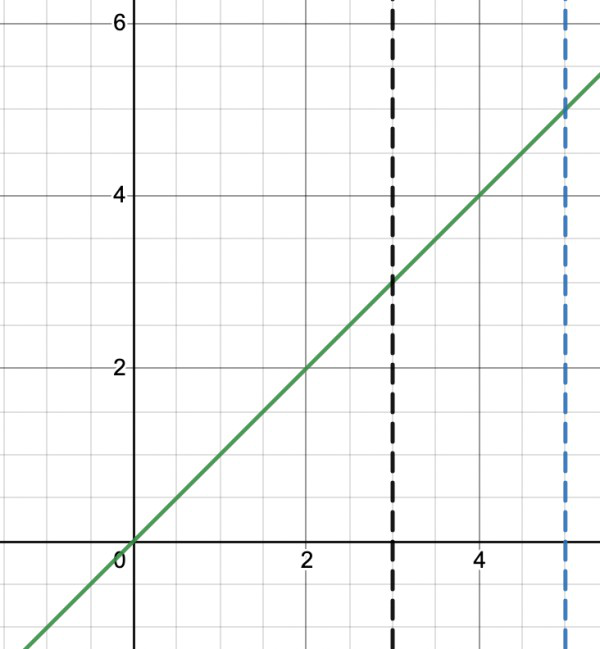
解决方案：

The area enclosed by the line = $\int^{5}_{3}x$

                                                   = $[\frac{x^2}{2}]^5_3$

                                                   = $\frac{5^2 - 3^2}{2}$

                                                    = $\frac{16}{2}$

                                                   = 8

问题3：求出圆x ² + y ² = b ²包围的面积。

解决方案：

Figure

The area enclosed by the circle = 4(Area enclosed by the curve between 0 to b) 

                                                       = 4(Area enclosed in the region POQP) 

                                                       = $4 \int^{b}_{a}ydx$

                                                       = $4 \int^{b}_{a}\sqrt{b^2 - x^2}dx$

                                                       = $4[\frac{x}{2}\sqrt{b^2 - x^2} + \frac{b^2}{2}sin^{-1}(\frac{x}{b})]$

                                                       = $4[\frac{b}{2}\times 0 + \frac{b^2}{2}sin^{-1}(\frac{b}{b})]$

                                                       = $4[ \frac{b^2}{2}\times\frac{\pi}{2}]$

                                                       = $\pi b^2$

问题4：找到曲线f(x)= x ^3的-2和2之间的面积。

解决方案：

Given the curve, f(x) = x³. From the graph we can see that half of it’s area is negative, so it will be added with it’s numerical value. 

Figure

A = $\int_{-2}^0 f(x)dx + \int^2_0 f(x)dx$

   = $\int_{-2}^{0} x^3dx + \int^{2}_{0}x^3dx$

   = $|\int_{-2}^{0} x^3dx| + \int^{2}_{0}x^3dx$

   = $|[\frac{x^4}{4}]^{0}_{-2}| + [\frac{x^4}{4}]^{2}_{0}$

   = $|-\frac{16}{2}| + \frac{16}{2}$

   = $\frac{16}{2} + \frac{16}{2}$

   = 16

问题5：找出曲线f(x)= x ²和y = 9之间所包围的区域的面积。

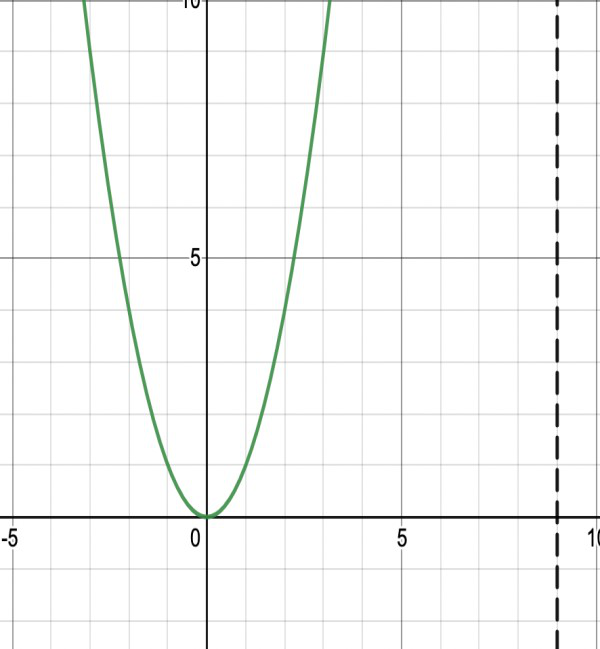
解决方案：

We can see in the graph that the area of the region enclosed between them will be given by the difference in the area under f(x) and the line y =9. 

Figure 

The line intersects f(x) at x = 3 and x = -3. So, a = -3 and b = 3. 

Let A₁ be the area under the f(x) 

A₁ = $\int^{3}_{-3}f(x)dx$

      = $\int^{3}_{-3}x^2dx$

      = $[\frac{x^3}{3}]^{3}_{-3}dx$

      = $[\frac{27}{3} - \frac{-27}{3}]dx$

      = 18 

Let A₂ be the area under the line y = 9.

A₂ = 9 (6) = 54

A = A_{2 –} A₁

   = 54 – 18 

   = 36