几何起源于多种文明。几乎每个主要文明都已在其黄金时期研究和使用几何学。埃及和印度文明更加关注使用几何作为工具。欧几里得来了,改变了人们过去在几何学中思考的方式。他没有将其用作工具,而是将几何学视为他所生活的世界的抽象模型。
欧几里得几何
根据欧几里得
固体具有形状,大小,位置,可以从一个地方移动到另一个地方。它的边界称为曲面。它们将空间的一部分与另一部分隔开,据说没有厚度。表面的边界是弯曲的或直线的。这些线以点结尾。”
从这些定义开始,他假定了某些尚待证明的特性。他们被称为“显而易见的普遍真理”。他将它们分为两种类型,
- 假设:它们是特定于几何的假设。
- 公理:它们通常是常见的概念。
让我们快速看一下Euclid的公理。
- 等同于同一事物的事物彼此相等。
- 如果将相等加到相等,则整体相等。
- 如果从等于中减去等于,则余数等于。
- 彼此重合的事物彼此相等。
- 整体大于部分。
- 相同事物的两倍的事物彼此相等。
- 一半相同的事物彼此相等。
这些公理大多数是不言自明的。
欧几里得的假设
假设1:可以从任何点到任何其他点画一条直线。
假设2:给定两个不同的点,有一条独特的线穿过它们。
假设2:可以无限期地产生终止线。
假设3:可以画出任何圆心和任何半径的圆。
假设4:所有直角彼此相等。
假设5:如果一条直线落在两条直线上,使得同一侧的内角合计小于两个直角,则两条直线(如果无限期产生)在那一侧的相交角相交小于两个直角。
除了这些假设5,其中大多数假设都非常简单明了。让我们详细了解其解释和等效版本。
第五种假设的等效版本
第五个假设指出,
“如果一条直线落在两条直线上,使得同一侧的内角合计小于两个直角,则这两条直线(如果无限期产生)会在该角度之和较小的那一侧相交超过两个直角。”
该假设在数学史上占有重要地位。
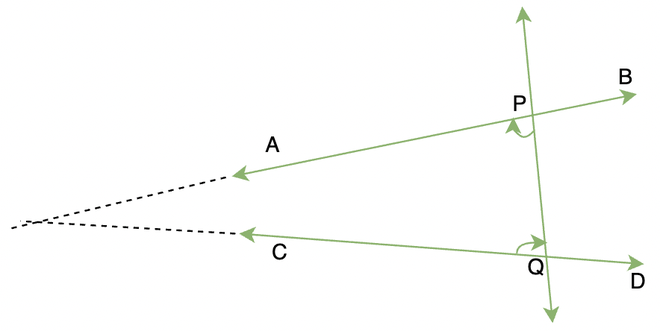
从图中可以看出,两个内角均小于90°。因此,它们的总和必须小于180°。因此,当它们延伸时,它们在特定点相交。如果内角为90°或大于90°,则不会发生这种情况
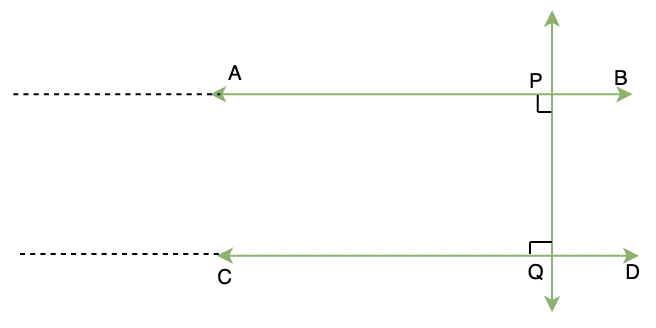
该假设有多个等效版本,其中一个称为Playfair的Axiom 。
Playfair的公理
“For every line “l” and for every point “P” lying not on “l”, there exists a unique line “m” passing through P and parallel to “l”.
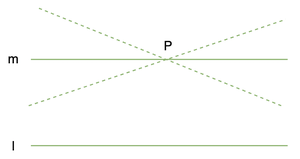
从上图可以看出,有多条线穿过P,但是只有一条线穿过P,且平行于“ l”。
我们还可以用另一种形式重写此语句,
“Two distinct intersecting lines cannot be parallel to the same line.”
注意(事实):欧几里德不需要第五种假设来证明他的第一个28个定理。包括欧几里得在内的许多数学家都坚信,第五种假设实际上是一个无法证明的定理。进行了几次尝试,但没有人能够证明第五种假设。
样本问题
让我们看一些关于这些假设和公理的例子,
问题1:苛刻的薪水等于拉姆的薪水。由于Covid-19衰退,Harsh和Ram的薪水减半。 Ram的最终薪金将仍然等于Harsh。这是根据
- 1日公理。
- 第7公理。
- 第6公理。
- 第二公理。
回答:
7th Axiom states that,
“Things which are halves of the same things are equal to one another.”
This axiom can be applied here directly. Thus, the answer is (2).
问题2:固体边界为:
- 线数
- 点数
- 表面
- 曲线图
回答:
According to Euclid’s definition,
“A solid has shape, size, position, and can be moved from one place to another. Its boundaries are called surfaces. They separate one part of the space from another, and are said to have no thickness. The boundaries of the surfaces are curves or straight lines. These lines end in points.”
This definition states that boundaries of solids are called surfaces.
Thus answer is (3).
问题3:在下图中,线段的PS = RQ。证明PR = SQ。

回答:
Given that PS = RQ
From the figure we can say,
PS = PR + RS
QR = RS + SQ
From the Euclid axiom number 3 stated above, we know that
“If equals are subtracted from equals, the remainders are also equal.”
Since, PS = QR
Subtracting RS from both sides.
⇒ PS – RS = QR – RS
⇒ PR = QS
问题4:已知a + b = 18,a = c。证明c + b = 18。
回答:
It is known by the Euclid axioms studied above,
“If equals are added to equals, the wholes are equal.”
The given equations are,
a + b = 18 ….. (1),
And a = c….. (2)
From the equations (1) and (2), we get.
a + b = c + b ….(3)
Now from equation (1) and equation (3).
We can conclude that
b + c = 18.
问题5:已知a + b = 11,然后a + b + c = 11 + c。证明这一说法的欧几里得公理是,
- 1日公理。
- 第三公理
- 第4公理
- 第二公理
回答:
The second axiom of Euclid stated above states that,
“If equals are added to equals, the wholes are equal.”
This axiom can be applied here. So, the answer is (4).