图形化的运动方程

英国著名科学家伊萨克·牛顿(Issac Newton)得出了三个运动方程，它们描述了物体运动的最基本概念。这些方程式在一个，两个和三个维度上控制对象的运动。这些方程式很容易用于计算对象在不同时间的位置，速度或加速度的值或表达式。首先让我们了解运动的基本概念以及与之相关的不同术语。

什么是运动?

运动可以描述为对象位置相对于时间的变化。可以使用参考点测量位置，并计算对象到参考点的距离。可以使用速度表来计算时间，速度表将确定改变位置所花费的时间。有很多伟大的科学家致力于研究运动等方程式或理论，例如伽利略·伽利莱(Galileo Galilei)和以撒(Isaac Newton)。

汽车在一定时间间隔内的运动

在讨论运动方程之前，让我们首先修改与物体运动有关的基本术语。可以使用以下四个不同的术语来描述对象的运动：

距离

物体位置(在特定时间段内)的总变化的实际量度称为距离。

距离(d)是标量，因此仅给出幅度。  

e.g. Consider the figure below, there is a car moving from position A to position B. The speedometer in the car will show the distance traveled from point A to point B.

Distance traveled by car

移位

物体位置(在特定时间间隔内)的净变化的最短度量称为位移。

位移是矢量，因此，它给出了幅度和方向。

e.g. Consider the figure below, there is a car moving from position A to position B. The displacement is equal to the distance covered in a certain direction of the motion.

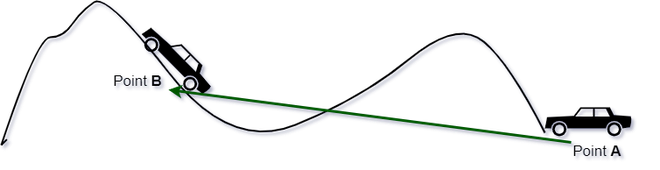
Displacement of the car

速度

物体的速度是确定物体移动或改变其位置的速度或速度的量度。

速度等于对象所覆盖距离中的时间速率变化。

e.g. Suppose a fan which is running very fast, but it is fast with respect to your stationary state, if you will also spin at the same speed as the fan you will see it is not even moving. 

Mathematically, the speed of an object is given as:

$\text{Speed\, (s)}=\dfrac{\text{Distance (d)}}{\text{Time (t)}}$

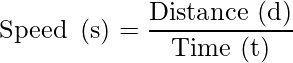
Since, both time and distance are scalar quantities therefore, speed is also a scalar quantity. 

The SI unit of speed is m/s.

速度

物体的位移相对于时间的变化率或位置的变化率称为速度。

在图形上，它是位移函数的斜率。它是一个向量，因此给出了幅度和方向。

Mathematically, the velocity is defined as:

$\text{Velocity (v)}=\dfrac{\text{Displacement (D)}}{\text{Time (t)}}$

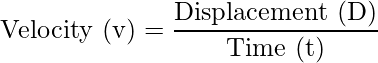
The SI unit of velocity is same as the speed that is, m/s. The difference between the speed and velocity of an object is that, the speed is a scalar quantity while the velocity is a vector quantity.

加速

将物体每单位时间的速度变化或速度函数相对于时间的变化率定义为加速度。

在图形上，它是速度函数的斜率。加速度是矢量，因此可以给出幅度和方向。

Mathematically, the acceleration is defined as:

$\text{Acceleration (a)}=\dfrac{\text{Velocity (v)}}{\text{Time (t)}}$

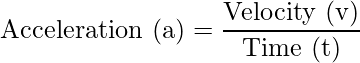
运动方程是什么?

根据物理系统的运动作为时间的函数来解释物理系统的性质和行为的方程式称为运动方程式。可以使用三个运动方程来计算运动分量，例如对象的距离，位移，速度(初始和最终)，时间(t)和加速度(a)。以下是三个运动方程：

• First Equation of Motion:             v = u + at
• Second Equation of Motion:        s = ut + 1/2(at²)
• Third Equation of Motion:          v² = u² – 2as

where, v and u are the initial and the final velocities, a is the acceleration, t is the time taken and s is the displacement of an object.

运动方程的推导

根据在不同解决方案中使用不同组件的目的，有三种不同的方法可以得出这些方程式：

1. Derivation of the equations of motion algebraically, using the definition and different formulae of the components of the motion.
2. Derivation of the equations of motion graphically, using the graphical representation for distance, velocity and acceleration of an object.
3. Derivation of the equations of motion using integral method.

这里，在本文中，三个运动方程的推导以图形方式讨论：

以图形方式推导第一运动方程：

第一个方程将速度与物体的加速度和时间相关联。因此，该方程在不给出位移的情况下适用，因此也称为速度-时间关系。

考虑如下的速度-时间图，物体的速度在时间t内从A到C以均匀的速率变化。从A到x轴的距离是最终速度，OC是总时间t。

从B到OC绘制一条垂直线，从A到D绘制一条平行线，从B到OE绘制另一条垂直线(由虚线表示)。

该图显示，对象具有可变的速度，当坡度为正时，对象的速度从u到v呈正方向增加。

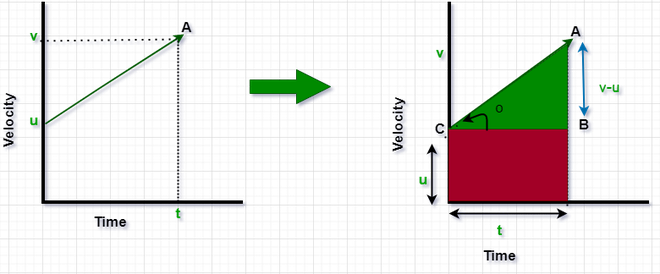
速度-时间图

现在，我们将使用该运动图来计算加速度。加速度是vt图中角度的切线。

$Acceleration = tan ∅ = \frac{AB}{CB} \\~\\ a = \frac{( v - u )}{t} \\~\\ rearrange\ above\ equation \\~\\ v = u + at\\$

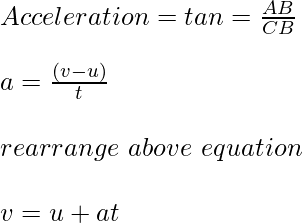
以图形方式推导第二运动方程：

vt下方的图显示了对象的速度和时间关系，初始速度为um / s，最终速度为vm / s。众所周知，vt图的面积给出了对象的位移，因此我们将计算图的面积并找出位移方程。我们在先前的推导中使用了相同的图形，但是这里的方法将有所不同，之前我们使用斜率来计算加速度，但是现在我们将使用面积。

vt运动图

对象的位移(d)=三角形ABC的面积+矩形BCOT的面积

在这里，三角形ABC的面积= 1/2×基数×高度

= 1/2 ×t×(vu)

矩形BCOT的面积=长×宽

= u×t

因此，物体的d位移d = 1/2 ×t×(vu)+ u×t……(1)

同样，从第一个运动方程式中，v – u = at

将at替换为方程式(1)中的vu，

d = 1/2 ×t×(at)+ u×t

d = ut +(1/2)在²

以图形方式推导第三运动方程：

我们有不同的计算面积的方式，例如将任何形式分成小块，然后将将问题转换为我们所熟知的形状的面积加起来。在该推导中，对矩形的面积进行转换，并使用矩形的面积计算位移。如果没有给出时间，则使用该方程式。

原始图到突出显示的图

在这里，P是中心点，所以物体的速度是(v + u)/ 2。

因此，对象的位移(d)=三角形ABC的面积+矩形ACTO的面积=矩形OPQT的面积

对象的位移，d =长度×宽度

= t×(v + u)/ 2……(2)

同样，根据第一个运动方程，v – u = at或t =(v – u)/ a

因此，等式(2)变为：

d =(v – u)/ a×(v + u)/ 2

v ² = u ² – 2ad

样本问题

问题1：粒子的速度-时间图如下所示，找到粒子在40分钟内覆盖的距离。

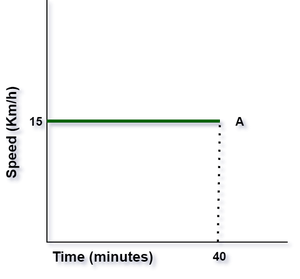
速度-时间图

解决方案：

Calculating distance using the area under the speed-time graph.

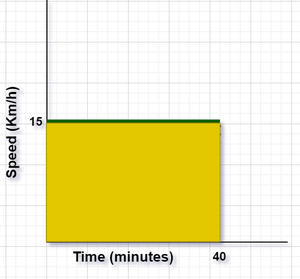
Area under the given curve.

Distance covered by the particle in 40 min = Area under the given curve.

The shape formed under the curve is of a rectangle therefore, the area under the curve = length × width.

Here, the length of the shape is equivalent to the time taken i.e. 40 min or 40 min × 60 s/1 min = 2400 s.

Similarly, the width of the shape is equivalent to the speed i.e. 15 km/h or 15 km/h × 1 h/3600 s × 1000 m/1 km = 4.17 m/s.

Therefore, the area under the curve or the distance covered in 40 min is given by:

⇒ 2400 s × 4.17 m/s = 10000 m or 10 km.

Hence, the distance covered by the particle in 40 min is equal to 10 km.

问题2：对于以下情况，该图计算20分钟到40分钟之间粒子所覆盖的距离。

速度-时间图

解决方案：

Distance covered by the particle from time t = 20 min to 40 min is equal to the Area under the given curve between the given time range.

Highlighted Area of Speed-time Plot

The shape formed under the curve is of a rectangle therefore, the area under the curve = length × width.

Here, the length of the shape is equivalent to the time taken i.e. (40 – 20) min or 20 min × 60 s/1 min = 1200 s.

Similarly, the width of the shape is equivalent to the speed i.e. 15 km/h or 15 km/h × 1 h/3600 s × 1000 m/1 km = 4.17 m/s.

Therefore, the area under the curve or the distance covered from time t = 20 min to 40 min is given by:

⇒ 1200 s × 4.17 m/s = 5004 m or 5.004 km.

Hence, the distance covered by the particle from time t = 20 min to 40 min is equal to 5.004 km.

问题3：找到在时间间隔t = 0 s和t = 4 s内粒子所覆盖的距离，其速度-时间图如下所示：

速度-时间图

解决方案：

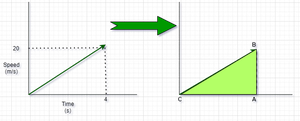
Speed-Time Graph

Distance covered by the particle in t = 0 s and t = 4 s is equal to area under the curve.

The shape formed under the curve is of a triangle therefore, the area under the curve = 1/2 × base × height.

Here, the base of the shape is equivalent to the time taken i.e. 4 s.

Similarly, the height of the shape is equivalent to the speed i.e. 20 m/s.

Therefore, the area under the curve or the distance covered in t = 0 s and t = 4 s is given by:

⇒ 1/2 × 4 s × 20 m/s = 40 m.

Hence, the distance covered by the particle in t = 0 s and t = 4 s is equal 40 m.

问题4：下图显示了三个对象A，B和C的距离-时间图。确定：

(a)哪个物体以更快的速度运动?

(b)哪个物体以较低的速度运动?

距离-时间图

解决方案：

The given graph is distance time graph and the slope gives us the value of speed i.e. more the slope more is speed. 

As it is observed from the given graph, A has maximum slope, so it is moving with greater speed and C has the least slope, so it is moving with the slowest speed.

问题5：借助给定的距离-时间运动图计算粒子的速度。

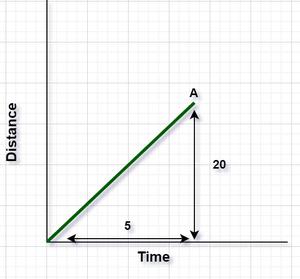
距离-时间图

解决方案：

Here, the speed of the particle is equal to the slope of the graph.

And the slope of the distance graph is equal to the speed of the particle.

Therefore, the formula to calculate the speed of the particle is:

Speed = Distance / Time

          = 20 m / 5 s

         = 4 m/s.

Hence, the speed of the particle is equal to 4 m/s.