Permutation– :设 G 是一个非空集,然后一对一映射到自身,即
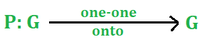
称为置换。
- 有限集 G 中元素的个数称为排列度。
- 设 G 有 n 个元素,则 P n 被称为 n 次所有排列的集合。
- P n 也称为 n 次对称群。
- P n也由 S n表示。
- P n或 S n 中的元素数为
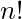
例子:
Case1: Let G={ 1 } element then permutation are Sn or Pn = ![]()
Case 2: Let G= { 1, 2 } elements then permutations are 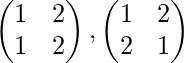
Case 3: Let G={ 1, 2, 3 } elements then permutation are 3!=6. These are,
读取排列符号
假设一个置换是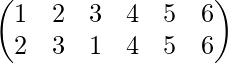
- 首先,我们看到在一个小括号里写着两行,这两行有数字。最小的数是 1,最大的数是 6。
- 从第一行的左边开始,我们读取的图像1为2,1的图像为2,2的图像为3,3的图像为1,4的图像为4 (Self image= same=identity) ,5 的图像是 6,6 的图像是 5。
- 上面的东西也可以理解为:从第一行左边开始1到2,2到3,3到3,4到4,5到6,6到5。
长度为 2 的循环称为置换。
例子:
1) ![]()
Length is 2, so it is a transposition.
2) ![]()
Length is three, so it is not a transposition.
置换的乘法
Problem: If 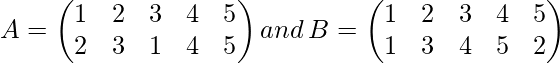
Find the product of permutation A.B and B.A
Solution: 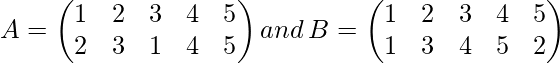

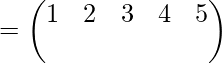
Here we can see that in first bracket 1 goes to 2 i.e. image of 1 is 2, and in second row 2 goes to 3 i.e. image of 2 is 3.
Hence, we will write 3 under 1 in the bracket shown below,
Do above step with all elements of first row, answer will be

Similarly,
