质量中心
质心是力学中非常重要的一部分。它允许我们通过将大物体视为点对象来对它们进行计算。这些简化随后成为不同力学领域发展的基础。例如,旋转力学、引力力学等。质心直观地表示我们可以从下方支撑物体的点。例如,可以从中间点支撑一根棍子,使其不会掉落。同样,每个对象都有一个相似的点。这称为质心。
质量中心
如果假设物体的质量位于某一特定点,则可以简化很多问题。如果选择了正确的位置,则力方程和运动方程的行为方式与它们在质量分散时的行为方式相同。这个特殊的位置被称为质量中心。
它的位置是相对于要计算其质心的对象或对象系统定义的。通常对于统一的形状,它是它们的质心。让我们从简单的形状开始,看看它们的质心在哪里。考虑下图中给出的形状。很容易猜出以下形状的质心。对于他们中的大多数人来说,质心位于他们的质心。
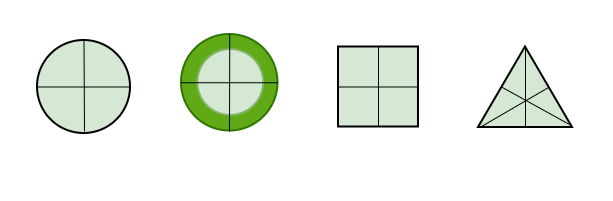
请注意,对于环来说,它的质心位于环内,这意味着物体的质心必须位于物体本身。
寻找质心
现在,很明显,均匀对称的物体的质心位于质心。但对于不对称和不均匀的物体,答案并不那么简单。这种物体的质心可以在任何地方。计算复杂物体的质心。取每个身体质量的位置的加权平均值。
Let’s say there is a body consisting of a set of masses “mi“, each at position ri, the location of the Centre of mass rcm is given by the formula below.
Mrcm = m1r1 + m2r2 + ….
⇒ rcm = ![]()
In this case, M = , which is the total mass of the body.
上述技术使用矢量算术。为了避免矢量算术,我们可以分别沿 x 轴和 y 轴找出物体的质心。这种情况的公式如下:
x厘米= ![]()
y厘米= ![]()
重心
通常,假设重力是作用在物体上的均匀力。重心是假设重力作用在身体上的点。因此,重心与质心位于同一位置。在物理学文献中,重心和质心这两个术语可以互换使用。他们的意思是一样的。
重要的质量中心
有些系统在现实生活中比其他系统更频繁地出现。在计算此类系统的质心时,传统方法需要时间。在解决与质心有关的问题时,应牢记某些质心公式。这些公式有助于简化计算。
1.两点质量系统
In such a system, COM lies closer to the heavier mass.
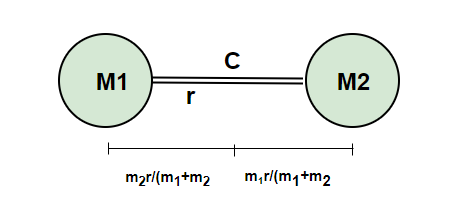
m1r1 = m2r2
Distance of COM from mass m1 = ![]()
Distance of COM from mass m2 = ![]()
2.等边三角形
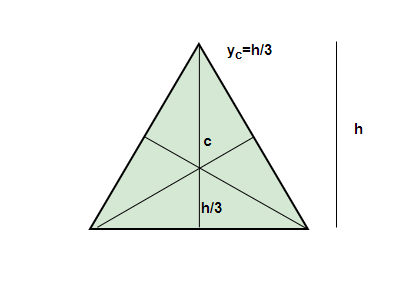
The Centre of mass lies at the centroid, which is at the height of ![]()
3.半圆盘
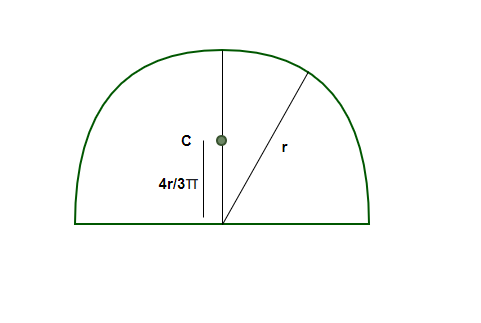
yc = ![]()
4.半圆环
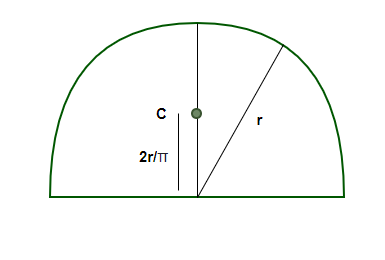
yc = ![]()
让我们看一些示例问题
示例问题
问题 1:两个点质量 m 1 = 5Kg 和 m 2 = 2Kg 分别位于 x = 2 m 和 x = 6 m。找到质心。
解决方案:
The formula for the Centre of mass is given by,
xcm = ![]()
m1 = 5Kg, m2 = 2Kg and x = 2 m and x = 6 m.
M = m1 + m2
⇒ M = 5 + 2 = 7
xcm = ![]()
⇒ xcm = ![]()
⇒ xcm =
⇒ xcm = ![]()
问题 2:两个点质量 m 1 = 5Kg 和 m 2 = 2Kg 分别位于 y = 10m 和 y = -5 m。找到质心。
解决方案:
The formula for the Centre of mass is given by,
ycm = ![]()
m1 = 5Kg, m2 = 2Kg and y = 10m and y = -5 m.
M = m1 + m2
⇒ M = 5 + 2 = 7
ycm = ![]()
⇒ ycm = ![]()
⇒ ycm = ![]()
⇒ ycm = ![]()
问题 3:两个点质量 m 1 = 1Kg 和 m 2 = 2Kg 分别位于向量 a = 6i + 4j 和向量 b = -5i + 2j。找到质心。
解决方案:
The formula for the Centre of mass in the vector notation is given by,
rcm = ![]()
m1 = 1Kg, m2 = 2Kg and a = 6i + 4j, b = -5i + 2j
M = m1 + m2
⇒ M = 1 + 2 = 3
rcm = ![]()
⇒rcm = ![]()
⇒ rcm = ![]()
⇒ rcm = ![]()
⇒ rcm = ![]()
问题 4:两个点质量 m 1 = 4Kg 和 m 2 = 2Kg 分别位于向量 a = i + j 和向量 b = -i + j。找到质心。
解决方案:
The formula for the Centre of mass in the vector notation is given by,
rcm = ![]()
m1 = 4Kg, m2 = 2Kg and a = i + j, b = -i + j
M = m1 + m2
⇒ M = 4 + 2 = 6
rcm = ![]()
⇒rcm = ![]()
⇒ rcm = ![]()
⇒ rcm = ![]()
⇒ rcm = ![]()
问题 5:从质量为 M、半径为 R 的较大圆盘中移除一个半径为 R/2 的圆盘。求质心。
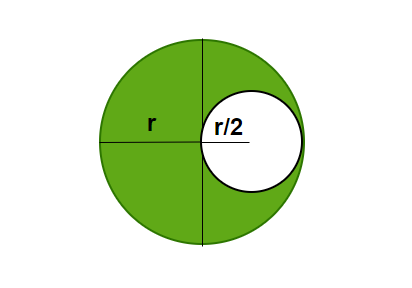
解决方案:
Since the density of the disk is uniform, the weight is uniformly distributed over all the area.
Mass “m” of the removed disk = 
Figure
The figure shows the center of masses of the remaining portion and the removed portion. Notice that if both of these are taken together, the center of mass should lie at the Centre. Let the distance of the center of mass of the remaining portion be “x”.
问题 6:半径为 R/4 的圆盘从质量为 M、半径为 R 的较大圆盘中移除,方法与上图相同。找到质心。
解决方案:
Since the density of the disk is uniform, the weight is uniformly distributed over all the area.
Mass “m” of the removed disk = 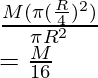
The figure shows the center of masses of the remaining portion and the removed portion. Notice that if both of these are taken together, the center of mass should lie at the Centre. Let the distance of the center of mass of the remaining portion be “x”.