功能定理

“工作”这个概念在日常言语中很常见，我们理解它是指完成某事的行为。例如，您目前正在通过阅读本文提高对物理学的理解！然而，物理学可能不同意这一点。功能定理解释了为什么这种无功物理学存在！

当操作力使粒子发生位移时，就称做功。没有搬迁就没有工作完成。如果你站的时间长了，你可能会感到疲倦，但根据物理学，你没有做任何劳动。

什么是工作？

The work done on a system by a constant force is the product of the component of the force in the direction of motion times the distance through which the force acts. 

一维运动中所做的功可以用方程形式表示为

W = Fdcosθ

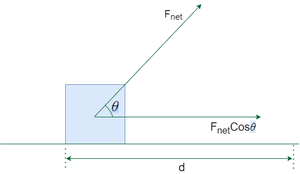
其中 W 是功，F 是系统力的大小，d 是系统位移的大小，θ 是力矢量 F 和位移矢量 d 之间的角度。

完成的工作可以是正数或负数，甚至为零。

• 正功：当一个力沿其方向移动物体时，所做的功被认为是正的。示例：球落向地球的运动，在此测量球的位移，是此类工作的一个示例。球沿重力方向移动。
• 负功：当力和位移方向相反时，假定功为负。示例：例如向上抛球时，位移会向上；然而，由于摩擦力将向下。地球的重力将向下。
• 零功：如果力的方向和位移相互垂直，则力对物体所做的总功为零。例如：当我们用力推墙时，我们施加在墙上的力是无效的，因为墙的位移等于 d = 0。

什么是能量？

工作能力被定义为能量。一些类型的能量包括势能、动能、热能、电能、化学能和核能。运动与所有能源有关。例如，当一个物体在运动时，它具有动能。当弓或弹簧等张紧的物体静止时，它具有产生运动的能力；它的配置具有势能。核能也是势能，因为它来自原子核中亚原子粒子的排列。

能量有很多种形式，这里我们主要关注动能。

动能：

• 动能是物体或粒子由于其运动而具有的能量类型。
• 当通过施加合力对物体做功时，该物体会加速并因此获得动能。
• 动能是运动物体或粒子的属性，由其质量和运动决定。
• 如果沿着一条路径从一个地方到另一个地方运动，那么物体所拥有的动能就是平移动能，如果绕轴旋转，那么物体就拥有旋转动能。

1. 转化动能：
   • 物体的平移动能等于其质量 m 和速度 v 乘积的一半，或$\frac{1}{2}mv^2$
   • 该公式仅适用于低速到中速运动的粒子；对于真正高速的粒子，它产生的值太小。当一个物体接近光速（每秒 3 × 10 ⁸米，或每秒 186,000 英里）时，它的质量会增加，需要应用相对论。
   • 粒子质量超过其静止质量的增加乘以光速的平方等于相对论动能。

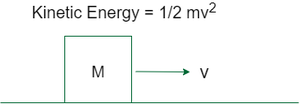
质量为 M 并以速度 V 移动的物体

• 旋转动能：转动体的转动惯量 I 与质量有关，而角速度 (omega) 与线性或平移速度有关。因此，旋转动能等于转动惯量和角速度平方乘积的一半，或者$\frac{1}{2}Iω^2$

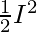
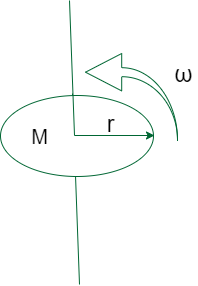
质量为 M、半径为 r 的圆盘沿其轴以角速度 ω 旋转

功能定理

功能定理的陈述是，

Work-energy theorem states that the work done by the sum of all forces acting on a particle equals the change in the kinetic energy of the particle (or) The net work done on a system is equal to the change in Kinetic energy.

功能定理

合力对粒子所做的功 W 等于粒子动能 KE 的变化。

$W=\triangle KE$

$W= \frac{1}{2}mv_f-\frac{1}{2}mv_i$

其中 v _i和 v _f是粒子在施加力之前和之后的速度，m 是粒子的质量。

恒力做功的推导

让我们考虑合力 F 在大小和方向上都是恒定的并且平行于粒子的速度的情况。粒子以恒定加速度 a 沿直线运动。

恒力所做的功

The relationship between the net force and the acceleration is given by the equation F = ma (Newton’s second law), and the particle’s displacement d, can be determined from the equation, 

 $v_f^2=v_i^2+2ad$                              (from the equation $v^2=u^2+2as$  where v=final velocity, u=initial velocity and s = displacement)

We get, 

$d = \frac{v_f^2-v_i^2}{2a}$

The work of the net force is calculated as the product of its magnitude and the particle’s displacement (W=Fd). 

Substituting the above equations yields:

$W=F(\frac{v_f^2-v_i^2}{2a})$

$W=(ma)(\frac{v_f^2-v_i^2}{2a})$

$W=m(\frac{v_f^2-v_i^2}{2})$

$W=\frac{mv_f^2}{2}-\frac{mv_i^2}{2}$

W=$KE_f-KE_i$  

$\triangle KE$

Therefore, the net work done = change in Kinetic Energy

变力做功的推导

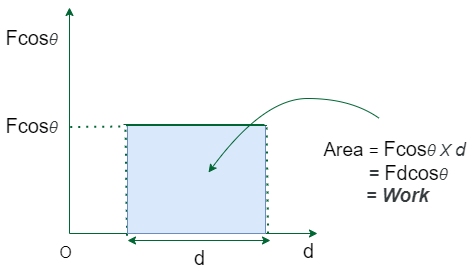
可变力是我们每天遇到的最常见的力。变力的功能量公式计算起来有点复杂。考虑一个在一个轴上具有位移而在另一个轴上具有可变力的图形。 x 轴表示位移，而 y 轴表示该图中的力。沿着 x 轴，将图形的区域划分为任意小宽度的矩形。

变力做的功

We can suppose that the force applied is constant for an arbitrarily tiny displacement $\triangle$x. As a result, we have;

⇒ $\triangle W=F(x) \triangle x$

As a definite integral of force over the net displacement, we can now define work as:

⇒ $W=\int_{x_i}^{x_f} F(x)dx$

Because kinetic energy is equal to $K=\frac{1}{2}mv^2$,

The change in kinetic energy over time can be described as follows:

⇒ $\frac{d}{dt}(K)=\frac{d}{dt}(\frac{1}{2}mv^2)$

⇒ $\frac{dK}{dt}=m\frac{dv}{dt}$

Acceleration, according to Newton’s second law of motion, is the change in velocity of an object with respect to time. As a result, the above equation can be rewritten as,

⇒ $\frac{dK}{dt}=mav$

We can express the previous equation as, because F= ma and velocity is the rate of change of displacement over time.

⇒ $\frac{dK}{dt}=F \frac{dx}{dt}$

When both sides of the equation’s time derivatives are removed, we get

⇒ dK=Fdx

By integrating the previous equation along the x-axis, we get,

⇒ $\int_{x_i}^{x_f}dK=\int_{x_i}^{x_f}Fxdx$

⇒ $K_f-K_i=\int_{x_i}^{x_f}Fxdx$

or we can write, $\triangle K=\int_{x_i}^{x_f}Fxdx$

Hence we get, $\triangle K=W$

The above equation is the proof of the work-energy theorem for the variable force.

示例问题

问题 1：一个 2 kg 的球从 10 m 的高度落下。计算从球被释放到落地的那一刻对球所做的功。假设空气阻力可以忽略不计。

解决方案：

Given, 

Mass of the ball, m=2 kg.

Initial height of the ball, h_i =10 m.

Final height of the ball, h_f =0 m.

We must calculate the amount of work done on the ball as it hits the ground.

The ball is dropping freely here, conserving energy. We know that the difference in kinetic energy equals to the work done.

Because the ball is in a motionless condition when it is dropped, it has no kinetic energy at first.

All the ball’s potential energy is transformed to kinetic energy when the ball touches the ground.

So, Potential Energy of Ball at height h_i is,

E_p = mgh

 $E_p=2\times 9.8 \times 10=196 J$

Now, The ball had 196 J of potential energy when it was released and 0 J of kinetic energy. 

When the ball hit the ground, it had 0 J of potential energy and 196 J of kinetic energy. 

Therefore, Initial Kinetic Energy KE_i=0 J and KE_f=196 J.

By work-energy theorem:

W_net = ΔKE

KE_f − KE_i = 196−0 

              = 196 J

Therefore, The net work done on the ball is 196 J.

问题 2：一辆质量为 500 kg 的汽车以 16 m/s 的速度行驶，在某个点应用汽车的刹车。汽车的制动器提供 4000 N 的摩擦力。确定汽车的制动距离。

解决方案：

Given, 

Mass of the car, m = 500 kg

Speed of the car, v = 16 m/s

Frictional force of brakes, $\vec{F}$ = 4000 N

Applying the work-energy theorem as:

The change in the kinetic energy is equal to the work done by the frictional force of the car’s brakes.

Therefore, Initial Kinetic energy of car at the moment brakes were applied is,

$KE_i = \frac{1}{2}mv^2$

$KE_i = \frac{1}{2}\times500\times (16)^2$ ⇒ 64 kJ.

As the car is at rest, Final Kinetic energy =0, therefore $\triangle KE$ = 64 kJ.                                                                                     ……(1)

Consider the displacement of car is ΔX. Since the direction of the applied force and the displacement are in opposite directions, θ=180°. 

Then the work done is given by:

$W=F\triangle x cos\theta$

$W = (4000)(\triangle x)(\cos{180\degree})$

$W = -4000\triangle x$                                                                                                                                                             ……(2)

By work-energy theorem, The change in kinetic energy is equal to the work done.

$64000 = -4000 \triangle x$

$\triangle x = 16m$

Therefore, The stopping distance of car is 16 m.

问题3：下雨天，司机看到红灯就踩刹车。实测汽车在刹车点后有 100 m 的防滑痕迹停止时，摩擦系数估计为 0.45。司机踩刹车时汽车的速度是多少？

解决方案：

Given, 

Displacement of car, d = 100m

Coefficient of Friction, μ = 0.45

We know that, force of friction is the cause of the work done on car to slow it to a stop.  We also know that the work is equal to the change in kinetic energy.

−W=ΔKE                                (-ve symbol because Frictional force and the displacement are in opposite direction, cos(180°)=-1)

 $-W=\frac{1}{2}mv_f^2-\frac{1}{2}mv_i^2$

Work is equal to the force times the displacement over which the force acted.

−W=Fd

Therefore, $Fd=\frac{1}{2}mv_f^2-\frac{1}{2}mv_i^2$

The force in this case is the force of friction. so, F_friction = μF_N

Since the car is on a level road the normal force is equal and opposite the force of gravity.

F_N=F_G

The force of gravity is equal to the mass times the acceleration due to gravity, F_G=mg

Therefore, F_friction = μmg

By, work-kinetic energy equation,

$-μmgd=\frac{1}{2}mv_f^2-\frac{1}{2}mv_i^2$

Since the final velocity is 0 we get,

$-μmgd=-\frac{1}{2}mv_i^2$

$μgd=\frac{1}{2}v_i^2$

$(0.45)(9.8\frac{m}{s^2})(100m)=\frac{1}{2}v_i^2$

$v_i^2=882 \frac{m}{s}$

$v_i=29.69m/s$

Therefore, Initial velocity of car is 29.69 m/s.

问题 4：2000 kg 火箭的净推进力为 600 N。火箭在很短的时间内从 50 m/s 的初始速度均匀加速到 80 m/s 的最终速度。假设火箭在燃烧燃料时质量保持不变，并且净力指向运动方向。火箭做了多少净功（KJ）？

解决方案：

Given,

Mass of rocket, m = 2000 kg.

Net propulsion Force, F = 600 N.

Initial Velocity of rocket, v_i = 50 m/s.

Final Velocity of rocket, v_f = 80 m/s.

 Applying Work Energy Theorem, 

W = ΔKE

Initial Kinetic energy of the rocket KE_i is given by:

 $KE_i=\frac{1}{2}mv_i^2$

$KE_i= 0.5(2000kg)(50)^2=2500000J$

Similarly, Final Kinetic energy of rocket KE_f is given by,

 $KE_f=\frac{1}{2}mv_f^2$

$KE_f= 0.5(2000kg)(80)^2=6400000J$

Net Work done = The change in the kinetic energy at the two velocities:

W = ΔKE

6400000−2500000 =3 900000 J

Therefore, Net work done on the rocket is 3900 kJ.

问题 5：一支 100 克的箭从弓上射出，弓的字符串在 50 厘米的距离上对箭施加平均 150 牛的力。箭离开弓的速度是多少？

解决方案：

Given, 

Mass of arrow, m = 100g

Force exerted on the arrow, F = 150N

Displacement of string, d = 50cm

Applying Work-Energy Theorem,

W = ΔKE

$W=\frac{1}{2}mv_f^2-\frac{1}{2}mv_i^2$

Work is equal to the force times the displacement over which the force acted.

W = Fd

Therefore, $Fd=\frac{1}{2}mv_f^2-\frac{1}{2}mv_i^2$

Substituting the values, we get

 $(150N)(0.5m)=\frac{1}{2}(0.01kg)(v_f)2−\frac{1}{2}(0.01kg)(0m/s)^2$      

$v_f^2=15000m/s$

$v_f=122.47m/s$

Therefore, Velocity of arrow as it leaves the bow is 122.47 m/s.

问题6：C罗踢了一个足球，它匀速跑了50m，C罗做了多少功？

解决方案：

Work is the product of a net force over a given distance.

W = F_net d

There must be a net force exerted and a non-zero displacement of the object in order for it to do work.

The displacement is provided, but we must solve for the force.

According to Newton’s Second Law of Motion, the football moves at a constant velocity, which means its acceleration is zero.

$a=\frac{v_f−v_i}{t}$

Since, v_f=v_i, a=0 m/s²

 F = ma 

   = m(0) 

   = 0N

If the force is zero, then the work is also zero. Therefore, Work done, W is 0 J.

问题 7：一个 20 kg 的箱子以 4 m/s 的速度移动。一个 100 N 的净力沿其移动方向不断地施加在盒子上，直到它移动了 25 m。盒子的大概最终速度是多少？

解决方案：

Given, 

Mass of box, m = 20kg

Initial Velocity of box, v_i = 4 m/s

Force acting on the box, F = 100N.

Displacement of the box, d = 25m.

Applying Work-Energy Theorem,

W = ΔKE

 $W=\frac{1}{2}mv_f^2-\frac{1}{2}mv_i^2$

Work done by the block, W = Fd

W = 100 × 25 J 

    = 2500 J

Initial Kinetic Energy, $KE_i=\frac{1}{2}mv_i^2$

$KE_i=\frac{1}{2}(20)(4 m/s)^2$

$KE_i=160 J$

Substituting the value, we get,

 $2500 J=\frac{1}{2}(20)v_f^2-160 J$ 

$v_f^2=532m/s$

 $v_f=23.05m/s$

Therefore, appropriate final velocity is 23.05 m/s.