等边三角形的一边是8厘米，周长的正方形面积是多少。

正方形是由四个相等的边组成的几何图形。它是一个规则的四边形，其中所有的角度都是直角。基本上，它可以被认为是矩形的一种特殊情况，其中所有边的长度都相等。例如，一个方形的庭院。

正方形的性质

• 正方形的四个边相等。
• 正方形的对角线相等。
• 正方形的四个角都相等。
• 正方形的对角线平分它的角。

正方形的周长

正方形的周长称为包围几何图形的边界的总长度。

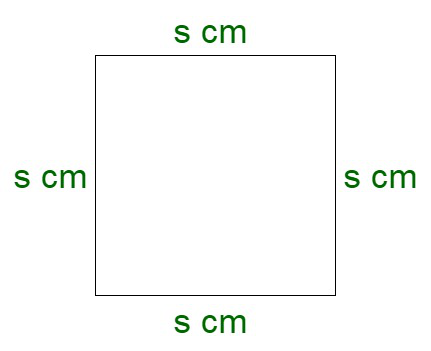
正方形的周长=正方形所有边的总和

让我们假设 s 是正方形的边。

因为，我们知道，正方形的所有边都是相等的。

正方形的周长 = s + s + s + s

正方形的周长 = 4s ..... (I)

正方形的面积

因此，正方形的面积由下式给出，

正方形面积 = s ² ………(II)

等边三角形

等边三角形是所有三个边相等的几何二维图形。所有的边在^拐角处对着一个 60 度的角。因此，三角形所有角的和为180°。

等边三角形的性质

• 等边三角形的三个边相等。
• 所有三个角度都相等，即各 60°。
• 正多边形有三个相等的边。

等边三角形的周长

正方形的周长称为包围几何图形的边界的总长度。

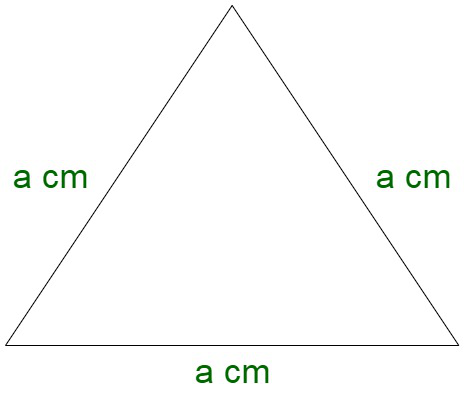
等边三角形的周长=等边三角形所有边的总和

让我们假设 a 是等边三角形的边。

因为，我们知道，等边三角形的所有边都是相等的。

等边三角形的周长 = a + a + a

等边三角形的周长= 3a……。 （三）

等边三角形的一边是8厘米，如果周长相同，正方形的面积是多少。

解决方案：

Since, we know, 

Perimeter of square = 4s  …(By I)

Perimeter of equilateral triangle = 3a … (By III)

As per the question, we get, equating we get, 

Perimeter of square = Perimeter of equilateral triangle

4s = 3a 

On solving, we get, 

=> s = 3/4 a 

Now, we have, side of equilateral triangle = 8 cm 

s = (3/4) * 8 cm 

s = 6 cm 

Therefore, the side of the square = 6 cm

Now,

Area of a square = s² …(By II)

On substituting the values, we get, 

Area of a square = 6²

= 36 sq. cm. 

Therefore, the area of a square = 36 sq. cm. 

示例问题

问题1：假设正方形和三角形的周长相同。如果等边三角形的一边是16厘米。然后求等边三角形和正方形的周长？

解决方案：

Here,

We have one side of the equilateral triangle 16 cm

we are given that the perimeter of the equilateral triangle and square are the same

Perimeter of Equilateral triangle = Side + Side + Side = 3a

Perimeter of Square = Side + Side + Side + Side = 4s

As per the question

Perimeter of Equilateral triangle = Perimeter of Square

3a = 4s

We have a = 16 cm

3 × 16 = 4 × s

48 = 4 × s

s = $\frac{48}{4}$ 

s = 12 cm

Therefore,

Perimeter of Equilateral triangle = 3a

= 3 × 16

= 48 cm

Perimeter of Square = 4s

= 4 × 12

= 48 cm

问题2：假设正方形和三角形的周长相同。如果等边三角形的一侧是12厘米。然后求正方形的面积？

解决方案：

Here,

We have one side of the equilateral triangle 12 cm

we are given that the perimeter of the equilateral triangle and square are the same

Perimeter of Equilateral triangle = Side + Side + Side = 3a

Perimeter of Square = Side + Side + Side + Side = 4s

As per the question

Perimeter of Equilateral triangle = Perimeter of Square

3a = 4s

We have a = 12 cm

3 × 12 = 4 × s

36 = 4 × s

s = $\frac{36}{4}$

s = 9 cm

Side of square is 9 cm.

Now we have to find the area of square

Area of Square = Side × Side

= 9 × 9

= 81 cm²

Area of Square = 81 cm²

问题3：考虑等边三角形和正方形的周长相同，正方形的一边是15厘米。然后找到正方形的周长和等边三角形的边。

解决方案：

Here,

We are given that one side of square = 15 cm

Perimeter of Square = 4 × Side

= 4 × 15

= 60 cm

Perimeter of Equilateral triangle = 3 × side

As per the question perimeter of the Equilateral triangle is equal to the perimeter of the square

Therefore,

Perimeter of Equilateral triangle = 3 × side = 60 cm

60 cm = 3 × Side

Side = $\frac{60}{3}$

Side of Equilateral triangle = 20 cm

问题4：考虑等边三角形和正方形的周长相同。那么求它们的面积之比？

解决方案：

Here,

We have to find the ratio of the area of equilateral triangle and area of square

Assume the side of the triangle be a

Thus the perimeter of triangle = 3a

Assume the side of square be s

Thus the perimeter of square = 4s

As per the question 

Perimeter of Equilateral Triangle = Perimeter of Square

3a = 4s

a = $\frac{4}{3}x$

Then,

Area of Equilateral triangle = $\frac{\sqrt3}{4}a^2=\frac{\sqrt3}{4}\left(\frac{4}{3}s\right)^2=\frac{4}{3\sqrt3}s^2$

Further

Area of square = s²

Now find the ratio of their areas

$\frac{Area\ of\ Triangle}{Area\ of\ Square}\\ =\frac{\frac{4}{3\sqrt3}s^2}{s^2}\\ =\frac{4}{3\sqrt3}\\ =4:3\sqrt3$

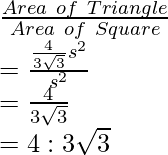
问题5：假设正方形和三角形的周长相同。如果等边三角形的一边是32厘米。然后求等边三角形和正方形的周长？

解决方案：

Here,

We have one side of equilateral triangle 32 cm

we are given that the perimeter of the equilateral triangle and square are the same

Perimeter of Equilateral triangle = Side + Side + Side = 3a

Perimeter of Square = Side + Side + Side + Side = 4s

As per the question

Perimeter of Equilateral triangle = Perimeter of Square

3a = 4s

We have a = 32 cm

3 × 32 = 4 × s

96 = 4 × s

s = $\frac{96}{4}$

s = 24 cm

Therefore,

Perimeter of Equilateral triangle = 3a

= 3 × 32

= 96 cm

Perimeter of Square = 4s

= 4 × 24

= 96 cm