在有向图中打印负重量周期

给定一个由V个顶点和E个边缘组成的加权有向图。任务是打印权重之和为负的循环路径。如果不存在这样的路径，则打印“ -1” 。

Input: V = 5, E = 5, Below is the graph: 
 

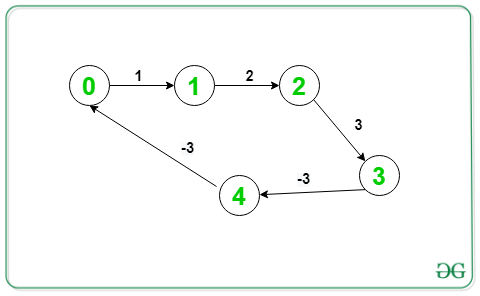
Output: 1 2 3 4 1 
Explanation: 
Given graph contains a negative cycle, (1->2->3->4->1)

Input: V = 5, E = 5, Below is the graph: 
 

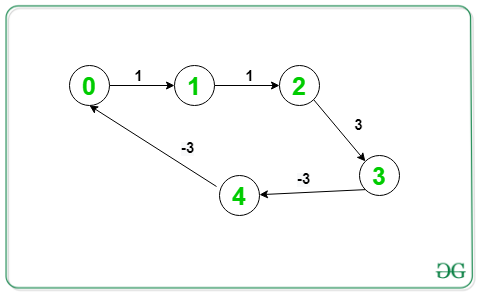
Output: 0 1 2 3 4 0 
Explanation: 
Given graph contains a negative cycle, (0->1->2->3->4->0) 
 

方法：想法是使用Bellman-Ford算法来检测负周期。要打印负周期，请执行Bellman-Ford的第N次迭代，并从该迭代中松弛的任何边上选取一个顶点。使用此顶点及其祖先，可以打印负周期。步骤如下：

• 执行Bellman-Ford算法的N-1次迭代，并放宽每个边(u，v) 。跟踪每个顶点的父级，并将其存储在数组parent []中。
• 现在，再进行一次迭代，如果在第N次迭代中没有发生边缘松弛，则图中不存在负权重的循环。
• 否则，取变量C并从任意边(u，v)存储顶点v ，在第N次迭代中将其放宽。
• 现在，从C顶点开始向其祖先移动，直到找到一个循环并最终将其打印出来。
• 该周期将是负重量的期望周期。

下面是上述方法的实现：

C++

// C++ program for the above approach
#include 
using namespace std;
 
// Structure to represent a weighted
// edge in graph
struct Edge {
    int src, dest, weight;
};
 
// Structure to represent a directed
// and weighted graph
struct Graph {
 
    // V -> Number of vertices,
    // E -> Number of edges
    int V, E;
 
    // Graph is represented as an
    // array of edges
    struct Edge* edge;
};
 
// Creates a new graph with V vertices
// and E edges
struct Graph* createGraph(int V, int E)
{
    struct Graph* graph = new Graph;
    graph->V = V;
    graph->E = E;
    graph->edge = new Edge[graph->E];
    return graph;
}
 
// Function runs Bellman-Ford algorithm
// and prints negative cycle(if present)
void NegCycleBellmanFord(struct Graph* graph,
                         int src)
{
    int V = graph->V;
    int E = graph->E;
    int dist[V];
    int parent[V];
 
    // Initialize distances from src
    // to all other vertices as INFINITE
    // and all parent as -1
    for (int i = 0; i < V; i++) {
 
        dist[i] = INT_MAX;
        parent[i] = -1;
    }
    dist[src] = 0;
 
    // Relax all edges |V| - 1 times.
    for (int i = 1; i <= V - 1; i++) {
        for (int j = 0; j < E; j++) {
 
            int u = graph->edge[j].src;
            int v = graph->edge[j].dest;
            int weight = graph->edge[j].weight;
 
            if (dist[u] != INT_MAX
                && dist[u] + weight < dist[v]) {
 
                dist[v] = dist[u] + weight;
                parent[v] = u;
            }
        }
    }
 
    // Check for negative-weight cycles
    int C = -1;
    for (int i = 0; i < E; i++) {
 
        int u = graph->edge[i].src;
        int v = graph->edge[i].dest;
        int weight = graph->edge[i].weight;
 
        if (dist[u] != INT_MAX
            && dist[u] + weight < dist[v]) {
 
            // Store one of the vertex of
            // the negative weight cycle
            C = v;
            break;
        }
    }
 
    if (C != -1) {
 
        for (int i = 0; i < V; i++)
            C = parent[C];
 
        // To store the cycle vertex
        vector cycle;
        for (int v = C;; v = parent[v]) {
 
            cycle.push_back(v);
            if (v == C
                && cycle.size() > 1)
                break;
        }
 
        // Reverse cycle[]
        reverse(cycle.begin(), cycle.end());
 
        // Printing the negative cycle
        for (int v : cycle)
            cout << v << ' ';
        cout << endl;
    }
    else
        cout << "-1" << endl;
}
 
// Driver Code
int main()
{
    // Number of vertices in graph
    int V = 5;
 
    // Number of edges in graph
    int E = 5;
 
    struct Graph* graph = createGraph(V, E);
 
    // Given Graph
    graph->edge[0].src = 0;
    graph->edge[0].dest = 1;
    graph->edge[0].weight = 1;
 
    graph->edge[1].src = 1;
    graph->edge[1].dest = 2;
    graph->edge[1].weight = 2;
 
    graph->edge[2].src = 2;
    graph->edge[2].dest = 3;
    graph->edge[2].weight = 3;
 
    graph->edge[3].src = 3;
    graph->edge[3].dest = 4;
    graph->edge[3].weight = -3;
 
    graph->edge[4].src = 4;
    graph->edge[4].dest = 1;
    graph->edge[4].weight = -3;
 
    // Function Call
    NegCycleBellmanFord(graph, 0);
 
    return 0;
}

Java

// Java program for the above approach
import java.util.ArrayList;
import java.util.Collections;
 
class GFG{
 
// Structure to represent a weighted
// edge in graph
static class Edge
{
    int src, dest, weight;
}
 
// Structure to represent a directed
// and weighted graph
static class Graph
{
     
    // V. Number of vertices, E.
    // Number of edges
    int V, E;
 
    // Graph is represented as
    // an array of edges.
    Edge[] edge;
}
 
// Creates a new graph with V vertices
// and E edges
static Graph createGraph(int V, int E)
{
    Graph graph = new Graph();
    graph.V = V;
    graph.E = E;
    graph.edge = new Edge[graph.E];
 
    for(int i = 0; i < graph.E; i++)
    {
        graph.edge[i] = new Edge();
    }
 
    return graph;
}
 
// Function runs Bellman-Ford algorithm
// and prints negative cycle(if present)
static void NegCycleBellmanFord(Graph graph, int src)
{
    int V = graph.V;
    int E = graph.E;
    int[] dist = new int[V];
    int[] parent = new int[V];
 
    // Initialize distances from src
    // to all other vertices as INFINITE
    // and all parent as -1
    for(int i = 0; i < V; i++)
    {
        dist[i] = 1000000;
        parent[i] = -1;
    }
    dist[src] = 0;
 
    // Relax all edges |V| - 1 times.
    for(int i = 1; i <= V - 1; i++)
    {
        for(int j = 0; j < E; j++)
        {
            int u = graph.edge[j].src;
            int v = graph.edge[j].dest;
            int weight = graph.edge[j].weight;
 
            if (dist[u] != 1000000 &&
                dist[u] + weight < dist[v])
            {
                dist[v] = dist[u] + weight;
                parent[v] = u;
            }
        }
    }
 
    // Check for negative-weight cycles
    int C = -1;
    for(int i = 0; i < E; i++)
    {
        int u = graph.edge[i].src;
        int v = graph.edge[i].dest;
        int weight = graph.edge[i].weight;
 
        if (dist[u] != 1000000 &&
            dist[u] + weight < dist[v])
        {
             
            // Store one of the vertex of
            // the negative weight cycle
            C = v;
            break;
        }
    }
 
    if (C != -1)
    {
        for(int i = 0; i < V; i++)
            C = parent[C];
 
        // To store the cycle vertex
        ArrayList cycle = new ArrayList<>();
        for(int v = C;; v = parent[v])
        {
            cycle.add(v);
             
            if (v == C && cycle.size() > 1)
                break;
        }
 
        // Reverse cycle[]
        Collections.reverse(cycle);
 
        // Printing the negative cycle
        for(int v : cycle)
            System.out.print(v + " ");
             
        System.out.println();
    }
    else
        System.out.println(-1);
}
 
// Driver Code
public static void main(String[] args)
{
     
    // Number of vertices in graph
    int V = 5;
 
    // Number of edges in graph
    int E = 5;
 
    Graph graph = createGraph(V, E);
 
    // Given Graph
    graph.edge[0].src = 0;
    graph.edge[0].dest = 1;
    graph.edge[0].weight = 1;
 
    graph.edge[1].src = 1;
    graph.edge[1].dest = 2;
    graph.edge[1].weight = 2;
 
    graph.edge[2].src = 2;
    graph.edge[2].dest = 3;
    graph.edge[2].weight = 3;
 
    graph.edge[3].src = 3;
    graph.edge[3].dest = 4;
    graph.edge[3].weight = -3;
 
    graph.edge[4].src = 4;
    graph.edge[4].dest = 1;
    graph.edge[4].weight = -3;
 
    // Function Call
    NegCycleBellmanFord(graph, 0);
}
}
 
// This code is contributed by sanjeev2552

Python3

# Python3 program for the above approach
  
# Structure to represent a weighted
# edge in graph
class Edge:  
    def __init__(self):
        self.src = 0
        self.dest = 0
        self.weight = 0
 
# Structure to represent a directed
# and weighted graph
class Graph:
 
    def __init__(self):
         
        # V. Number of vertices, E.
        # Number of edges
        self.V = 0
        self.E = 0
         
        # Graph is represented as
        # an array of edges.
        self.edge = []
      
# Creates a new graph with V vertices
# and E edges
def createGraph(V, E):
    graph = Graph();
    graph.V = V;
    graph.E = E;
    graph.edge = [Edge() for i in range(graph.E)]
    return graph;
   
# Function runs Bellman-Ford algorithm
# and prints negative cycle(if present)
def NegCycleBellmanFord(graph, src):
    V = graph.V;
    E = graph.E;
    dist =[1000000 for i in range(V)]
    parent =[-1 for i in range(V)]
    dist[src] = 0;
  
    # Relax all edges |V| - 1 times.
    for i in range(1, V):
        for j in range(E):
     
            u = graph.edge[j].src;
            v = graph.edge[j].dest;
            weight = graph.edge[j].weight;
  
            if (dist[u] != 1000000 and
                dist[u] + weight < dist[v]):
             
                dist[v] = dist[u] + weight;
                parent[v] = u;
  
    # Check for negative-weight cycles
    C = -1;   
    for i in range(E):  
        u = graph.edge[i].src;
        v = graph.edge[i].dest;
        weight = graph.edge[i].weight;
  
        if (dist[u] != 1000000 and
            dist[u] + weight < dist[v]):
              
            # Store one of the vertex of
            # the negative weight cycle
            C = v;
            break;
          
    if (C != -1):      
        for i in range(V):      
            C = parent[C];
  
        # To store the cycle vertex
        cycle = []      
        v = C
         
        while (True):
            cycle.append(v)
            if (v == C and len(cycle) > 1):
                break;
            v = parent[v]
  
        # Reverse cycle[]
        cycle.reverse()
  
        # Printing the negative cycle
        for v in cycle:      
            print(v, end = " ");            
        print()  
    else:
        print(-1);
  
# Driver Code
if __name__=='__main__':
      
    # Number of vertices in graph
    V = 5;
  
    # Number of edges in graph
    E = 5;
    graph = createGraph(V, E);
  
    # Given Graph
    graph.edge[0].src = 0;
    graph.edge[0].dest = 1;
    graph.edge[0].weight = 1;
  
    graph.edge[1].src = 1;
    graph.edge[1].dest = 2;
    graph.edge[1].weight = 2;
  
    graph.edge[2].src = 2;
    graph.edge[2].dest = 3;
    graph.edge[2].weight = 3;
  
    graph.edge[3].src = 3;
    graph.edge[3].dest = 4;
    graph.edge[3].weight = -3;
  
    graph.edge[4].src = 4;
    graph.edge[4].dest = 1;
    graph.edge[4].weight = -3;
  
    # Function Call
    NegCycleBellmanFord(graph, 0);
 
# This code is contributed by Pratham76

C#

// C# program for the above approach
using System;
using System.Collections;
using System.Collections.Generic;
 
class GFG {
 
    // Structure to represent a weighted
    // edge in graph
    class Edge {
        public int src, dest, weight;
    }
    // Structure to represent a directed
    // and weighted graph
    class Graph {
 
        // V. Number of vertices, E. Number of edges
        public int V, E;
 
        // graph is represented as an array of edges.
        public Edge[] edge;
    }
 
    // Creates a new graph with V vertices
    // and E edges
    static Graph createGraph(int V, int E)
    {
        Graph graph = new Graph();
        graph.V = V;
        graph.E = E;
        graph.edge = new Edge[graph.E];
 
        for (int i = 0; i < graph.E; i++) {
            graph.edge[i] = new Edge();
        }
 
        return graph;
    }
 
    // Function runs Bellman-Ford algorithm
    // and prints negative cycle(if present)
    static void NegCycleBellmanFord(Graph graph, int src)
    {
        int V = graph.V;
        int E = graph.E;
        int[] dist = new int[V];
        int[] parent = new int[V];
 
        // Initialize distances from src
        // to all other vertices as INFINITE
        // and all parent as -1
        for (int i = 0; i < V; i++) {
 
            dist[i] = 1000000;
            parent[i] = -1;
        }
        dist[src] = 0;
 
        // Relax all edges |V| - 1 times.
        for (int i = 1; i <= V - 1; i++) {
            for (int j = 0; j < E; j++) {
 
                int u = graph.edge[j].src;
                int v = graph.edge[j].dest;
                int weight = graph.edge[j].weight;
 
                if (dist[u] != 1000000
                    && dist[u] + weight < dist[v]) {
 
                    dist[v] = dist[u] + weight;
                    parent[v] = u;
                }
            }
        }
 
        // Check for negative-weight cycles
        int C = -1;
        for (int i = 0; i < E; i++) {
 
            int u = graph.edge[i].src;
            int v = graph.edge[i].dest;
            int weight = graph.edge[i].weight;
 
            if (dist[u] != 1000000
                && dist[u] + weight < dist[v]) {
 
                // Store one of the vertex of
                // the negative weight cycle
                C = v;
                break;
            }
        }
 
        if (C != -1) {
 
            for (int i = 0; i < V; i++)
                C = parent[C];
 
            // To store the cycle vertex
            ArrayList cycle = new ArrayList();
            for (int v = C;; v = parent[v]) {
 
                cycle.Add(v);
                if (v == C && cycle.Count > 1)
                    break;
            }
 
            // Reverse cycle[]
            cycle.Reverse();
 
            // Printing the negative cycle
            foreach(int v in cycle) Console.Write(v + " ");
            Console.WriteLine();
        }
        else
            Console.WriteLine(-1);
    }
 
    // Driver Code
    public static void Main(string[] args)
    {
 
        // Number of vertices in graph
        int V = 5;
 
        // Number of edges in graph
        int E = 5;
 
        Graph graph = createGraph(V, E);
 
        // Given Graph
        graph.edge[0].src = 0;
        graph.edge[0].dest = 1;
        graph.edge[0].weight = 1;
 
        graph.edge[1].src = 1;
        graph.edge[1].dest = 2;
        graph.edge[1].weight = 2;
 
        graph.edge[2].src = 2;
        graph.edge[2].dest = 3;
        graph.edge[2].weight = 3;
 
        graph.edge[3].src = 3;
        graph.edge[3].dest = 4;
        graph.edge[3].weight = -3;
 
        graph.edge[4].src = 4;
        graph.edge[4].dest = 1;
        graph.edge[4].weight = -3;
 
        // Function Call
        NegCycleBellmanFord(graph, 0);
    }
}
 
// This code is contributed by rutvik_56

输出：

1 2 3 4 1

时间复杂度： O(V * E)
辅助空间： O(V)