风筝公式的面积
四边形可以定义为具有四个边、四个顶点和四个角以及一对对角线的多边形类型。四边形的内角和是360°。有各种各样的四边形。正如名字本身所暗示的那样,这个词是两个拉丁词的组合,“Quadri”的意思是四个的变体,“latus”的意思是边。有一些特殊类型的平行四边形,如矩形、正方形、菱形、风筝形等。
风筝被定义为一个四边形,它的四个边被分成两对,其中每对有两条等长的交替边。风筝的元素是四个角、四个边和两个对角线。
特性
- 边被分成两对,每对具有相等长度的相邻边。
AB = AC and BD = CD
- 连接不等边的两个顶点的角度相等。
∠B = ∠C
- 风筝的对角线相交成直角。
- 连接两个相等角度的对角线被风筝的另一个对角线平分。
- 风筝的周长是不等边之和的两倍。
Perimeter = 2 × (AB + BD) = 2 × (AC + CD)
风筝的面积
风筝的面积是风筝两条对角线长度的乘积的一半。
Area of a kite = 1/2 × d1 × d2
where,
d1 = shorter diagonal of the kite
d2 = longer diagonal of the kite
证明:
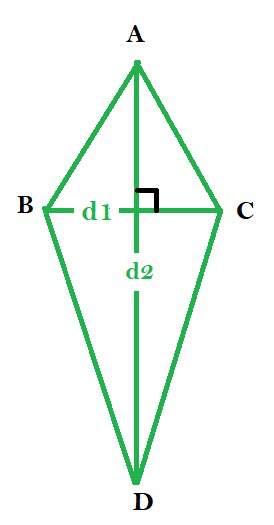
考虑上面显示的图像。形成了两个三角形,即ACD和ABD。
由于对角线 d1 被对角线 d2 一分为二,因此两个三角形的高度都等于(d1)/2 。
三角形 ACD 和 ABD 的公共底是 AD。
因此,底边的长度 = AD 的长度 = d2
三角形的面积由下式给出:
Area of triangle = 1/2 × base × altitude
对于风筝 ABCD,我们可以写
风筝面积 ABCD = 三角形 ACD 面积 + 三角形 ABD 面积
风筝面积 ABCD = 1/2 × d2 × (d1)/2 + 1/2 × d2 × (d1)/2 = 1/2 × d2 × d1
因此,我们可以写
Area of kite ABCD = 1/2 × d1 × d2
示例问题
问题 1. 求对角线长度为 5 厘米和 6 厘米的风筝的面积。
解决方案:
We know the area of a kite is equal to half of the product of both the diagonals.
Given, d1 = 5cm and d2 = 6cm
Thus, we can write,
Area of the kite = 1/2 × 5 × 6 = 5 × 3 = 15 cm2
问题 2. Rahul 想买一块面积为 70 平方码的风筝形地块。给定地块的对角线长度为 20 码,求地块的另一条对角线的长度。
解决方案:
We know the area of a kite is equal to half of the product of both the diagonals.
Given the length of a diagonal is 20 yards, and the area is 70 square yards.
Let the length of the other diagonal be ‘d‘ yards. Then we can write
70 = 1/2 × 20 × d
70 = 10 × d
d = 70/10 = 7 yards
Thus, the length of the other diagonal is 7 yards.
问题 3. 求风筝的对角线,给定对角线之和为 9 个单位,面积为 10 个平方单位。
解决方案:
Given, the area is 10 square units and the sum of diagonals is 9 units.
Let the diagonals be d1 and d2. Then we can write
d1 + d2 = 9
or, d2 = 9 – d1 —- (i)
1/2 × d1 × d2 = 10 —- (ii)
Replacing the value of d2 in eq.(ii), we get
1/2 × d1 × (9 – d1) = 10
d1 × (9 – d1) = 20
d12 – 9d1 + 20 = 0
d12 – 5d1 – 4d1 + 20 = 0
d1(d1 – 5) – 4(d1 – 5) = 0
(d1 – 5) × (d1 – 4) = 0
d1 = ‘5 units’ or ‘4 units’
If the value of d1 is 5 units, then d2 = 9 – d1 = 9 – 5 = 4 units
Else if the value of d1 is 4 units, then d2 = 9 – d1 = 9 – 4 = 5 units
Hence the length of the diagonals are 4 units and 5 units.
问题 4. 求风筝的面积与较小对角线之间的关系,给定较大的对角线,是较小对角线的两倍。
解决方案:
Lets the smaller and larger diagonal be d and 2d respectively.
Let the area of the kite be A.
Then we can write,
A = 1/2 × d × (2d)
A = d2
Thus, the area of the kite is square of the smaller diagonal.