风筝–四边形

风筝是四边形，其中四个边可分为彼此相邻的两对等长边，对角线彼此成直角相交。下图显示了一个风筝：

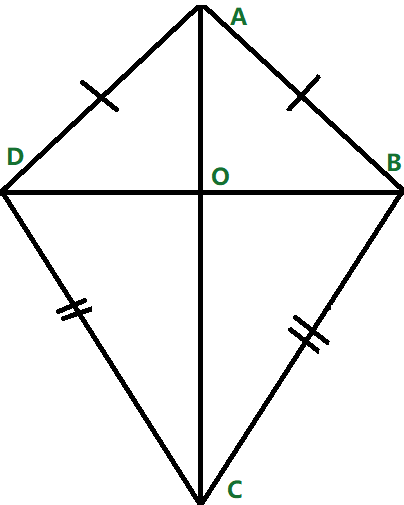
风筝的性质

1. 风筝有2条对角线，彼此成直角相交。
2. 风筝关于它的主要对角线是对称的。
3. 与主对角线相反的角度相等。
4. 风筝可以看作是一对具有共同底数的全等三角形。
5. 风筝的面积是1/2 * d ₁ * d ₂

证明：风筝的对角线相交成直角(垂直)。

证明：

In the figure given above, in ∆ABD and ∆BCD

AB = BC (property of kite)

AD = CD (property of kite)

BD = BD (common side)

Hence ∆ABD ≅ ∆BCD (SSS congruency)

Now, in ∆ABC and ∆ADC

AB = BC (property of kite)

Hence  ∆ABC is an isosceles triangle.

AD = CD (property of kite)

Hence ∆ADC is an isosceles triangle.

∠BAO = ∠BCO

BO = BO (common side)

Thus, ∆ABO ≅ ∆BCO (SAS rule of congruency)

Now we know ∠AOB = ∠BOC

Also, ∠AOB + ∠BOC = 180° (Linear pair)

Hence, ∠AOB = ∠BOC = 90°

Hence diagonals of kite intersect at right angles.

例子

示例1：找到对角线分别为40厘米和35厘米的风筝区域?

解决方案：

The area of the kite with diagonals as d₁ and d₂ is given as 1/2 * d₁ * d₂.

Area = 1/2 * 40 * 35

Area = 700 cm²

Hence the area is 700 cm² 

示例2：找到给定风筝的未知角度?

鉴于：

KLJKL = 100

∠KJL= 40

解决方案：

As we know that the main diagonal bisects the kite into two halves.

Hence the angles ∠KJL = ∠KLM

Hence ∠KLM = 100

Also since the sum of all angles of the quadrilateral is 360.

Hence ∠JML = 120

示例3：风筝形区域的面积为450平方厘米，其对角线之一的长度为50厘米。一个男人想穿过另一个对角线穿过田野。找到男人必须走的距离。

解决方案：

Given,

Area of a kite = 450 cm²

Length of one diagonal = 50 cm

We know that the Area of Kite = 1/2 * d₁ * d₂

450 = 1/2 × 50 × d₂

d₂ = 18 cm

Hence the other man has to travel a distance of 18 cm.