不等边三角形公式
三角形是由三个点连接形成的平面形状,其中两个不共线。如果所有三个点都共线,则所得形状将不会被视为三角形。三角形,顾名思义,包含三个角,总和总是180度。它是一个二维形状,因为它是一个由三个共面点联合而成的封闭图形。这意味着它是一个没有厚度的扁平形状。三角形 ABC 的三个顶点分别为 A、B 和 C,三个边分别为 AB、BC 和 CA,如下图所示。
不等边三角形
不等边三角形是一个三角形,其中三个边中的每一个都具有不同的长度,并且三个角中的每一个都具有不同的测量值。然而,不等角三角形的所有内角的总和不受各种测量值的影响。不等边三角形的三个内角之和总是180°,满足三角形的角和条件。
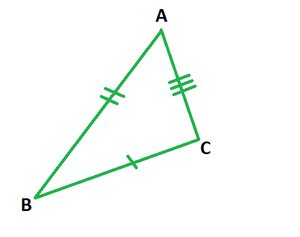
不等边三角形公式
不等边三角形有两个与其周长和面积相关的主要公式。
不等边三角形的周长
不等边三角形的周长定义为其所有三个边的总和。
P = (x + y + z) units
where, x, y and z are the side lengths of the triangle.
不等边三角形的面积
A = (1/2) × b × h sq. units
where, b is the base and h is the height (altitude) of the triangle.
如果给出三个边的长度而不是底和高,我们使用 Heron 公式计算面积,该公式由下式给出,
A = √(s(s – x)(s – y)(s – z)) sq. units
Here s denotes the semi-perimeter of the triangle, that is, s = (x + y + z)/2, and x, y, and z denotes the sides of the triangle.
示例问题
问题 1. 求边长分别为 10 cm、15 cm 和 6 cm 的不等边三角形的周长。
解决方案:
We have, x = 10, y = 15 and z = 6.
Use the formula P = (x + y + z) to find the perimeter.
P = (10 + 15 + 6)
= 31 cm
问题 2. 求两条边长分别为 3 厘米和 7 厘米,周长为 20 厘米的不等边三角形的第三条边的长度。
解决方案:
We have, x = 3, y = 7 and P = 20.
Use the formula P = (x + y + z) to find the unknown length.
P = (x + y + z)
=> 20 = (3 + 7 + z)
=> 20 = 10 + z
=> z = 10 cm
问题 3. 求边长分别为 8 cm、6 cm 和 10 cm 的不等边三角形的面积。
解决方案:
We have, x = 8, y = 6 and P = 10.
Use the formula s = (x + y + z)/2 to find the semi-perimeter.
s = (8 + 6 + 10)/2
= 24/2
= 12 cm
Use the formula √(s(s – x)(s – y)(s – z)) to find the area.
A = √(12(12 – 8)(12 – 6)(12 – 10))
= √(12(4)(6)(2))
= √576
= 24 sq. cm
问题 4. 求底边为 20 厘米,高为 10 厘米的不等边三角形的面积。
解决方案:
We have, b = 20 and h = 10.
Area of a scalene triangle given its base and height = 1/2 × b × h
= 1/2 × 20 × 10
= 100 sq. cm
Thus, the area of the given scalene triangle is 100 sq. cm.
问题 5. 如果斜角三角形的两条边长分别为 28 厘米和 15 厘米,半周长为 42 厘米,则求其面积。
解决方案:
We have, x = 28, y = 15 and s = 42.
Use the formula s = (x + y + z)/2 to find the unknown third side.
=> 42 = (28 + 15 + z)/2
=> 84 = 33 + z
=> z = 41 cm
Use the formula √(s(s – x)(s – y)(s – z)) to find the area.
A = √(42(42 – 28)(42 – 15)(42 – 41))
= √(42(14)(27)(1))
= √15876
= 126 sq. cm