复数的绝对值

复数定义为 a+ib 形式的数，其中 a 是实部，ib 是复数的虚部，其中 i 称为 iota，b 是实数。 i 的值为 √(-1)。或者换句话说，复数是实数和虚数的组合。例如，5+11i、10+20i 等。

• 实数：实数是存在于数系中的数，可以是正数、负数、整数、有理无理数等。例如，23、-3、3/6。
• 虚数：虚数是那些不是实数的数字。例如，√3、√11 等。
• 零复数：零复数是实部和虚部都等于零的数字。例如，0+0i。

复数的图形表示：

复数的图形表示如下图所示：

这里，复数的实部表示在水平轴上，而复数的虚部表示在垂直轴上。

绝对值

数字的绝对值（模数）是数字与零的距离。绝对值始终以模数 (|z|) 表示，其值始终为正。所以，复数 Z = a + ib 的绝对值是

|z| = √ (a² + b²)

所以，复数的绝对值是实部平方和虚部平方和的正平方根，即

证明：

让我们考虑复数 z 的模从 0 扩展到 z 并且 a、b 实数的模从 a 扩展到 0 和 b 到 0。所以这些值创建了一个直角三角形，其中 0 是顶点锐角的

因此，使用毕达哥拉斯定理，我们得到，

|z| ² = |a| ² + |b| ²

|z| = √ a ² + b ²

现在，在复数集合中，z ₁ > z ₂或 z ₁ < z ₂没有意义，但 |z ₁ | > |z ₂ |或 |z ₁ | < |z ₂ |有意义是因为 |z ₁ |和 |z ₂ |是一个实数。

复数模的性质：

1. |z| = 0 <=> z = 0i，即 Re(z) = 0 和 Im(z) = 0
2. |z| = | $\bar{z}$ | = |-z|
3. -|z| ≤ Re(z) ≤ |z|, -|z| ≤ Im(z) ≤ |z|
4. z。 $\bar{z}$ = |z ² |
5. |z ₁ z ₂ | = |z ₁ ||z ₂ |
6. |z ₁ / z ₂ | = |z ₁ |/|z ₂ |
7. |z ₁ + z ₂ | ² = |z ₁ | + |z ₂ | + 2Re(z ₁ $\bar{z}_2$ )
8. |z ₁ – z ₂ | ² = |z ₁ | + |z ₂ | – 2Re(z ₁ $\bar{z}_2$ )
9. |z ₁ + z ₂ | ² ≤ |z ₁ | + |z ₂ |
10. |z ₁ – z ₂ | ² ≥ |z ₁ | – |z ₂ |
11. |az ₁ – bz ₂ | ² + |bz ₁ + az ₂ | ² = (a ² + b ² )(|z ₁ | ² + |z ₂ | ² ) 或 |z ₁ – z ₂ | ² + |z ₁ + z ₂ | ² = 2(|z ₁ | ² + |z ₂ | ² )
12. |z ⁿ | = |z| ⁿ
13. 1/z = a – ib/a ² + b ² = 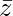$\bar{z}$ /|z| ²

例子：

(i) z = 3 + 4i

|z| = √(3² + 4²)

= √(9 + 16)

= √25

= 5

(ii) z = 5 + 6i

|z| = √(5² + 6²)

= √(25 + 36)

= √61

复数角度

复数的角度或复数的自变量是在复平面或argand平面上表示的复数方向上从实轴倾斜的角度。

θ = tan^-1(b/a)

or 

arg(Z) = tan^-1(b/a)

在这里，Z = a + ib

复数的天使或自变量的性质：

• arg(Z ⁿ ) = n arg(Z)
• arg (Z ₁ / Z ₂ ) = arg (Z ₁ ) – arg (Z ₂ )
• arg (Z ₁ Z ₂ ) = arg (Z ₁ ) + arg (Z ₂ )

例子：

(i) z = 2 + 2i

θ = tan^-1(2/2)

= tan^-1(1)

= 45°

(ii) z = -4 + 4i

θ = tan^-1(4/-4)

= tan^-1(-1)

= -45°

It is important to note here that  the angle θ =-45° is in 4^th quadrant, 

while we always measure angle with the positive x-axis. 

So, we will have to add 180° to the answer to obtain the real  opposite angle.

So, θ = 180° + (-45°)

= 135°

So , the above complex number will make an angle of 135° with the positive x-axis.

复数的极坐标形式

复数的极坐标形式也是表示复数的一种方式。通常，我们表示复数，如 Z = a + ib，但在极坐标形式中，复数以模数和参数的组合表示。这里，复数的模称为复数的绝对值，而自变量称为复数的角度。

Z = r(cos θ + isin θ)

证明：

假设我们有一个复数 Z = a+ib。所以我们画了一个 argand 平面，它是一个我们可以表示复数的平面，它也被称为复平面。在 argand 平面图中，水平线代表实轴，垂直线代表虚轴。现在我们在实轴上绘制 a，在虚轴上绘制 b。现在我们将向量 Z 表示为从 0 开始且尖端位于坐标 (a, b) 的位置向量。假设θ是 Z 在实轴上所成的角度，0 到 Z 之间的距离为 r（也称为向量 Z 的大小/绝对值）。这里，(r, θ ) 对被称为 Z 的极坐标。

根据图，我们有一个直角三角形

所以，使用毕达哥拉斯定理，我们得到，

r = |z| ² = |a| ² + |b| ²

r = |z| = √ a ² + b ²

这是模数

现在我们找到θ的值

tan θ = (a/b)

θ = tan ^-1 (a/b)

这是论据

现在我们找到复数的极坐标形式：

所以使用三角公式，我们得到

cos θ = a/r

现在将两边乘以 r 我们得到

rcos θ = a

罪 θ = b/r

现在将两边乘以 r 我们得到

rsin θ = b

所以，我们得到

Z = rcos θ + irsin θ

Z = r(cos θ + isin θ)

这里，r 是复数的绝对值，θ 是复数的自变量。

复数的指数形式：

复数的指数形式使用正弦和余弦的三角比来将复指数定义为指数形式的旋转平面。复数的指数形式通常由欧拉恒等式给出，以著名数学家莱昂哈德欧拉命名。给出如下：

Z = re^iθ

例子：

(i) Given that r = 5 and  θ = 45°. Find the polar form of the complex number

z = rcosA + (rsinA)i

z = 5cos45° + (5sin45°)i

= 5(1/√2) + (5 (1/√2))i

= 5/√2 + (5/√2)i

(ii) Given that r = 6 and  θ = 30°. Find the polar form of the complex number

z = rcosA + (rsinA)i

z = 6cos30° + (6sin30°)i

= 6(√3/2) + (6(1/2))i

= 3√3 + 3i

将矩形形式转换为极坐标形式：

让我们借助一个例子来讨论这个概念：

Suppose we have a complex number, i.e., Z = 3 + 4i

It is in rectangular form so now we have to convert it to a polar form.

Step 1: So, first, we will calculate the modulus of the complex numbers and then the angle.

|Z|= √(3² + 4²)

= √(9 + 16)

= √25

= 5

Step 2: Now we find the angle of the complex number,

θ  = tan^-1(y/x)

= tan^-1(4/3)

= 53.1°

Step 3: As we know that the formula of polar form is:

Z = r(cos θ + isin θ)

Now put the value of r and θ in this equation, we get

Z = 5(cos 53.1 + isin 53.1)

Hence, the polar from of 3 + 4i is 5(cos 53.1 + isin 53.1)

示例问题

问题 1. 求 z = 4 + 8i 的绝对值

解决方案：

Given complex number is z = 4 + 8i

As we know that the formula of absolute value is

|z| = √ (a² + b²)

So, a = 4, and b = 8, we get

|z| = √(4² + 8²)

|z| = √80

问题 2. 求 z = 2 + 4i 的绝对值

解决方案：

Given complex number is z = 2 + 4i

As we know that the formula of absolute value is

|z| = √ (a² + b²)

So, a = 2, and b = 4, we get

|z| = √(2² + 4²)

|z| = √20

问题3.求复数的角度：z = √3 + i

解决方案：

Given complex number is z = √3 + i

As we know, that 

θ = tan^-1(b/a)

So, a = √3 , and b = 1, we get

θ = tan^-1(1/ √3 )

θ = 30°

问题4.求复数的角度：z = 6 + 6i

解决方案：

Given complex number is z = 6 + 6i

As we know, that 

θ = tan^-1(b/a)

So, a = 6 , and b = 6, we get

θ = tan^-1(6/6)

θ = 45°

问题 5. 将 z = 5 + 5i 转换为极坐标形式

解决方案：

Given complex number is z = 5 + 5i

As we know that 

Z = r(cos θ + isin θ) …(1)

Now, we find the value of r

r = √(5² + 5²)

r = √(25 + 25)

r = √50

Now we find the value of θ

θ = tan^-1(5/5)

θ = tan^-1(1)

θ = 45°

Now put all these values in eq(1), we get

Z = √50(cos 45° + isin 45°)

问题 6. 将 z = 2 + √3i 转换为极坐标形式

解决方案：

Given complex number is z = 2 + 2√3i

As we know that 

Z = r(cos θ + isin θ) …(1)

Now, we find the value of r

r = √(2² + (2√3)²)

r = √(4 + 12)

r = √16

r = 4

Now we find the value of θ

θ = tan^-1(2√3/2)

θ = tan^-1(√3)

θ = 60°

Now put all these values in eq(1), we get

Z = 4(cos 60° + isin 60°)