复数

复数是形式(a + ib)的数字，其中a和b是实数，而i是一个称为iota的虚构单位，代表√-1。例如，2 + 3i是一个复数，其中2是实数，3i是虚数。

需要复数

最初，我们只有自然数( N )的集合，该自然数被扩展为形成整数( I )的集合，因为等式(x + a = b)对于a> b是不可解的，其中a，b∈N。

然后，这组整数(I)扩展为一组有理数( Q )，因为形式(xa = b)的每个方程在a≠0且a，b∈I时不是唯一可解的。

同样，这组整数(Q)扩展为一组实数(有理数和无理数)( R )，因为形式(x ² = a)的每个方程在a> 0和a∈Q即x ^{2时都不可解。} = 5，因为不存在平方为5的有理数。因此，将无理数的概念赋予√2，√3，√5等数字。

最后，我们将这组实数(R)扩展为一组复数( C )，因为形式(x ² = a)的方程不可解，其中a <0和a∈R即x ² + 5 = 0，因为没有任何平方为-5的实数。因此，复数(虚数)的概念被赋予诸如√-1，√-2，√-3，√-5等数字，其中√-1被称为虚数单位(iota)，并由符号i表示。 (i ² = -1)。

复数的分类

众所周知，复数的标准形式为z =(a + ib) ，其中a，b∈R，而i为iota(虚数单位)。因此，根据a(称为实部)和b(称为虚部)的值，复数可分为四种类型：

1. Zero Complex Number (a = 0 & b = 0)

Example: 0 (zero)

2. Purely Real Numbers (a ≠ 0 & b = 0)

Examples: 2, 3, 7, etc.

3. Purely Imaginary Numbers (a = 0 & b ≠ 0)

Examples: -7i, -5i, -i, i, 5i, 7i, etc.

4. Imaginary Numbers (a ≠ 0 & b ≠ 0)

Examples: (-1 – i), (1 + i), (1 – i), (2 + 3i), etc.

不同形式的复数

1. Rectangular Form also called Standard Form = (a + i b)

Examples: (5 + 5i), (-7i), (-3 – 4i), etc.

2. Polar Form = r [cos(θ) + i sin(θ)]

Examples: [cos(π/2) + i sin(π/2)], 5[cos(π/6) + i sin(π/6)], etc.

3. Exponential Form = $r\cdot e^{i\theta}$

Examples: eⁱ⁽⁰⁾, e^i(π/2), 5.e^i(π/6), etc.

注意：上面讨论的复数的所有三种形式都是可互换的。

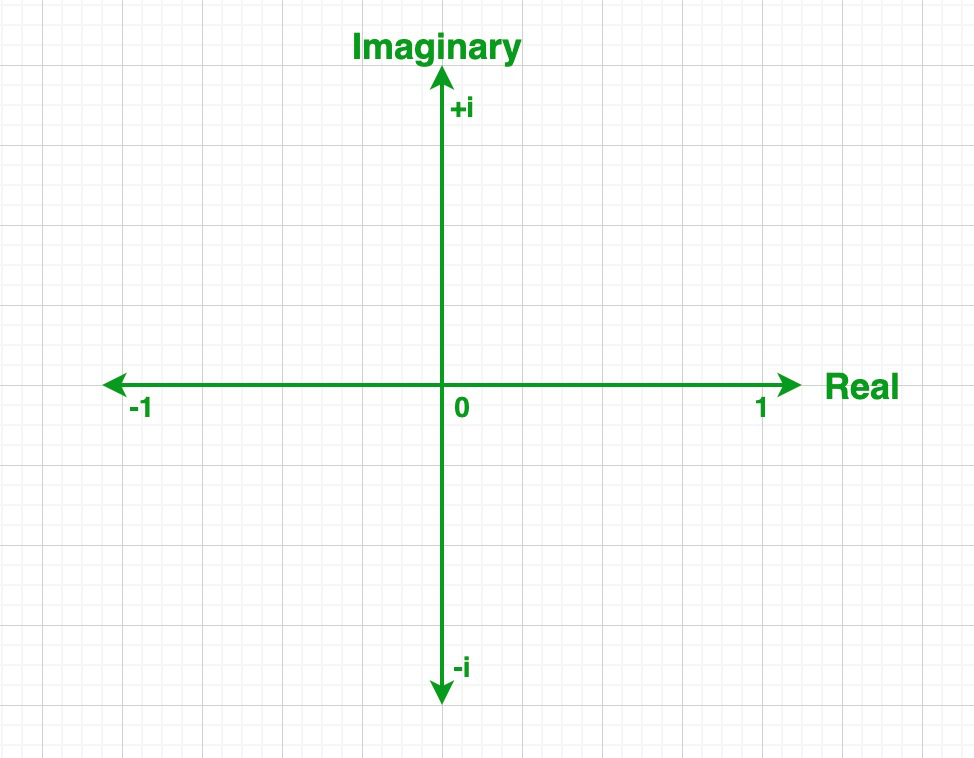
复杂平面

唯一表示复数的平面称为“复数平面”或“ Argand平面”或“ Gaussian平面” 。

The Complex plane has two axes:

1. X-axis

• All the purely real complex numbers are uniquely represented by a point on it.
• Real part Re(z) of all complex numbers are plotted with respect to it.
• That’s why X-axis is also called Real axis.

2. Y-axis

• All the purely imaginary complex numbers are uniquely represented by a point on it.
• Imaginary part Im(z) of all complex numbers are plotted with respect to it.
• That’s why Y-axis is also called Imaginary axis.

复数的几何表示

众所周知，每个复数(z = a + ib)由复数平面上的唯一点p(a，b)表示，而复数平面上的每个点都表示唯一的复数。

To represent any complex number z = (a + i b) on the complex plane follow these conventions:

• Real part of z (Re(z) = a) becomes the X-coordinate of the point p
• Imaginary part of z (Im(z) = b) becomes the Y-coordinate of the point p

And finally z (a + i b) ⇒ p (a, b) which is a point on the complex plane.

示例1：绘制这些复数z = 3 + 2 i在复平面上。

解决方案：

Given:

z = 3 + 2 i

So, the point is z(3, 2). Now we plot this point on the below graph, here in this graph x-axis represents the real part and y-axis represents the imaginary part. 

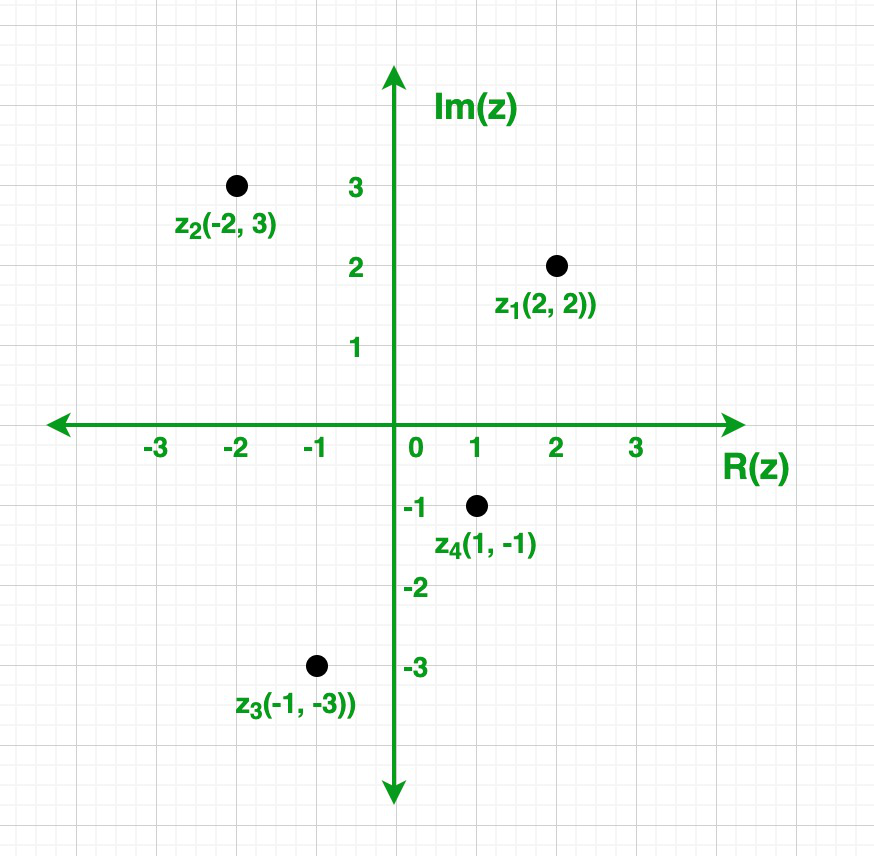
示例2：在复数上绘制这些复数z ₁ =(2 + 2 i)，z ₂ =(-2 + 3 i)，z ₃ =(-1 – 3 i)，z ₄ =(1 – i)飞机。

解决方案：

Given:

z₁ = (2 + 2 i)

z₂ = (-2 + 3 i)

z₃ = (-1 – 3 i)

z₄ = (1 – i)

So, the points are z₁ (2, 2), z₂(-2, 3), z₃(-1, -3), and z₄(1, -1). Now we plot these points on the below graph, here in this graph x-axis represents the real part and y-axis represents the imaginary part.