正弦定律公式
三角学是数学领域,它涉及直角三角形的边的比率与其角度之间的关系。用于检查这种连接的三角比是正弦、余弦、正切、余切、正割和余割。三角学派生自术语“Trigonon”和“Metron”,分别表示三角形和度量。它是研究直角三角形的边和角之间的关系。因此,它通过使用基于此连接的公式和恒等式来帮助确定直角三角形的未知尺寸。
正弦三角比
三角比是两个直角三角形边的比率。这些比率之一是正弦比率。角的正弦是对边的长度除以斜边的长度的比值。
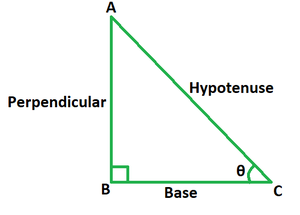
如果 θ 是直角三角形的底边和斜边所形成的角度,那么,
sin θ = Perpendicular/Hypotenuse
正弦定律公式
正弦定律公式用于将三角形边的长度与连续角的正弦相关联。它是三角形边的长度与其他两个剩余边产生的角度的正弦之比。除 SAS 和 SSS 三角形外,正弦定律公式适用于每个三角形。它指出,
a/sin A = b/sin B = c/sin C
where,
a, b, and c are the lengths of the triangle ABC
A, B, and C are the angles of the triangle ABC
推导

To derive the sine law, consider the two oblique triangles illustrated below.
In the left triangle, we have:
sin A = h/b
=> h = b sin A ……….. (1)
In the right triangle, we have:
sin B = h/a
=> h = a sin B ……….. (2)
From (1) and (2), we get
a sinB = b sinA
=> a/sinA = b/sinB
Similarly, a/sinA = c/sinC.
By combining the two formulas above, we get the sine law as shown below.
a/sin A = b/sin B = c/sin C
示例问题
问题 1. 给定三角形 ABC,a = 20 个单位,c = 25 个单位,∠C = 30º。求三角形的∠A。
解决方案:
We have, a = 20 units, c = 25 units and ∠C = 30º.
Use the sine formula to find A.
a/sin A = c/sin C
20/sin A = 25/sin 30
sin A = 0.40
A = 23.5°
问题 2. 给定三角形 ABC,b = 15 个单位,c = 20 个单位,∠C = 60º。求三角形的∠B。
解决方案:
We have, b = 15 units, c = 20 units and ∠C = 60º
Use the sine formula to find B.
b/sin B = c/sin C
15/sin B = 20/sin 60
sin B = 0.649448
B = 40.5°
问题 3. 给定三角形 ABC,b = 30 个单位,c = 40 个单位,∠C = 30º。求三角形的∠B。
解决方案:
We have, b = 30 units, c = 10 units and ∠C = 30º.
Use the sine formula to find B.
b/sin B = c/sin C
30/sin B = 40/sin 30
sin B = 0.374607
B = 22°
问题 4. 给定三角形 ABC,b = 5 个单位,c = 10 个单位,∠C = 60º。求三角形的∠B。
解决方案:
We have, b = 5 units, c = 10 units and ∠C = 60º
Use the sine formula to find B.
b/sin B = c/sin C
5/sin B = 10/sin 60
sin B = 0.433659
B = 25.7°
问题 5. 给定三角形 ABC,b = 9 个单位,c = 18 个单位,∠C = 75º。求三角形的∠B。
解决方案:
We have, b = 9 units, c = 18 units and ∠C = 75º
Use the sine formula to find B.
b/sin B = c/sin C
9/sin B = 20/sin 75
sin B = 0.483282
B = 28.9°
问题 6. 给定三角形 ABC,b = 11 个单位,c = 22 个单位,∠C = 70º。求三角形的∠B。
解决方案:
We have, b = 11 units, c = 22 units and ∠C = 70º.
Use the sine formula to find B.
b/sin B = c/sin C
11/sin B = 22/sin 70
sin B = 0.469472
B = 28°
问题 7. 给定三角形 ABC,b = 8 个单位,c = 13 个单位,∠C = 85º。求三角形的∠B。
解决方案:
We have, b = 8 units, c = 13 units and ∠C = 85º.
Use the sine formula to find B.
b/sin B = c/sin C
8/sin B = 13/sin 85
sin B = 0.612907
B = 37.8°