隐分化
隐式微分是一种利用链式法则来区分隐式定义函数的方法。明确地找到函数然后进行微分一般不容易。相反,我们可以完全微分 f(x, y) 并求解方程的其余部分以找到 dy/dx 的值。即使可以显式求解原始方程,由全微分得出的公式通常也更简单且更易于使用。在跳到主题之前,让我们看一个示例问题。这将帮助您非常容易地理解这些概念。
问题:求以原点为圆心的圆上任意一点的切线斜率方程?
解决方案:
To solve this problem we need to find the equation of the function in terms of x (i.e. y = f(x)) and then find f'(x). Let us first write the general equation for a circle.
x2 + y2 = r2
In this form of the equation, the function is expressed in terms of both y and x. This function is known as implicit function as y is not explicitly defined as a function of x i.e. this function is not in the form y = f(x). Let’s find the derivative explicitly. To solve this explicitly,
- Solve the equation for y
- Differentiate the function
- Substitute back y
In this case,
x2 + y2 = r2
Subtract x2 from both sides:
y2 = r2 – x2
Taking square root on both sides:
y = ± ![]()
Case 1:
=> y = (r2 − x2)1/2
Derivative by Chain Rule:

Since,
![]()
Case 2:
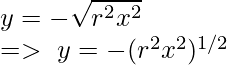
Derivative by Chain Rule:
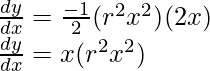
Since, ![]()
In either case, the answer is same i.e dy/dx = – x/y
But our main intention in this article would be to try to solve the problem without explicitly finding the value of f(x). In this case finding the function f(x) was quite easy but it wouldn’t be the same case elsewhere.
基本前提
- 链式法则:链式法则是计算复合函数导数的公式。也就是说,如果 f 和 g 是可微函数,那么链式法则将它们的复合 f ∘ g 的导数表示为: f(g(x))' = f'(g(x)) * g'(x)
- 隐式与显式函数:函数可以是显式的或隐式的:
- 显式: y = f(x) 例如 y = x 2
- 隐式: f(x, y) = 0 例如 y + x 2 = 5
这里我们只用了 2 个变量 x 和 y 来定义隐式函数。但是你可以有任意数量的变量。
求解隐式微分的方法
- 对等式两边对 x 求微分。
- 遵循差异化规则。
- 使用链式法则来区分涉及 y 的表达式。
- 求解 dy/dx 的方程。
现在,我们将使用隐式微分来解决上述问题。
解决方案:
Given above equation is:
x2 + y2 = r2
Step 1: Differentiate both sides wrt to x and follow the differentiatopn
![]()
Step 2: Using the chain rule
![]()
Step 3: Simplify the equation
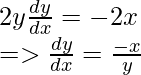
We got the same expected answer as in case of explicit differentiation.
我们已经成功地证明了隐式和显式微分给出了相同的结果。让我们看一些更多的例子来清楚地理解这个概念。有时,隐式方式在显式方式很难或不可能的情况下起作用。在以下所有示例中,我们必须找到 dy/dx 的值。
例子
示例 1:求 y + x + 5 = 0 的导数?
解决方案:
Using explicit differentiation:
y + x + 5 = 0
=> y = -(x + 5)
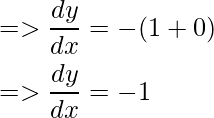
Using implicit differentiation:
y + x + 5 = 0
Differentiating both sides wrt x
![]()
Isolate dy/dx
示例 2:求 y 5 – y = x 的导数?
解决方案:
Given equation:
y5 – y = x
示例 3:求 10x 4 – 18xy 2 + 10y 3 = 48 的导数?
解决方案:
Given equation:
10x4 – 18xy2 + 10y3 = 48
Differentiating both sides wrt x
(the differentiation of term xy2 is explained below)
On simplifying we get:
Keeping all the terms involving dy/dx on left and rest terms on right side of equation:
![]()
Dividing both sides by 2
Finally isolate dy/dx
For the term xy2 we used the Product Rule: (fg)’ = f g’ + f’ g
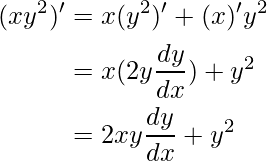
示例 4:求 x 4 + 2y 2 = 8 的导数?
解决方案:
Given equation:
x4 + 2y2 = 8
示例 5:求 y = sin -1 (x) 的导数?
解决方案:
Given equation:
y = sin-1(x)
=> sin y = x
We can simplify it more by using the below observation:
sin2(y) + cos2(y)
We know,
sin(y) = x
=> x2 + cos2(y) = 1
=> cos2(y) = 1 – x2
=> cos(y) = ![]()
Substituting the value, we get

概括
隐式求导数(当函数不能轻易求解 y 时很有用):
- 关于 x 的微分
- 收集一侧的所有 dy/dx
- 求解 dy/dx
隐式微分可以帮助我们解决反函数。要导出反函数,请在没有反函数的情况下重述它,然后使用隐式微分。