穿过单个点的最大不同线

给定$N$两点代表的线$(x1, y1)$和$(x2, y2)$ 。任务是找到可以通过单个点的最大线数，而不会重叠(或覆盖)任何其他线。我们可以移动任何线，但不能旋转它。

例子：

Input : Line 1 : x1 = 1, y1 = 1, x2 = 2, y2 = 2
        Line 2 : x2 = 2, y1 = 2, x2 = 4, y2 = 10
Output : 2
There are two lines. These two lines are not 
parallel, so both of them will pass through
a single point.


Input : Line 1 : x1 = 1, y1 = 5, x2 = 1, y2 = 10
        Line 2 : x2 = 5, y1 = 1, x2 = 10, y2 = 1
Output : 2

• 将线表示为一对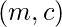$(m, c)$哪里可以给定线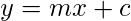$y=mx+c$ ，称为线斜率形式。现在我们可以看到可以更改任何行的c ，但是不能修改m 。
• 给定(c1≠c2) ，具有m的相同值的线平行。同样，没有两条平行线可以通过同一点而不相互重叠。
• 因此，我们的问题减少到从给定的线集中找到不同的斜率值。

我们可以将线的斜率计算为$\frac{(y2-y1)}{(x2-x1)}$ ，将它们添加到集合中并计算集合中斜率的不同值的数量。但是我们必须分别处理垂直线。
因此，如果$x1 = x2$然后， lope = INT_MAX 。
否则，斜率= $\frac{(y2-y1)}{(x2-x1)}$ 。

下面是该方法的实现。

C++

// C++ program to find maximum number of lines
// which can pass through a single point
#include 
using namespace std;
  
// function to find maximum lines which passes
// through a single point
int maxLines(int n, int x1[], int y1[], 
                int x2[], int y2[])
{
    unordered_set s;
  
    double slope;
    for (int i = 0; i < n; ++i) {
        if (x1[i] == x2[i])
            slope = INT_MAX;
        else
            slope = (y2[i] - y1[i]) * 1.0 
                    / (x2[i] - x1[i]) * 1.0;
  
        s.insert(slope);
    }
  
    return s.size();
}
  
// Driver program
int main()
{
    int n = 2, x1[] = { 1, 2 }, y1[] = { 1, 2 },
            x2[] = { 2, 4 }, y2[] = { 2, 10 };
    cout << maxLines(n, x1, y1, x2, y2);
    return 0;
}
// This code is written by
// Sanjit_Prasad

Java

// Java program to find maximum number of lines
// which can pass through a single point
  
import java.util.*;
import java.lang.*;
import java.io.*;
  
class GFG{
  
// function to find maximum lines which passes
// through a single point
static int maxLines(int n, int x1[], int y1[], 
                    int x2[], int y2[])
{
    Set s=new HashSet();
  
    double slope;
    for (int i = 0; i < n; ++i) {
        if (x1[i] == x2[i])
            slope = Integer.MAX_VALUE;
        else
            slope = (y2[i] - y1[i]) * 1.0 
                    / (x2[i] - x1[i]) * 1.0;
  
        s.add(slope);
    }
  
    return s.size();
}
  
// Driver program
public static void main(String args[])
{
    int n = 2, x1[] = { 1, 2 }, y1[] = { 1, 2 },
            x2[] = { 2, 4 }, y2[] = { 2, 10 };
    System.out.print(maxLines(n, x1, y1, x2, y2));
}
}
// This code is written by
// Subhadeep

Python3

# Python3 program to find maximum number 
# of lines which can pass through a 
# single point
import sys
# function to find maximum lines 
# which passes through a single point
def maxLines(n, x1, y1, x2, y2):
  
    s = [];
  
    slope=sys.maxsize;
    for i in range(n):
        if (x1[i] == x2[i]):
            slope = sys.maxsize;
        else:
            slope = (y2[i] - y1[i]) * 1.0 /(x2[i] - x1[i]) * 1.0;
  
        s.append(slope);
  
    return len(s);
  
# Driver Code
n = 2;
x1 = [ 1, 2 ];
y1 = [1, 2];
x2 = [2, 4];
y2 = [2, 10];
print(maxLines(n, x1, y1, x2, y2));
  
# This code is contributed by mits

C#

// C# program to find maximum number of lines
// which can pass through a single point
using System;
using System.Collections.Generic;
  
class GFG
{
  
// function to find maximum lines which passes
// through a single point
static int maxLines(int n, int []x1, int []y1, 
                    int []x2, int []y2)
{
    HashSet s = new HashSet();
  
    double slope;
    for (int i = 0; i < n; ++i) 
    {
        if (x1[i] == x2[i])
            slope = int.MaxValue;
        else
            slope = (y2[i] - y1[i]) * 1.0
                    / (x2[i] - x1[i]) * 1.0;
  
        s.Add(slope);
    }
  
    return s.Count;
}
  
// Driver code
public static void Main()
{
    int n = 2;
    int []x1 = { 1, 2 }; int []y1 = { 1, 2 };
    int []x2 = { 2, 4 }; int []y2 = { 2, 10 };
    Console.Write(maxLines(n, x1, y1, x2, y2));
}
}
  
/* This code contributed by PrinciRaj1992 */

输出：

2

时间复杂度： $O(N)$