📌  相关文章
📜  找到最小的四位数，它是一个完美的正方形

📅  最后修改于: 2022-05-13 01:54:13.662000             🧑  作者: Mango

找到最小的四位数，它是一个完美的正方形

用于表示数量和进行计算的算术值被定义为数字。像“4,5,6”这样代表数字的符号称为数字。没有数字，我们就无法计算事物、日期、时间、金钱等。这些数字也用于测量和标记。

数字的属性使它们有助于对它们执行算术运算。这些数字可以写成数字形式，也可以写成文字。

例如3 写成 3，35 写成 35 等等。学生可以用 1 到 100 的数字写出更多信息。

有不同类型的数字，我们可以学习。它们是整数和自然数，奇数和偶数，有理数和无理数等

什么是数字系统？

数字系统是一种通过书写显示数字的方法，这是一种通过以数学方式使用数字或符号来表示给定集合的数字的数学方式。以逻辑方式使用数字或符号表示数字的书写系统被定义为数字系统。

我们可以用 0 到 9 的数字组成所有的数字。有了这些数字，任何人都可以创造无限的数字。

例如 156,3907, 3456, 1298, 784859 等

什么是平方根？

平方根数的值，其自身相乘得出原始数。假设 a 是 b 的平方根，那么它表示为 a = √b 或者我们可以将相同的方程表示为 a 2 = b。在这里，我们用来表示数字根的'√'这个符号称为部首。与自身相乘时的正数表示该数的平方。任何正数的平方的平方根给出原始数。

例如，4 的平方是 16，4 2 = 16，16 的平方根，√16 = 4。由于 4 是完全平方，因此很容易找到这些数的平方根，但对于不完美的正方形，真的很棘手。

平方根表示为“√”。它被称为激进符号。使用此符号将数字“a”表示为平方根可以写为： “√a”，其中 a 是数字。

这里激进符号下的数字称为radicand。例如，4 的平方根也表示为 4 的根号。两者都表示相同的值。

求平方根的公式是： b = √a

平方根的性质

它被定义为一个以正数作为输入并返回给定输入数的平方根的一对一函数。

f(x) = √x

例如，如果 x = 9，则函数将输出值返回为 3。

这些是平方根的属性，如下所示：

  • 如果一个数是一个完全平方数，那么肯定存在一个完全平方根。
  • 如果一个数字以偶数个零（0）结尾，那么我们可以有一个平方根。
  • 这两个平方根值可以相乘。例如，√3 可以乘以√2，则结果为√6。
  • 当两个相同的平方根相乘时，结果必须是一个根数。它表明结果是一个非平方根数。例如，当 √7 乘以 √7 时，得到的结果是 7。
  • 负数的平方根是未定义的。因此完美平方不能为负。
  • 有些数字以 2、3、7 或 8（在个位）结尾，则不存在完美的平方根。
  • 有些数字的个位数以 1、4、5、6 或 9 结尾，则该数字将有一个平方根。

很容易找到一个完美平方数的平方根。

完美的平方是那些可以写成一个数本身的乘积的正数。或者你可以说一个完美的正方形是一个数字，它是任何整数的 2 次方的值。

可以表示为两个相等整数的乘积的数字。例如， 16 是一个完全平方，因为它是两个相等整数的乘积，4 × 4 = 16。但是，24 不是一个完全平方，因为它不能表示为两个相等整数的乘积。 (8 × 3 = 24)。

如果我们假设 N 是整数 y 的完全平方，这可以写成 N = y 和 y = y 2的乘积。

因此，完美的平方公式可以表示为：

N = Y 2

让我们使用带有值的公式。

如果 y = 5，并且 N = y 2 。

这意味着，N = 5 2 = 25。

在这里，25 是一个完美的平方，因为它是一个整数的平方。

在平方根的帮助下，我们可以确定一个数字是否是一个完美的平方，以及我们是否计算给定数字的平方根。

如果平方根是一个整数，那么给定的数字将是一个完美的平方，如果平方根值不是一个整数，那么给定的数字不是一个完美的平方。

让我们再举一个例子

找到最小的四位数，它是一个完美的正方形

所有整数的平方称为完全平方数。

下面解释寻找最小的四位数字是完美正方形的方法：

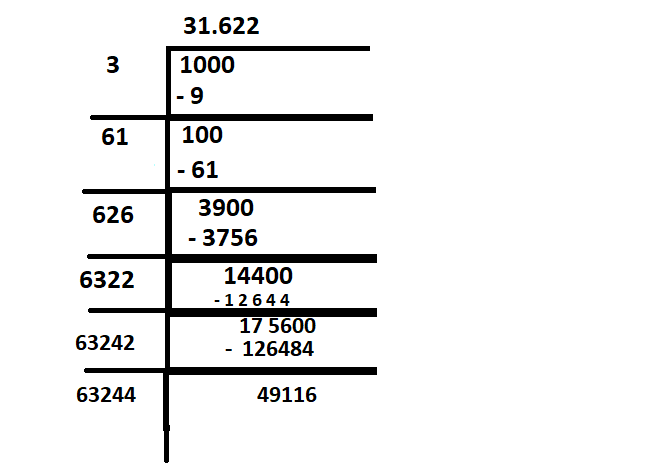
在 1000 的平方根之后，我们很清楚，作为完美平方的最小四位数应该接近 1000。

让我们尝试一些完全平方的数字，

所以这里1000 的平方根是 31.622 并且不是整数，

所以让我们试试32 的平方：

类似问题

问题1：找到具有完美平方的最小三位数？

解决方案：

问题2：找到具有完美平方的最大三位数字？

解决方案：