凹函数

函数图为我们提供了大量关于函数性质、趋势以及函数的最大值和最小值等临界点的信息。导数允许我们对这些函数进行数学分析，它们的符号可以为我们提供有关绘制图形所必需的函数的最大值和最小值的信息。有时仅仅知道一个函数的图形是增加还是减少是不够的，我们还需要查看图形弯曲的方向。方向告诉我们函数是向上凹还是向下凹。让我们看看如何使用衍生品来识别这些趋势。

什么是凹函数？

凹函数也称为凹向下图。直观上，函数的凹度表示函数打开的方向，凹度描述了凹函数的状态或质量。例如，如果函数向上打开，则称为向上凹；如果向下打开，则称为向下凹。下图显示了两个函数，分别是上凹和下凹。

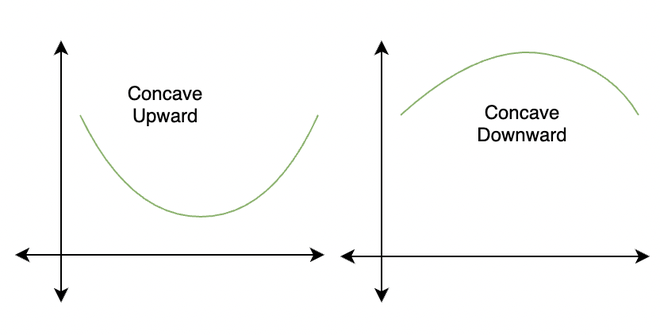
使用图形分析凹函数的凹度

凹度也可以通过在图形上的点处绘制切线来识别。例如，如果在该点绘制到图形的切线位于该点附近的图形下方，则称该图形在该点上凹。类似地，如果绘制到该点的切线位于该点附近的图形上方，则称该图在该点处向下凹。这可以在下图中看到，

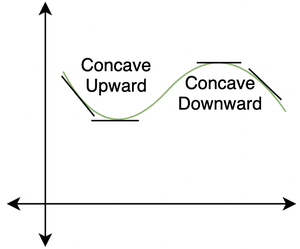
请注意，也可能有一个点，其切线既不位于图形上方，也不位于图形下方。这样的切线在该点与图形相交。此时，凹度从向上变为向下，反之亦然。这个点称为拐点。

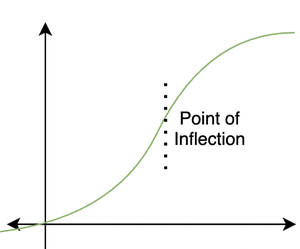
现在让我们看看所有这些点的正式定义，

Let’s say we have a function f(x), 

1. f(x) is said to be concave up in the interval I if all the possible tangents drawn to the curve at different points in the interval I, lie below the graph.
2. f(x) is said to be concave downwards in the interval I if all the possible tangents drawn to the curve at different points in the interval I, lie above the graph.

A point x = a on the curve f(x) is called point of inflection if the function is continuous and the concavity of the graph changes at that point. 

使用导数分析凹函数（凹度）的状态

上述方法是分析函数凹度的图形方法，但有时我们没有函数的图形。当我们对函数的图一无所知时，导数就派上用场了。假设我们有一个函数f(x)。考虑下图中给出的不同情况，我们知道切线的斜率给出了函数在该接触点处的导数的值。

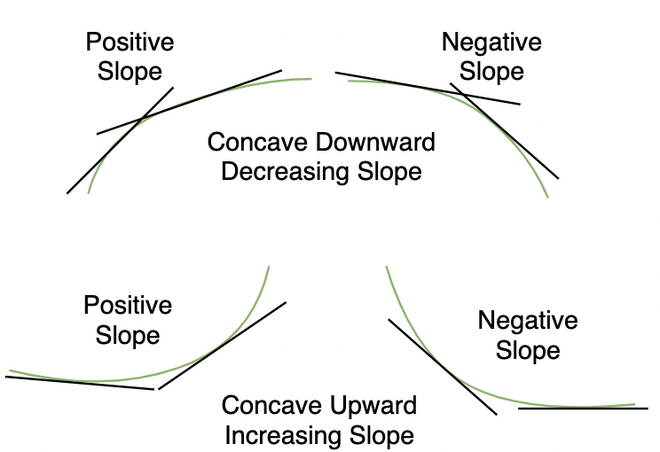
请注意，在上图中，当函数向下凹时，切线的斜率在减小，这意味着导数在减小。类似地，当函数向上凹时，导数的值在减小。因此，我们可以得出以下结论：

1. If the function is concave up, its derivative f'(x) is decreasing.
2. If the function is concave down, its derivative f'(x) is increasing.
3. When the function f(x) has an inflection point at point x = a. f'(x) either goes from increasing to decreasing or vice-versa. That means the graph of the function f'(x) has a minimum/maximum at x = a.

我们可以从上面给出的这些观察中得出数学规则，

Let’s say we have a function f(x), 

1. For the interval I, if f”(x) > 0 then the function f(x) is concave up in the interval I.
2. For the interval I, if f”(x) < 0 then the function f(x) is concave down in the interval I.
3. If x = a is a point of inflection, then at x = a, f”(a) = 0.

示例问题

问题 1：如果函数f(x) = ax ³ + 4x ² + 1 在 x = 1 处向下凹，“a”的值应该是多少。

解决方案：

We need to analyze the functions through the second derivative test explained above, 

f(x) =ax² + 4x + 1 

Differentiating the function, 

⇒ f'(x) = 3ax² + 8x

Differentiating it again to find the second derivative,

⇒ f”(x) = 6ax + 8

At x = 1.

f”(1) = 6a + 8 

For the function to be concave downward, f”(x) < 0

6a + 8 < 0 

⇒ a = $\frac{-4}{3}$

问题 2：函数f(x) = 的图形是什么形状$\frac{1}{x + 4}$在 x = 2。

解决方案：

We need to analyze the functions through the second derivative test explained above, 

f(x) = $\frac{1}{x + 4}$

Differentiating the function, 

⇒ f'(x) = $\frac{-1}{(x + 4)^2}$

Differentiating it again to find the second derivative,

⇒ f”(x) = $\frac{-2}{(x + 4)^3}$

At x = 2. 

f”(x) = $\frac{-2}{(x + 4)^3}$

⇒ f”(x) = $\frac{-2}{(2 + 4)^3}$

f”(2) = $\frac{-2}{(6)^3}$

So, f”(2) < 0. Thus, from the definition above we can say that, the shape of the function is concave downward at x = 2. 

问题 3：函数 f(x) = x 2 + x + 1在 x = 0 处的^图形是什么形状。

解决方案：

We need to analyze the functions through the second derivative test explained above, 

f(x) = x² + x + 1

Differentiating the function, 

⇒ f'(x) = 2x + 1

Differentiating it again to find the second derivative,

⇒ f”(x) = 2

At x = 2. 

f”(0) = 2

So, f”(0) > 0. Thus, from the definition above we can say that, the shape of the function is concave upward at x = 2. 

问题 4：函数 f(x) = x 3 + 4x 2 + 1在 x = 0 处的^图形是什么形状。

解决方案：

We need to analyze the functions through the second derivative test explained above, 

f(x) = x³ + 4x² + 1

Differentiating the function, 

⇒ f'(x) = 3x² + 8x

Differentiating it again to find the second derivative,

⇒ f”(x) = 6x + 8

At x = 0. 

f”(0) = 8

So, f”(0) > 0. Thus, from the definition above we can say that, the shape of the function is concave upward at x = 0. 

问题 5：判断函数f(x) = e ^x + cos(x) 在 x = 0 处是向上凹还是向下凹。

回答：

We need to analyze the functions through the second derivative test explained above, 

f(x) = e^x + cos(x) 

Differentiating the function, 

⇒ f'(x) = e^x – sin(x) 

Differentiating it again to find the second derivative,

⇒ f”(x) = e^x – cos(x) 

At x = 0. 

f”(0) = e⁰ – cos(0) 

⇒f”(0) = 1 – 1

⇒f”(0) = 0 

Since, f”(0) =0. From the definition above we can say that, the function is neither concave up or concave downward at x = 0. So, x = 0 is point of inflection for the function f(x). 

问题 6：绘制函数f(x) = (x-3) ² + 5 的图。

解决方案：

First let’s check for the asymptotic values for the function f(x). 

at x = ∞ and x = -∞ the function f(x) goes towards positive infinity. 

at x = 0 

f(0) = 3² + 5

⇒ f(0) = 14 

Let’s look for the critical points, 

f(x) = (x-3)² + 5

Differentiating f(x) w.r.t. x, 

f'(x) = 2(x – 3) 

Solving, 

f'(x) = 0

⇒ 2(x – 3) = 0 

⇒x = 3

x = 3 is a minima. 

Now let’s see what will be the shape of the graph at x = 3 

f”(x) = 2

f”(x) > 0 Thus the graph will be concave upward. 

Thus, the graph of the function will look like,