以反螺旋形式打印矩阵

给定一个二维数组，任务是以反螺旋形式打印矩阵：
例子：

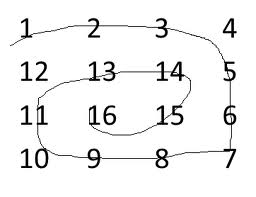
输出：16 15 14 13 12 11 10 9 8 7 6 5 4 3 2 1

Input : arr[][4] = {1, 2, 3, 4
                    5, 6, 7, 8
                    9, 10, 11, 12
                    13, 14, 15, 16};
Output : 10 11 7 6 5 9 13 14 15 16 12 8 4 3 2 1

Input :arr[][6] = {1, 2, 3, 4, 5, 6
                  7, 8, 9, 10, 11, 12
                  13, 14, 15, 16, 17, 18};
Output : 11 10 9 8 7 13 14 15 16 17 18 12 6 5 4 3 2 1

这个想法很简单，我们以螺旋形式遍历矩阵并将所有遍历的元素放入堆栈中。最后从堆栈中逐一打印并打印它们。

C++

// C++ program to print matrix in anti-spiral form
#include 
using namespace std;
#define R 4
#define C 5
 
void antiSpiralTraversal(int m, int n, int a[R][C])
{
    int i, k = 0, l = 0;
 
    /*  k - starting row index
        m - ending row index
        l - starting column index
        n - ending column index
        i - iterator  */
    stack stk;
 
    while (k <= m && l <= n)
    {
        /* Print the first row from the remaining rows */
        for (i = l; i <= n; ++i)
            stk.push(a[k][i]);
        k++;
 
        /* Print the last column from the remaining columns */
        for (i = k; i <= m; ++i)
            stk.push(a[i][n]);
        n--;
 
        /* Print the last row from the remaining rows */
        if ( k <= m)
        {
            for (i = n; i >= l; --i)
                stk.push(a[m][i]);
            m--;
        }
 
        /* Print the first column from the remaining columns */
        if (l <= n)
        {
            for (i = m; i >= k; --i)
                stk.push(a[i][l]);
            l++;
        }
    }
 
    while (!stk.empty())
    {
        cout << stk.top() << " ";
        stk.pop();
    }
}
 
/* Driver program to test above functions */
int main()
{
    int mat[R][C] =
    {
        {1,  2,  3,  4,  5},
        {6,  7,  8,  9,  10},
        {11, 12, 13, 14, 15},
        {16, 17, 18, 19, 20}
    };
 
    antiSpiralTraversal(R-1, C-1, mat);
 
    return 0;
}

Java

// Java Code for Print matrix in antispiral form
import java.util.*;
 
class GFG {
     
    public static void antiSpiralTraversal(int m, int n,
                                             int a[][])
    {
        int i, k = 0, l = 0;
      
        /*  k - starting row index
            m - ending row index
            l - starting column index
            n - ending column index
            i - iterator  */
        Stack stk=new Stack();
      
        while (k <= m && l <= n)
        {
            /* Print the first row from the remaining
             rows */
            for (i = l; i <= n; ++i)
                stk.push(a[k][i]);
            k++;
      
            /* Print the last column from the remaining
            columns */
            for (i = k; i <= m; ++i)
                stk.push(a[i][n]);
            n--;
      
            /* Print the last row from the remaining
            rows */
            if ( k <= m)
            {
                for (i = n; i >= l; --i)
                    stk.push(a[m][i]);
                m--;
            }
      
            /* Print the first column from the remaining
            columns */
            if (l <= n)
            {
                for (i = m; i >= k; --i)
                    stk.push(a[i][l]);
                l++;
            }
        }
      
        while (!stk.empty())
        {
            System.out.print(stk.peek() + " ");
            stk.pop();
        }
    }
     
    /* Driver program to test above function */
    public static void main(String[] args)
    {
         int mat[][] =
                {
                    {1,  2,  3,  4,  5},
                    {6,  7,  8,  9,  10},
                    {11, 12, 13, 14, 15},
                    {16, 17, 18, 19, 20}
                };
              
        antiSpiralTraversal(mat.length - 1, mat[0].length - 1,
                                                       mat);
    }
  }
// This code is contributed by Arnav Kr. Mandal.

Python 3

# Python 3 program to print
# matrix in anti-spiral form
R = 4
C = 5
 
def antiSpiralTraversal(m, n, a):
    k = 0
    l = 0
 
    # k - starting row index
    # m - ending row index
    # l - starting column index
    # n - ending column index
    # i - iterator
    stk = []
 
    while (k <= m and l <= n):
         
        # Print the first row
        # from the remaining rows
        for i in range(l, n + 1):
            stk.append(a[k][i])
        k += 1
 
        # Print the last column
        # from the remaining columns
        for i in range(k, m + 1):
            stk.append(a[i][n])
        n -= 1
 
        # Print the last row
        # from the remaining rows
        if ( k <= m):
            for i in range(n, l - 1, -1):
                stk.append(a[m][i])
            m -= 1
 
        # Print the first column
        # from the remaining columns
        if (l <= n):
            for i in range(m, k - 1, -1):
                stk.append(a[i][l])
            l += 1
         
    while len(stk) != 0:
        print(str(stk[-1]), end = " ")
        stk.pop()
 
# Driver Code
mat = [[1, 2, 3, 4, 5],
       [6, 7, 8, 9, 10],
       [11, 12, 13, 14, 15],
       [16, 17, 18, 19, 20]];
 
antiSpiralTraversal(R - 1, C - 1, mat)
 
# This code is contributed
# by ChitraNayal

C#

using System;
using System.Collections.Generic;
 
// C# Code for Print matrix in antispiral form
 
public class GFG
{
 
    public static void antiSpiralTraversal(int m, int n, int[][] a)
    {
        int i, k = 0, l = 0;
 
        /*  k - starting row index
            m - ending row index
            l - starting column index
            n - ending column index
            i - iterator  */
        Stack stk = new Stack();
 
        while (k <= m && l <= n)
        {
            /* Print the first row from the remaining 
             rows */
            for (i = l; i <= n; ++i)
            {
                stk.Push(a[k][i]);
            }
            k++;
 
            /* Print the last column from the remaining
            columns */
            for (i = k; i <= m; ++i)
            {
                stk.Push(a[i][n]);
            }
            n--;
 
            /* Print the last row from the remaining 
            rows */
            if (k <= m)
            {
                for (i = n; i >= l; --i)
                {
                    stk.Push(a[m][i]);
                }
                m--;
            }
 
            /* Print the first column from the remaining 
            columns */
            if (l <= n)
            {
                for (i = m; i >= k; --i)
                {
                    stk.Push(a[i][l]);
                }
                l++;
            }
        }
 
        while (stk.Count > 0)
        {
            Console.Write(stk.Peek() + " ");
            stk.Pop();
        }
    }
 
    /* Driver program to test above function */
    public static void Main(string[] args)
    {
         int[][] mat = new int[][]
         {
             new int[] {1, 2, 3, 4, 5},
             new int[] {6, 7, 8, 9, 10},
             new int[] {11, 12, 13, 14, 15},
             new int[] {16, 17, 18, 19, 20}
         };
 
        antiSpiralTraversal(mat.Length - 1, mat[0].Length - 1, mat);
    }
}
 
// This code is contributed by Shrikant13

Javascript

 
// Javascript Code for Print matrix in antispiral form
     
    function antiSpiralTraversal(m,n,a)
    {
        let i, k = 0, l = 0;
         
        /*  k - starting row index
            m - ending row index
            l - starting column index
            n - ending column index
            i - iterator  */
        let stk=[];
        
        while (k <= m && l <= n)
        {
            /* Print the first row from the remaining
             rows */
            for (i = l; i <= n; ++i)
                stk.push(a[k][i]);
            k++;
        
            /* Print the last column from the remaining
            columns */
            for (i = k; i <= m; ++i)
                stk.push(a[i][n]);
            n--;
        
            /* Print the last row from the remaining
            rows */
            if ( k <= m)
            {
                for (i = n; i >= l; --i)
                    stk.push(a[m][i]);
                m--;
            }
        
            /* Print the first column from the remaining
            columns */
            if (l <= n)
            {
                for (i = m; i >= k; --i)
                    stk.push(a[i][l]);
                l++;
            }
        }
        
        while (stk.length!=0)
        {
            document.write(stk[stk.length-1] + " ");
            stk.pop();
        }
     
    }
     
    /* Driver program to test above function */
    let mat = [[1, 2, 3, 4, 5],
       [6, 7, 8, 9, 10],
       [11, 12, 13, 14, 15],
       [16, 17, 18, 19, 20]];
    antiSpiralTraversal(mat.length - 1, mat[0].length - 1,
                                                       mat);
     
    // This code is contributed by avanitrachhadiya2155

输出：

12 13 14 9 8 7 6 11 16 17 18 19 20 15 10 5 4 3 2 1 

• 矩阵的对角线遍历
• 以螺旋形式打印矩阵
• 以之字形形式打印给定的矩阵