📌  相关文章
📜  等电位面

📅  最后修改于: 2022-05-13 01:57:38.482000             🧑  作者: Mango

等电位面

当外力做功时，将物体从一个点移动到另一个点，以抵抗弹簧力或重力等力，该功被收集或存储为物体的势能。当排除外力时，身体移动，获得动能并失去等量的势能。因此动能和势能之和是守恒的。这类力量被称为保守力量。这些力的例子是弹簧力和重力。

等电位面

在表面上所有位置具有固定电位值的表面称为等电位面。对于单个电荷 q，电势可以表示为

V=\frac{1}{4\pi\epsilon_0}\frac{q}{r}

在上面的表达式中，观察到如果 r 是常数，那么 V 也保持常数。因此，单点电荷的等势面是以电荷为中心的同心球面。

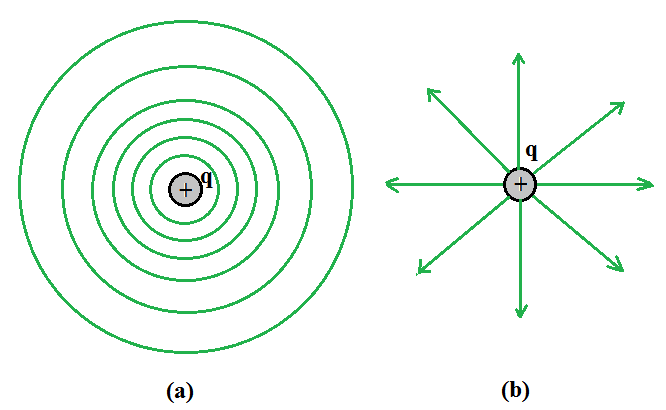
对于单个电荷 q(a) 等势面是以电荷为中心的球面，并且 (b) 电场线是径向的，如果 q > 0 从电荷开始。

根据 q 是正还是负，单个电荷 q 的电场线是在电荷处开始或结束的径向线。每个地方的电场明显垂直于通过该点的等势面。对于任何电荷排列，通过一点的等势面垂直于该位置的电场。这个断言的证明是直截了当的。

如果场不垂直于等势面，则该场沿该表面具有非零分量。将需要工作以将单元测试电荷向与场的组成部分相反的方向移动。然而，这与等势表面的定义相矛盾，该定义表明表面上任何两个位置之间都没有电位差，并且不需要做任何工作来将测试电荷移动到其上。因此，在所有点上，电场都必须垂直于等势面。除了电荷排列周围的电场线图像之外，等电位表面还允许另一种视觉图像。

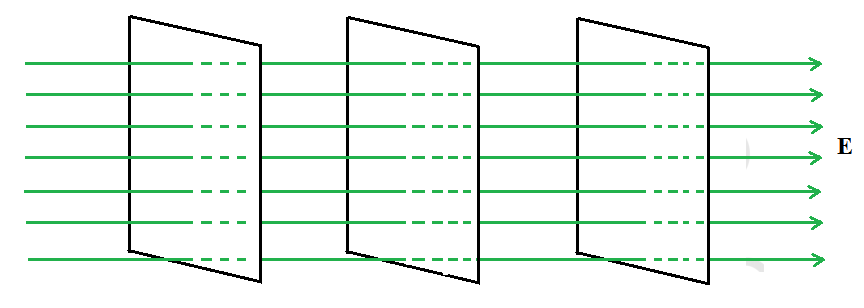
均匀电场的等势面。

对于均匀电场 E，比如说，沿 x 轴，等势面是垂直于 x 轴的平面，即平行于 yz 平面的平面，如上图所示。

(a) 一个偶极子，(b) 两个相同的正电荷的一些等势面。

上图是 (a) 偶极子的等势面和 (b) 具有两个相同正电荷的等势面。

在等电位面上做功

在等势面上的两个位置之间移动电荷始终为零。在等势面上，如果点电荷从具有势能 V A 的 A点传输到具有势能 V B的 B 点，则移动电荷所做的功为

W = q(V A –V B ) = 0

因为 V A – V B = 0，

完成的总功 W 为 0。

等电位面的性质

  • 等势面具有始终垂直于它的电场。
  • 两个等势面不可能相交。
  • 点电荷的等势面是同心球壳。
  • 对于均匀电场，等势面是垂直于 x 轴的平面。
  • 等电位面从高电位指向低电位。
  • 空心带电球形导体内部的电势是恒定的。等电位体积可用于此。将电荷从中心移动到表面不需要做任何工作。
  • 孤立点电荷的等势面是一个球体。点电荷周围存在不同的等势面，即同心球。
  • 任何垂直于均匀场方向的平面都是等势面。
  • 等势面之间的距离使我们能够区分强场和弱场。

电位

当物体逆着电场运动时，它会获得称为电势能的能量。将势能除以电荷量即可得到电荷的电势。电场的强度由电势决定。这与是否应将电荷置于电场中无关。电势是一个标量。在点电荷 +q 处，距离为 r 的所有点具有相同的电位。

物体的电势由以下因素决定：

  • 一个电荷。
  • 带电物体相对于其他带电物体的位置。

点电荷产生的电势

考虑点电荷 Q 的起源。取 Q 为正。使用从原点开始的位置矢量 r，我们想要找到任意点 P 的电势。为此，我们必须计算将一个单位正测试电荷从无穷远传输到点 P 所需的工作量。当 Q > 0 时，对排斥力的测试电荷所做的工作是正的。我们选择一条方便的路径——沿着从无穷远到 P 点的径向——因为完成的工作与路径无关。

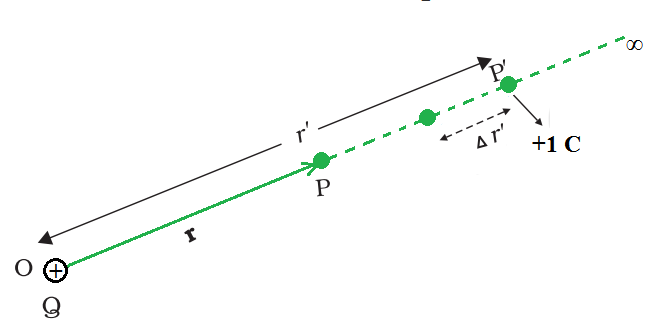
将一个单位正测试电荷从无穷远处带到 P 点，抵抗电荷排斥力 Q (Q > 0) 所做的功是电荷 Q 在 P 处的电势。

在路径上某个中间点 P' 处单位正电荷上的静电力等于

\frac{Q\times1}{4\pi\epsilon_0r'^2}\hat{r'}

在哪里 ' \hat{r'} } 是沿 OP' 的单位向量，因此，从 r' 到 r' + Δr' 对这个力所做的功可以写成

\Delta{W}=-\frac{Q}{4\pi\epsilon_0r'^2}\Delta{r'}

负号表示 Δr′ < 0，ΔW 为正。外力完成的总功 (W) 由上述方程两边积分确定，从 r' = ∞ 到 r' = r，

W=-\int_{∞}^{r} \frac{Q}{4\pi\epsilon_0r'^2}d{r'}\\ W=\left[\frac{Q}{4\pi\epsilon_0r'}\right]_∞^r\\ W=\frac{Q}{4\pi\epsilon_0r}

由于电荷 Q 在 P 处的电位可以表示为

V(r)=\frac{Q}{4\pi\epsilon_0r}

示例问题

问题 1：计算点 P 处 9 cm 外的 4 × 10 –7 C 电荷所产生的电势。

解决方案：

问题 2：获得将 2 × 10 –9 C 的电荷从无穷远处带到 P 点所做的功。答案是否取决于电荷被带入的路径？ (V = 4 × 10 4 V)

解决方案：

问题 3：确定由分别位于 (–9 cm, 0, 0) 和 (9 cm, 0, 0) 的两个电荷 7 µC 和 –2 µC（并且没有外场）组成的系统的静电势能。

解决方案：

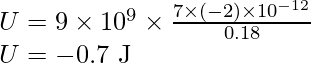
问题 4：6 物质分子的永久电偶极矩为 10 –29 C m。通过施加大小为 10 6 V m –1的强静电场，一摩尔这种物质会被极化（在低温下）。场的方向突然改变了 60º 的角度。估计物质在将其偶极子沿场的新方向对齐时释放的热量。为简单起见，假设样品 100% 极化。

解决方案：

问题 5：写出等势面的性质。

解决方案：