假设一个点将一条线段分为两个相等或不相等的部分,则在截面公式的帮助下,如果给出了线段的坐标,我们可以找到该点,并且还可以找到该点将线段的比例除以给定的线段(如果已给出该点的坐标)。
当点C以m:n的比例划分线段AB时,我们使用截面公式来找到该点的坐标。该部分公式有2种类型。这些类型取决于点C,该点C可以出现在点之间或线段之外。
这两种类型是:
- 内部截面公式
- 外部截面公式
内部截面公式
当点在点C内部以m:n的比例将线段划分时,该点位于线段的坐标之间,那么我们可以使用此公式。也称为内部部门。
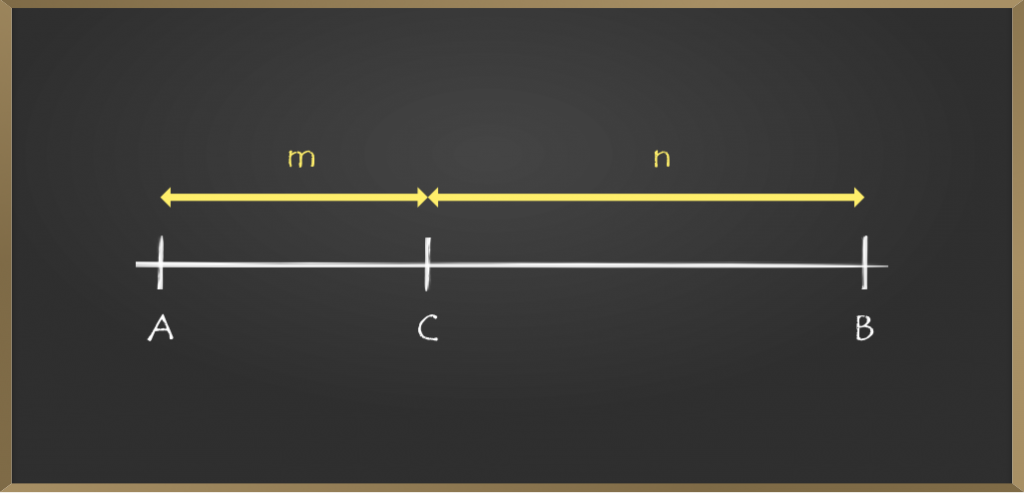
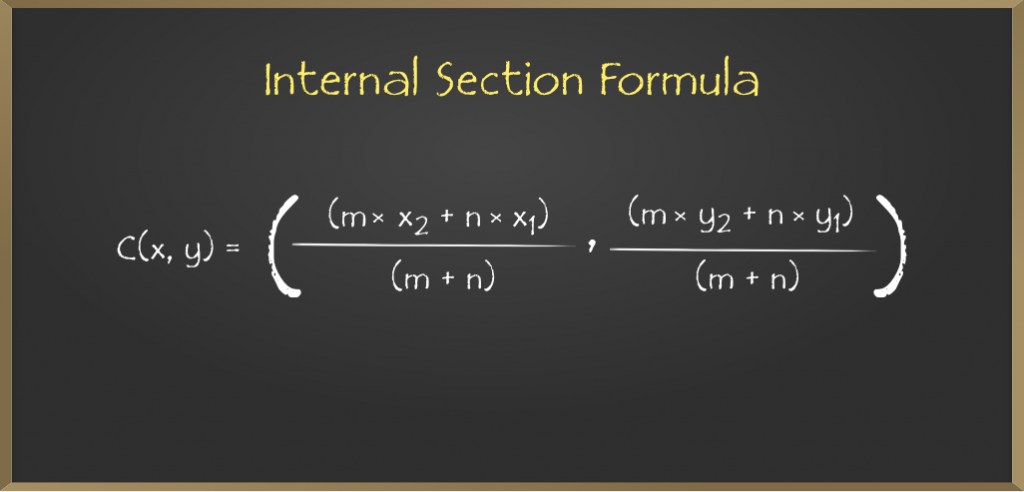
如果A和B的坐标分别为(x1,y1)和(x2,y2),则内部截面公式为:
公式的推导
设A(x1,y1)和B(x2,y2)是给定线段AB的端点,而C(x,y)是将AB以m:n的比例划分的点。
然后, AC / CB = m / n
我们想找到C的坐标(x,y)。
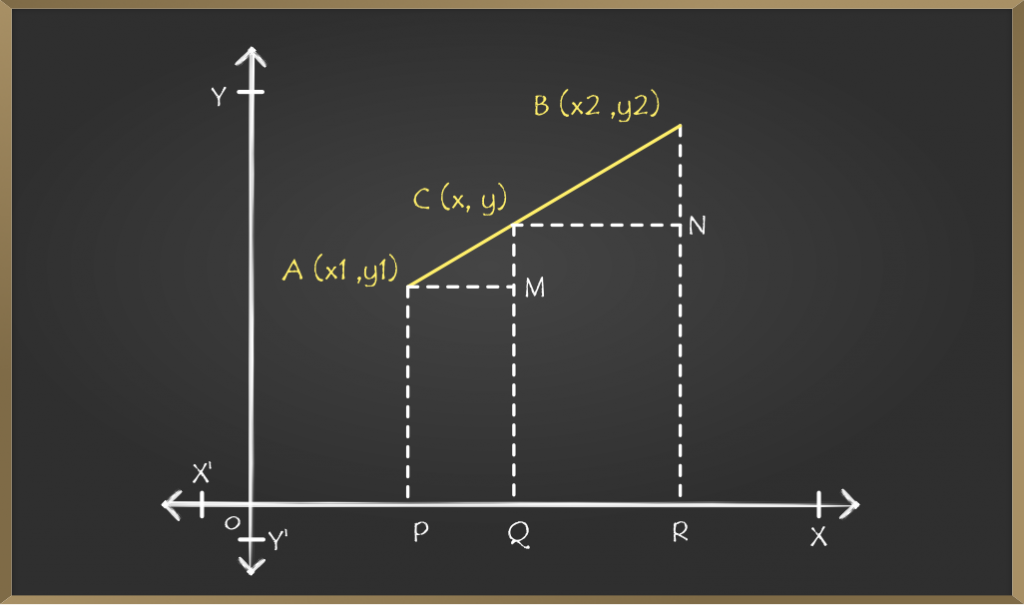
Now draw perpendiculars of A, C, B parallel to Y coordinate joining at P, Q, and R on X-axis.
By seeing the above diagram,
AM = PQ = OQ – OP = (x – x1)
CN = QR = OR – OQ = (x2 – x)
CM = CQ – MQ = (y – y1)
BN = BR – NR = (y2 – y)
Clearly, we can see that ∆AMC and ∆CNB are similar and, therefore, their sides are proportional by AA congruence rule.
AC / CB = AM / CN = CM / BN
Now substituting the values in the above relation
=> m / n = [x – x1 / x2 -x] = [y – y1 / y2 – y]
=> m / n = [x – x1 / x2 -x] and m / n = [y – y1 / y2 – y]
Solving the 1st condition,
=> m(x2 – x) = n(x – x1)
=> (m + n)x = (mx2 + nx1)
=> x = (mx2 + nx1) / (m + n)
Solving the 1st condition,
=> m(y2 – y) = n(y – y1)
=> (m + n)y = (my2 + ny1)
=> y = (my2 + ny1) / (m + n)
因此,C(x,y)的坐标为
{(m×x 2 + n×x 1 )/(m + n),(m×y 2 + n×y 1 )/(m + n)}
外部截面公式
当将线段划分的点以m:n的比例从外部进行划分时,即,当我们扩展线时,它与该点重合,则可以使用此公式。也称为外部部门。
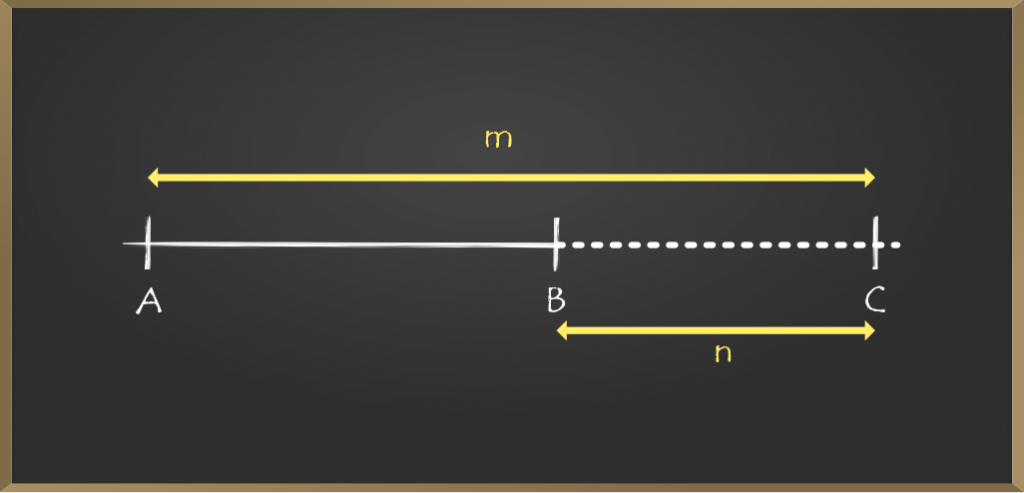
如果A和B的坐标分别为(x1,y1)和(x2,y2),则外部截面公式为
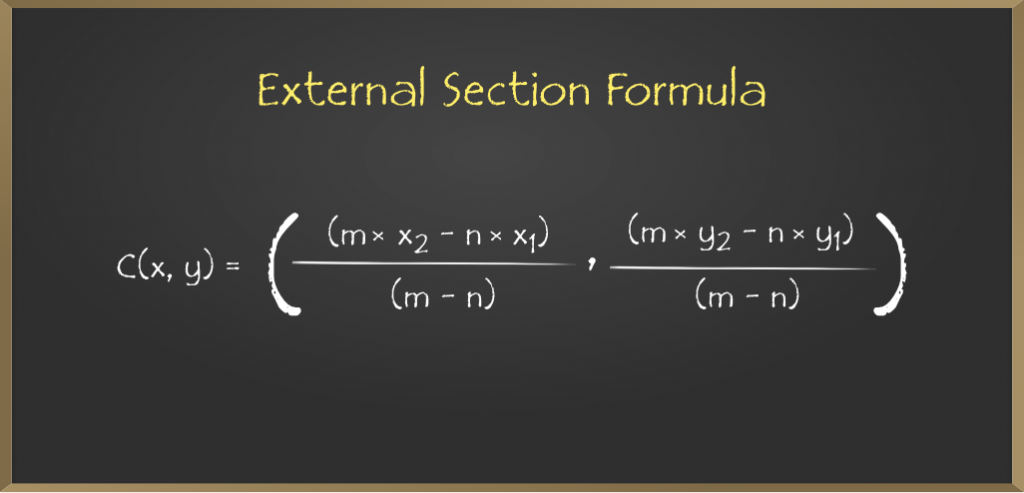
公式的推导
为了得出内部截面,我们采用了线段和线内的点C(x,y),但是对于外部截面公式,我们必须采用线段外的点C(x,y)。
假设A(x1,y1)和B(x2,y2)是给定线段AB的端点,而C(x,y)是将AB按外部比率m:n划分的点。
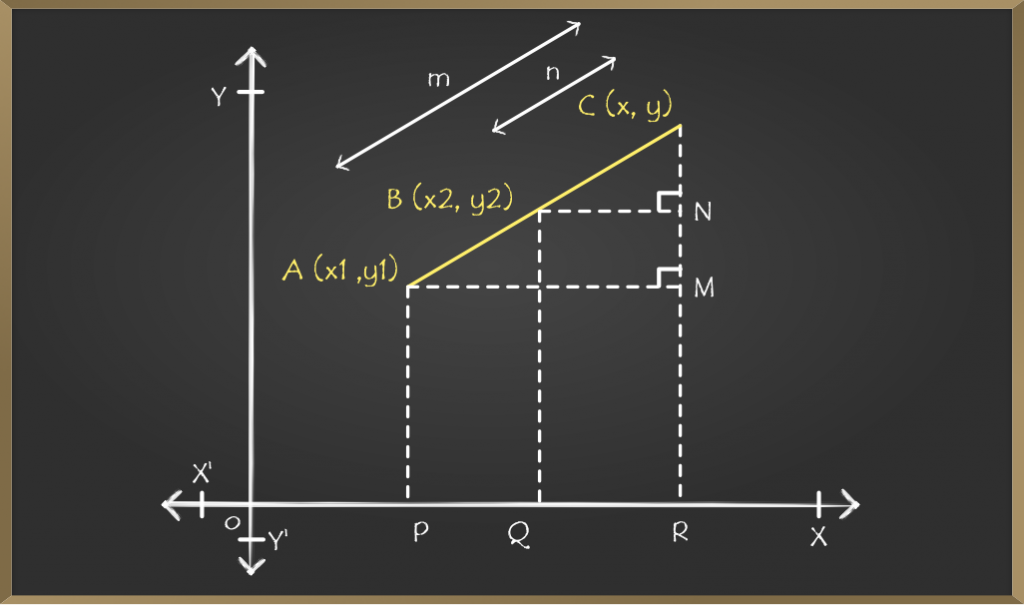
We want to find the coordinates (x, y) of C. For that, draw perpendiculars of A, B, C parallel to Y coordinate joining at P, Q, and R on X axis.
By seeing the above diagram,
AM = PR = OR – OP = (x – x1)
BN = QR = OR – OQ = (x – x2)
similarly,
CM = RC – MR = (y – y1)
CN = CR – NR = (y – y2)
Clearly, we can see that triangle AMC and triangle BNC are similar and, therefore, their sides are proportional by AA congruence rule
AC / BC = AM / BN = CM / CN
Now substituting the values in the above relation
=> m / n = [x – x1 / x – x2] = [y – y1 / y – y2]
=> m / n = [x – x1 / x – x2] and m / n = [y – y1 / y – y2]
Solving the 1st condition,
=> m(x – x2) = n(x – x1)
=> (m – n)x = (mx2 – nx1)
=> x = (mx2 – nx1) / (m – n)
Solving the 2nd condition,
=> m(y – y2) = n(y – y1)
=> (m – n)y = (my2 – ny1)
=> y = (my2 – ny1) / (m – n)
因此,C(x,y)的坐标为
{(m×x 2 – n×x 1 )/(m – n),(m×y 2 – n×y 1 )/(m – n)}
关于截面公式的问题
问题1:找到点C(x,y)的坐标,该点在内部将连接(4,– 1)和(4,3)的线段以3:1的比率划分?
解决方案:

Given coordinates are A (4, -3) and B (8, 5)
Let C (x, y) be a point which divides the line segment in the ratio of 3 : 1 i.e m : n = 3 : 1
Now using the formula C(x, y) = { (m × x2 + n × x1) / (m + n ) , (m × y2 + n × y1) / (m + n ) } as C is dividing internally.
=> C(x, y) = {(3*4 + 1*4 ) / (3+1), (3 * 3 + 1 *(-1)) / (3+1)}
=> C(x, y) = {16 / 4, 8 / 4}
=> C(x, y) = {4, 2}
Hence, the coordinates are (4, 2).
问题2:如果点P(k,7)将连接A(8,9)和B(1,2)的线段按m:n的比例进行划分,则找到m和n的值。
解决方案:
没有提到该点在内部或外部划分线段。因此,那时我们将内部部分视为默认部分。
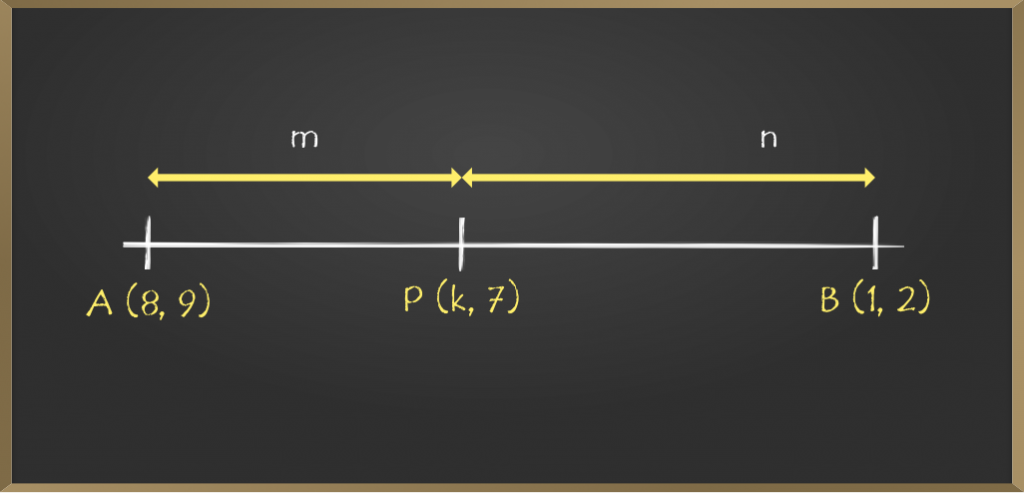
Given coordinates are A (8, 9) and B (1, 2)
Let the given point P (k, 7) divides the line segment in the ratio of m : 1
Now using the section formula, finding only the x coordinate,
=> k = (m × x2 + n × x1) / (m + n )
=> k = (m × 1 + 1 × 8) / (m +1)
=> k = (m + 8) / (m + 1)
=> km + k = m + 8 …….(1)
Again using section formula for y coordinate.
=> 7 = (m × y2 + n × y1) / (m + n )
=> 7 = (m × 2 + 1 × 9) / (m + 1)
=> 7 = (2m + 9) / (m +1)
=> 7m + 7 = 2m +9
=> 5m = 2
=> m = 5 / 2
So the required ratio is 5 : 2
Therefore, value of m is 5 and value of n is 2
问题3: A(4,5)和B(7,-1)是两个给定的点,并且点C在外部以4:3的比例将线段AB分开。找到C的坐标。
解决方案:
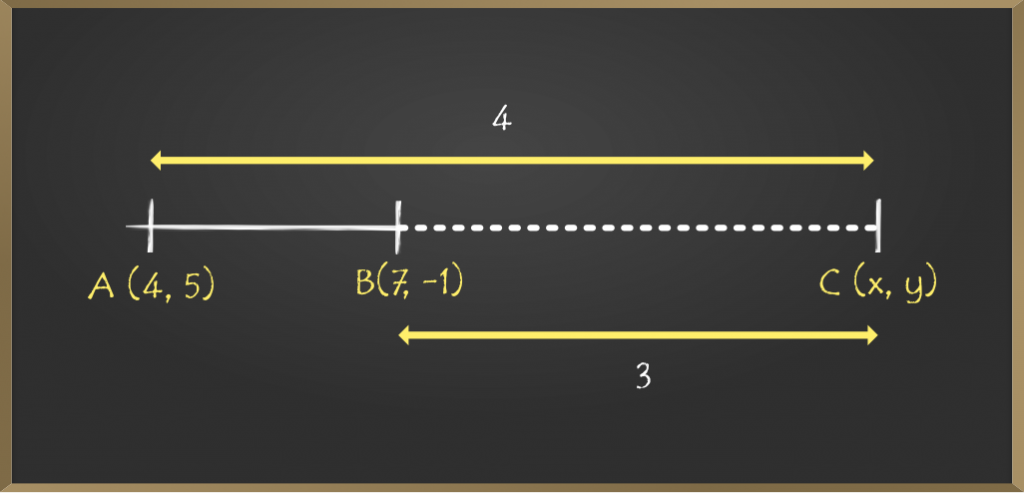
Given coordinates are A (4, 5) and B (7, -1)
Let C (x, y) be a point which divides the line segment externally in the ratio of 4 : 3 i.e m : n = 4 : 3
Now using the formula C(x, y) = { (m × x2 – n × x1) / (m – n) , (m × y2 – n × y1) / (m – n ) } as C is dividing internally.
value of x = (mx2 – nx1) / (m – n)
=> (4 * 7 – 3 * 4) / (4 – 3)
=> 16
value of y = (my2 – ny1) / (m – n )
=> (4 * (-1) – 3 * 5) / (4 – 3)
=> -19
Hence, the coordinates are (16, -19).
问题4:线2x + y-4 = 0划分了连接点A(2,-2)和B(3,7)的线段。找出线被分割的线段的比例?
解决方案:
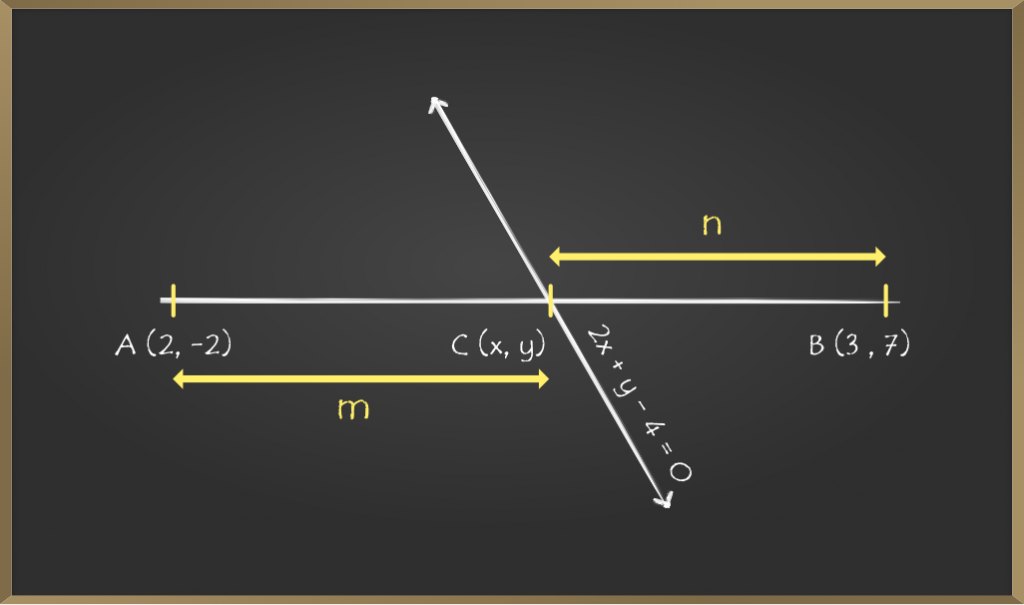
Given coordinates are A (2, -2) and B (3, 7).
Line with equation 2x + y – 4 = 0 divides the line segment at point C (x, y)
Let us assume the given line cuts the line segment in the ratio 1 : n.
By section formula,
=> x = (m * x2 + n * x1) / (m + n)
=> x = (3 + 2n) / (1 + n) ………..1
Similarly,
=> y = (m * y2 + n * y1) / (m + n)
=> y = (7 – 2n) / (1 + n) ……….2
Now substituting the equations 1 and 2 in the given equation of the line.
=> 2x + y – 4 = 0
=> 2 [(3 + 2n) / (1 + n) ] + [(7 – 2n) / (1 + n)] – 4 = 0
=> 6 + 4n + 7 − 2n − 4(1 + n) = 0
=>13 + 2n − 4 − 4n = 0
=>9 − 2n = 0
=> n = 2 / 9
Therefore the ratio at which the line divides is 9 : 2. We can also find the values of x and y by substituting the value of n in the equation 1 and 2.
问题5: A(2,7)和B(–4,–8)是线段AB的坐标。有两点将线段一分为三。查找它们的坐标。

解决方案:
The two points trisected the line segment, which means the segment is divided into 3 equal parts.
AS = ST = TB …………1
=> AS / SB
=> AS / ST + TB
=> AS / (AS + AS) from equation 1
=> AS / 2 AS
=> 1 / 2
So, S divides the line segment AB in the ratio of 1 : 2
Now applying section formula to find the coordinates of point S
=> x1 = (1 × (-4) + 2 × 2) / (1 + 2)
=> x1 = (-4 + 4) / 3
=> x1 = 0
Similarly, for y coordinate,
=> y1 = (1 × (-8) + 2 × 7) / (1 + 2)
=> y1 = (14 – 8) / 3
=> y1 = 2
Also.
=> AT / TB
=> (AS +ST) / TB
=> 2 TB / TB from equation 1
=> 2 / 1
So, T divides the line segment AB in the ratio of 2 : 1
Now applying section formula to find the coordinates of point T
=> x2 = (2 × (-4) + 1 × 2) / (2 + 1)
=> x2 = (-8 + 2) / 3
=> x2 = -2
Similarly, for y coordinate
=> y2 = (2 × (-8) + 1 × 7) / (2 + 1)
=> y2 = (-16 + 7) / 3
=> y2 = -3
Thus, the coordinates are :
S (x1, y1) = (0, 2)
T (x2, y2) = (-2, -3)