二次方程的根

二次方程式是多项式为二阶的方程式。二次方程式是ax ² + bx + c = 0的方程式，其中x是未知的，而a，b，c是已知的实数，并且a不应为零。如果a = 0，则方程将不会保持二次方程，则将变为线性，因为a = 0将消除x ²项。由于二次方程具有最高的二阶，因此该方程具有两个根，或者可以说我们将为二次方程找到两个x值。

如何找到根?

方法1：可以通过Shridharacharaya公式找到二次方程式的根。

x = [-b±√(b ² – 4ac)] / 2a

示例：矩形的边长由x – 3和x – 5给出，矩形的面积为3 unit ² 。找到矩形的边。

解决方案：

Area of rectangle = length*breadth = (x – 3)(x – 5) = 3

Area = x² – 8x + 15 = 3

        = x² – 8x + 12 = 0

Discriminant = b² – 4ac = 64 – (4(1)(12)) = 64 – 48 = 16

 x = [-b ± √(b²-4ac)]/2a = [-(-8) ± √16]/2 = (8±4)/2

 x = 12/2 or 4/2

x = 2 or 6

When x is 2, sides are x – 3 = 2 – 3 = -1 and x – 5 = 2 – 5 = -3.

Since length of sides cannot be equal therefore x = 2 is not a valid ans.

When x is 6, sides are x – 3 = 6 – 3 = 3 and x – 5= 6 – 5 =1.

Therefore, x = 6 is the valid answer and the sides are 3 and 1.

方法2。另一种方法是分解方法。二次方程式可以认为是两个项的因数。像ax ² + bx + c = 0可以写成(x – x ₁ )(x – x ₂ )= 0，其中x ₁和x ₂是二次方程的根。

脚步：

1. 找出两个数字，使得乘积= ac且和= b。
2. 然后将x系数写为这两个数字的总和，并将它们除以得到x的两个项。
3. 将前两个项作为一个组，将后两个项作为一个组。
4. 从这些中取公因数，并在取公因数并重新排列方程后，将两个表达式等于0，得到了根。

例子：设二次方程x ² + 3x = 18

x² + 3x – 18 = 0

Step:

1. 6 and -3 are the numbers whose sum is equal to b and product is equal ac.

2. x² + (6-3)x – 18 = x² + 6x -3x – 18 =0  

3. x(x + 6) – x(x + 6) = 0

4. taking (x + 6) as common. 

(x + 6)(x – 3) = 0

x = -6 or x = 3

在分解方法中，不必总是容易找到这两个数字(尤其是在根是虚数或无理数的情况下)，因此最好使用二次公式。

根的性质

根的性质取决于二次方程的判别式。二次方程的判别式由b ² – 4ac给出。之所以这样，是因为在二次方程式中存在判别式的平方根。

根1：如果b ² – 4ac> 0，则根是真实且不同的。由于判别式> 0，因此它的平方根将不会是虚的。它有两种情况。

• 如果b ² – 4ac是一个完美的平方，则根是有理的。由于判别式是一个完美的平方，因此我们将有一个整数作为判别式的平方根。因此，根是有理数。

示例：令二次方程为x ² -5x + 6 = 0。

Then the discriminant of the given equation is b² – 4ac=(-5)² – 4*1*6 = 25-24 = 1

According to Shridharacharaya formula

   x = [-b±√(b²-4ac)]/2a = x = [-(-5) ± √1]/2 

   x₁ = [-(-5) + √1]/2 = 6/2 = 3

   x₂ = [-(-5) – √1]/2 = 4/2 = 2

   Therefore, the roots are 3,2. Both are rational and different.

• 如果B ² – 4AC不是一个完美的正方形然后判别的平方根是不合理的，因此根是不合理的，发生在对。

示例：令二次方程为x ² -7x + 8 = 0。

Then the discriminant of the given equation is

b² – 4ac=(-7)² – 4*1*8 = 49-32 = 17

According to Shridharacharaya formula

x = [-b±√(b²-4ac)]/2a =  x = [-(-7) ± √17]/2 

x₁ = [-(-7) + √17]/2 = [7 + √17]/2

x₂ = [-(-7) – √17]/2 = [7 – √17]/2

Therefore, the roots are [7 + √17]/2,[7 – √17]/2. Both are irrational and in pairs.

根2：如果b ² – 4ac = 0，则根是真实且相等的。

示例：令二次方程为3x ² -6x + 3 = 0。

Then the discriminant of the given equation is

b² – 4ac=(-6)² – 4*3*3 = 36 – 36 = 0

According to Shridharacharaya formula

x = [-b±√(b²-4ac)]/2a =  x = [-(-6) ± √0]/[(2)(3)]

x₁ = [-(-6) + √0]/2 = 6/6 = 1

x₂ = [-(-6) – √0]/2 = 6/6 = 1

Therefore, the roots are 1,1. Both are real and equal.

根3：如果b ² – 4ac <0根是假想的，或者您可以说复数根。这是虚构的，因为平方根下的项为负。这些复杂的根将始终成对出现，即两个根彼此共轭。

示例：令二次方程为x ² + 6x + 11 = 0。

Then the discriminant of the given equation is

b² – 4ac=(6)² – 4*1*11 = 36-44 = -8

According to Shridharacharaya formula

x = [-b±√(b²-4ac)]/2a = x = [-(6) ± √(-8)]/2 

x₁ = [-(6) + √(-8)]/2 = [-6 + √8i]/2 = 2[-3 + √2i]/2 = -3 + √2i

x₂ = [-(6) – √(-8)]/2 = [-6 – √8i]/2 = 2[-3 – √2i]/2 = -3 – √2i

Therefore, the roots are 3,2. Both are imaginary and conjugate of each other(in pair).

根图

在x = -b / 2a处发现二次函数的最大值/最小值

证明：

当d(f(x))/ dx = 0时，我们得到最大值或最小值。

关于微分二次函数f(x)= ax ² + bx + c。

我们得到

2ax + b = 0

x = -b / 2a

x是最大值(a <0)或最小值(a> 0)。

1.当b ² – 4ac> 0时

2.当b ² – 4ac = 0时

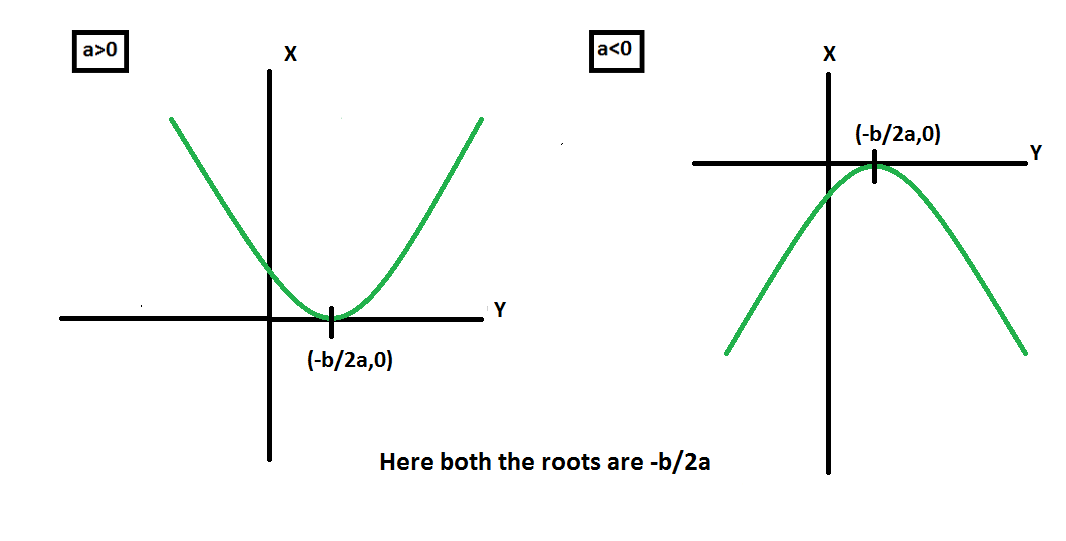
3.当b ² – 4ac <0时

二次方程词问题

问题1.三角形的高度比底边小4厘米。三角形的面积为30 cm ² 。找到三角形的高度和底边。

解决方案：

Let the base of triangle be x cm then height is x-4 cm 

Area of triangle = 1/2*height*base = 1/2*(x)(x – 4)=30

Area = x² – 4x  = 30*2

        = x² – 4x = 60

        = x² – 4x – 60 = 0

Discriminant = (-4)² – 4(1)(-60) = 16+240 = 256

x = [-b±√(b² – 4ac)]/2a = [-(-4)±√256]/2 = (4±16)/2

x = 20/2 or -12/2

x = 10 or -6

As side cannot be negative, therefore -6 is not correct.

So, when x is 10, base =10 cm and height =x – 4 = 10 – 4 = 6 cm

Therefore, x = 10 is the valid answer and the base and height of the triangle are 10 and 6 respectively.

问题2.盒子的体积是600英寸² 。盒子的长度比宽度小2英寸。盒子的高度是5英寸。找到盒子的尺寸。

解决方案：

Let the width of box be x inches then length = x – 2 inches.

Volume of box =Length* Width *Height = (x-2)(x)5= 600

x² – 2x = 120 => x² – 2x -120 = 0

x² + 10x – 12x – 120 = 0

x(x + 10) – 12(x + 10)=0

(x – 12)(x + 10)=0

x = 12 or x = -10

As width can not be negative, therefore, -10 is not correct.

When x = 12, width = 12 inches, length = x – 2 = 12 – 2 = 10 inches, height = 5 inches 

问题3.从建筑物的顶部扔一个球。其在地下作为时间的函数米以上的高度为h(T)= -4t ² +24吨+ 3 a)有多少时间达到最大高度，什么是最大高度给定。 b)还找出球击中地面的时间。

解决方案：

a) Since a<0, therefore the time to reach maximum height is = -b/2a. (refer graph part)

t = -24/(2(-4)) = -24/-8

t = 3 sec.

Height = h(t) = -4(3)² +24(3)+3 = 39 meters.

b) When the ball hits the ground h(t)=0.

-4t² + 24t + 3 = 0

The discriminant of the given equation is

b² – 4ac=(24)² – 4*(-4)*3 = 576 + 48 = 624

According to Shridharacharaya formula

x = [-b±√(b²-4ac)]/2a = x = [-(24) ± √624]/[(2)(-4)]

x₁ = [-24 + √624]/-8= 4 [-6 + √39]/-8 = [-6 + √39]/-2 = [6 – √39]/2 = -0.122499 = -0.1225(approx)

x₂ = [-24 – √624]/-8= 4 [-6 – √39]/-8 = [-6 – √39]/-2 = [6 + √39]/2 = 6.122499 = 6.1225(approx)

As time can not be negative, therefore, [6 – √39]/2 sec is not correct.

Therefore, the ball hits the ground at [6 + √39]/2 = 6.122499 = 6.1225 sec