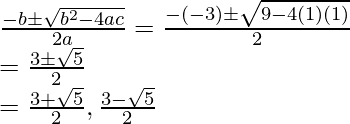二次方程

多项式具有不同的类型，其中两个多项式的形式为ax ² + bx + c，a≠0。当将此多项式等于0时，我们得到一个二次方程。在许多现实生活中都会出现这类方程式。让我们研究这些方程式，如何求解它们以及如何在现实生活中公式化它们。

例如，一个校务委员会决定为他们的学生做一个足球场。他们决定将足球场的面积定为1000m ² 。教练指示地面长度必须比地面宽度的两倍长50米。我们需要找到地面的长度和宽度。

假设地面的宽度为“ x”。则地面的长度将为“ 2x + 50”。我们知道面积必须为1000m ² 。所以，

x(2x + 50)= 1000

⇒2x ² + 50x = 1000

⇒2x ² + 50x – 1000 = 0

在这里，我们得到一个二次方程。让我们正式定义二次方程。

二次方程

变量“ x”中的二次方程是形式为等式的方程，

斧² +斧+ c = 0

其中，a，b，c是实数和常数，且a≠0。

例如： 4x ² – 5x = 0，5x ² + 16x + 5 = 0

In general, any second-degree polynomial P(x), when put like P(x) = 0 represents a quadratic equation. 

问题1：Rahul和Ravi共有45个糖果。他们俩各损失了5个糖果。现在，他们两个人拥有的糖果数的乘积为124。我们被要求找出一开始每个人拥有的糖果数。为这个问题制定二次方程。

解决方案：

Let’s say Rahul had “x” candies. Then Ravi must have “45 – x” candies because both of them had 45 candies. 

Now after losing the candies, we are given that product of the number of candies they have is 124. That is, 

x(45 – x) = 124

⇒ 45x – x² = 124 

⇒ 0 = x² – 45x + 124

问题2：检查以下方程式是否为二次方程式。

(x – 2)(x +1)=(x – 1)(x + 3)

解决方案：

We know that a quadratic equation must be of degree 2. 

Let’s simplify and check the given equation. 

 (x – 2)(x + 1) = (x – 1)(x + 3)

⇒ x² + x – 2x – 2 = x² + 3x – x – 3

⇒ x² – x – 2 = x² + 2x – 3

⇒ -x – 2 = 2x – 3 

⇒ -3x + 1 = 0  

This equation is of degree 1. Thus, it cannot be a quadratic equation. 

求解二次方程

假设二次方程P(x)=0。满足该方程的点称为二次方程的解或零。可以找到三种方法来求解二次方程式：

1. 分解法
2. 完成平方方法
3. Shree Dharacharya或二次方程式

让我们通过示例逐一查看这三种方法。

分解法

二次方程式可以认为是两个项的因数。像ax ² + bx + c = 0可以写成(x – x ₁ )(x – x ₂ )= 0，其中x ₁和x ₂是二次方程的根。

解决步骤：

1. 找到两个数字，以使这些数字的乘积为“ ac”，且总和为“ b”。
2. 然后将x系数写为这两个数字的总和，并将它们除以得到x的两个项。
3. 将前两个项作为一个组，将后两个项作为另一个组。
4. 从这些中取公因数，并在取了公因数并重新排列方程后将两个表达式等于零，我们得到了根。

问题1：使用因式分解法找出给定二次方程的解。

2x ² – 3x +1 = 0

解决方案：

2x² – 3x + 1 = 0

⇒ 2x² – 2x – x + 1 = 0

⇒ 2x(x – 1) – 1(x -1) = 0

⇒ (2x – 1)(x-1) = 0

Now this equation will be zero when either of these two terms of both of these terms are zero

So, putting 2x – 1 = 0, we get x = $\frac{1}{2}$

Similarly, x – 1 = 0, we get x = 1 

Thus, we get two roots x = 1 and $\frac{1}{2}$

问题2：使用相同的方法找出以下二次方程的根。

2x ² – x – 6 = 0

解决方案：

2x² – x – 6 = 0

⇒ 2x² – 4x. +3x – 6 = 0

⇒ 2x (x – 2) +3(x – 2) = 0 

⇒ (2x + 3) (x – 2) = 0 

Now, 

2x + 3 = 0 

x = $-\frac{3}{2}$

x – 2 = 0 

x = 2

Thus, this equation has roots x = 2 and $-\frac{3}{2}$

完成平方方法

任何等式ax ² + bx + c = 0都可以转换为(x + m) ² – n ² = 0的形式。之后取平方根，得到等式的根。完成平方只是重新调整给定二次方程的一种方式，使它们以完整平方的形式出现。我们来看一个例子。

问题1：通过完成平方法找到给定方程的根。

x ² + 4x – 5 = 0

解决方案：

We are given, x² + 4x – 5 = 0 

To solve it by completing the square method, we need to bring it in the above mentioned form. 

x² + 4x – 5 = 0

⇒ x² + 4x + 4 – 9 = 0

⇒ (x + 2)² – 3² = 0

⇒ (x + 2)² = 3²

Taking square root both sides, 

x + 2 = 3 and x + 2 = -3 

This gives us x = 1, -5

问题2：通过完成平方法找到给定方程的根。

x ² + 6x + 9 = 0

解决方案：

Given, x² + 6x + 9 = 0

x² + 6x + 9 = 0

⇒ x² + 2(3x) + 3² = 0

⇒ (x + 3)² = 0 

Taking square root, 

x + 3 = 0 

x = – 3

Thus, this equation has only one root with multiplicity of 2. 

x = -3,-3

Shree Dharacharya或二次方程式

这个公式说

For a quadratic equation in general form, 

ax² + bx + c = 0

If b² – 4ac > 0, 

Then roots are given by $\frac{-b \pm \sqrt{b^2 - 4ac}}{2a}$

问题1：找到方程3x ² – 5x + 2 = 0的根。

解决方案：

For finding out the roots using Shree Dharacharya formula, 

We need to check If b² – 4ac > 0,

In this particular equation, a = 3, b = -5 and c = 2. 

So, b² – 4ac 

⇒ (-5)² – 4(3)(2) 

⇒ 25 – 24 

⇒ 1 > 0 

Thus, roots are possible, 

Now let’s calculate the roots by plugging in the values in the formula mentioned above. 

$\frac{-b \pm \sqrt{b^2 - 4ac}}{2a} = \frac{-5 \pm \sqrt{25 - 4(3)(2)}}{6} \\ = \frac{-5 \pm 1}{6} \\ = \frac{-4}{6}, \frac{-6}{-6} \\ = \frac{-2}{3}, -1$

问题2：找到方程式的根

$x + \frac{1}{x} = 3$

解决方案：

We need to first simplify this equation and bring it to the quadratic form so that we can apply Dharacharya Formula. 

 $x + \frac{1}{x} = 3 \\ = x^2 + 1 = 3x \\ = x^2 -3x + 1 = 0$

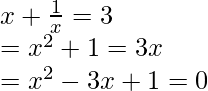
Now let’s check if b² – 4ac > 0 first. 

Here a = 1, b = -3 and c = 1

b² – 4ac 

⇒ 9. -4(1)(1) 

⇒ 5 > 0 

So we can apply the formula now, 

$\frac{-b \pm \sqrt{b^2 - 4ac}}{2a} = \frac{-(-3) \pm \sqrt{9 - 4(1)(1)}}{2} \\ = \frac{3 \pm \sqrt{5}}{2} \\ = \frac{3 + \sqrt{5}}{2}, \frac{3 - \sqrt{5}}{2} \\$