求解两个变量的线性方程对的代数方法

让我们想象一个情况，Ankita去了她村庄的集市。她想骑巨轮之类的游戏并玩Hoopla(一种游戏，在游戏中，将一个戒指扔在摊位上的物品上，如果戒指完全覆盖了任何物体，则玩家可以拿到它)。她玩Hoopla的次数是她在“巨轮”上的骑行次数的一半。如果在巨型方向盘上的每次骑行花费为3卢比，而一场Hoopla的游戏花费为4卢比，则只要她花费20卢比，就可以了解她的骑行次数以及玩Hoopla的次数。

为了解决这类问题，第一步是根据方程式将其表述。令x为Ankita骑巨轮的次数，y为她扮演Hoopla的次数。等式变成

x = 2y，

3x + 4y = 20。

我们可以找到该方程组的解吗?找到解决方案的方法有几种，下面让我们详细介绍一下。

对两个变量的线性方程组

可以以ax + x + c = 0形式表示的方程，其中a，b和c是实数，而a和b都非零，被称为两个变量x和y的线性方程。在几何上，如果所有满足该方程的点都绘制在笛卡尔平面上。它代表一条线。

类似地，由两个线性方程组组成的系统代表两条线。该系统的解表示满足两个方程的点。可以没有点，也可以只有一个点，也可以有无限多个点。

一个解决方案，没有解决方案，以及无限多个解决方案。

求解线性方程组的代数方法

有几种方法可以代数求解线性方程组，让我们看一下其中的两种方法：

• 替代方法
• 消除方法

替代方法

该方法主要包括两个步骤：

步骤1：找到一个变量的值，以另一个变量的形式表示y，即从任一等式中的x取其方便。

步骤2：将y的值替换为另一个方程式，并将其简化为一个变量的方程式，即以x表示，可以求解。

问题：求解以下方程组：

7x – 15y = 2

x + 2y = 3

解决方案：

It can be solved according to the steps explained above, 

We pick an equation to represent value of one variable in terms of others, 

Let’s pick, x = 3- 2y (It’s also convenient). 

Substitute the value of x in the other equation. 

7(3-2y) -15y = 2 

⇒ 21 – 14y -15y = 2 

⇒ 19 = 29y 

⇒ y = 19/29

Now, the x value becomes x = 3 – 2(19/29) = 3 – (38/29) = 49/29

也可以通过将这两个值代入这些方程式来检查该解决方案。  

Note: We have substituted the value of one variable by expressing it in terms of other variable to solve the problem. That’s why this method is called substitution.

有时，如下面的示例所示，我们可以获得没有变量的语句。如果这个说法是正确的，我们可以说这对线性方程组具有无限多个解。如果该语句为假，则一对线性方程组不一致。

问题：Anuj和Rahul从商店购买了一些文具。 Anuj买了2支铅笔和3支橡皮。 2支铅笔和3块橡皮的价格为9卢比，Rahul购买了4支铅笔和6块橡皮。 4支铅笔和6块橡皮的成本为18卢比。找到每支铅笔和每个橡皮的成本。

解决方案：

The pair of linear equations that is formed from the above description is,

2x + 3y = 9 

4x + 6y = 18

where x is the cost of a pencil and y is the cost of eraser. 

x = $\frac{9 - 3y}{2}$

Putting this value in the other equation. 

$4(\frac{9 - 3y}{2}) + 6y = 18 \\ 18 - 6y + 6y = 18 \\ 18 = 18$

This statement is true for all values of y. However, we do not get a specific value of y as a solution. Therefore, we cannot obtain a specific value of x. This situation has arisen because both the given equations are the same. Therefore, these equations both have infinitely many solutions. 

消除方法

该方法主要包括以下步骤：

1. 将两个方程式乘以一些合适的非零常数，并使一个变量(x或y)的系数在数值上相等。
2. 在另一个方程式中添加或减去一个方程式，以便消除一个变量。如果获得一个变量中的方程，请转到步骤5。
3. 如果在步骤2中获得了不包含任何变量的真实语句，则原始方程对将具有无限多个解。
4. 如果在步骤2中获得了一个不包含变量的错误陈述，则原始方程对没有解，即不一致。
5. 将方程式求解为一个变量(x或y)以获得其值。
6. 将这个x值(或y值)替换为两个原始方程式中的一个，即可获得另一个变量的值。

问题1：使用消除法找到以下方程组的所有可能解。

2x + 3y = 8 –(1)

4x + 6y = 7 –(2)

解决方案：

Multiply equation (1) by 2 and equation (2) by 1. This will make coeffecients of x in both the equations same. Then we get the equations as: 

4x + 6y = 16 

4x + 6y = 7

Subtracting both of the equations, 

we get 0 = 9 

This is false. So, no solution exists for this system of linear equations. 

问题2：一个两位数的数字和通过反转数字获得的数字的总和为66。如果该数字的数字相差2，则找到该数字。有多少个这样的数字?

解决方案：

Let x and y be the ten’s and unit’s digit respectively. So the first number can be written as 10x + y is the expanded form(for example 56= 10(6) + 5). 

(10x + y) + (10y + x) = 66, 

i.e⇒ 11(x + y) = 66 

i.e ⇒ x + y = 6

It’s also given that the digits differ by 2,

So, either x – y = 2 or y – x = 2. Let’s look for both of the cases, 

Case 1: x – y = 2, 

Substituting x = y + 2 in the equation given above, 

y + 2 + y = 6

⇒ 2y = 4 

⇒ y = 2. 

So, x = 4 

Case 2: y – x = 2, 

Substituting y = x + 2, in the equation given above, 

x + y = 6. 

⇒ x + x + 2 = 6 

⇒ 2x = 4

⇒ x = 2

y = 4. 

交叉相乘法

确定线性方程在两个变量中的解的技术之一，众所周知这是最快的方法。

假设一对线性方程：

a ₁ x + b ₁ y + c ₁ = 0

a ₂ x + b ₂ y + c ₂ = 0

By using Cross-multiplication, we can find the values of ‘x’ and ‘y’:

$x=\frac{(b_{1}c_{2})-(b_{2}c_{1})}{(b_{2}a_{1})-(b_{1}a_{2})}\\y=\frac{(c_{1}a_{2})-(c_{2}a_{1})}{(b_{2}a_{1})-(b_{1}a_{2})}$

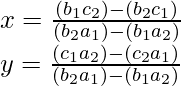
交叉乘法的推导

a ₁ x + b ₁ y + c ₁ = 0⇢(1)

a ₂ x + b ₂ y + c ₂ = 0⇢(2)

分别乘以方程式(1)中的_{b 2}和方程式(2)中的_{b 1：}

a ₁ b ₂ x + b ₁ b ₂ y + c ₁ b ₂ = 0(3)

a ₂ b ₁ x + b ₁ b ₂ y + c ₂ b ₁ = 0⇢(4)

从等式(3)中减去等式(4)将得到x和y的值，

$x=\frac{(b_{1}c_{2})-(b_{2}c_{1})}{(b_{2}a_{1})-(b_{1}a_{2})}\\y=\frac{(c_{1}a_{2})-(c_{2}a_{1})}{(b_{2}a_{1})-(b_{1}a_{2})}$

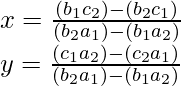
轻松掌握公式的技巧

以原始形式写下系数：

a ₁ b ₁ c ₁

a ₂ b ₂ c ₂

忽略x的系数并乘以其余系数，然后减去它们：

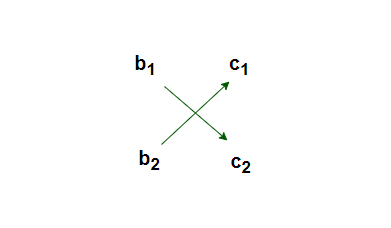
因此，第一部分方程的解变为⇒  $\frac{x}{(b_{1}c_{2})-(b_{2}c_{1})}$

现在，忽略y的系数，并将其余项乘以：

请记住要考虑(-y)下的等式，解的第二部分⇒ $\frac{-y}{(a_{1}c_{2})-(a_{2}c_{1})}$

对于第三部分，请忽略系数1并将其余项与它们相乘并相减：

等式的第三部分变为⇒ $\frac{1}{(a_{1}b_{2})-(a_{2}b_{1})}$

结合所有三个部分⇒ $\frac{x}{(b_{1}c_{2})-(b_{2}c_{1})}=\frac{-y}{(a_{1}c_{2})-(a_{2}c_{1})}=\frac{1}{(a_{1}b_{2})-(a_{2}b_{1})}$

问题1：使用交叉乘法找到满足方程式的变量的值：

2x + 3y = 11

3x + 2y = 9

解决方案：

Write equations in general form: 

2x + 3y – 11 = 0

3x + 2y – 9 = 0

Using Cross- multiplication method,

$\frac{x}{(b_{1}c_{2})-(b_{2}c_{1})}=\frac{-y}{(a_{1}c_{2})-(a_{2}c_{1})}=\frac{1}{(a_{1}b_{2})-(a_{2}b_{1})}$

Put the values of all the coefficients in the formula,

$\frac{x}{(3.(-9))-(2.(-11))}=\frac{-y}{(2.(-9))-(3.(-11))}=\frac{1}{(2.2)-(3.3)}$

$\frac{x}{-5}=\frac{-y}{15}=\frac{1}{-5}$

Solving, we get: x=1, y=3

问题2：使用交叉乘法，求解x和y：

3x – 4y – 2 = 0

y – 2x – 7 = 0

解决方案：

Using the formula: $\frac{x}{(b_{1}c_{2})-(b_{2}c_{1})}=\frac{-y}{(a_{1}c_{2})-(a_{2}c_{1})}=\frac{1}{(a_{1}b_{2})-(a_{2}b_{1})}$

Substitute the values in the formula: $\frac{x}{(-4.(-7))-(-2.(-2))}=\frac{-y}{(3.(-7))-(1.(-2))}=\frac{1}{(3.(-2))-(1.(-4))}$

Solving the equation, x=-6, y=-5