线性方程组只是两条可能相交或不相交的线。线性方程的图形是一条线。有几种方法可以用来求解两个线性方程,例如,代换法,消除法等。它们也可以使用图形方法来求解,在这种方法中,我们绘制方程的图并尝试找到该交点。首先,让我们看一些线性方程及其图。
线性方程和图形
要将两个线性方程式放在图形上,请在图形上绘制它们,然后查看它们在何处以及是否相交。图上相交的线给出了相交点,称为线性方程的解。让我们看一下一对线性方程及其来自现实世界中问题的图表。
问题1: Roman到文具店以9卢比的价格购买了2支铅笔和3个橡皮擦。他的朋友Sonam用Roman看到了新的铅笔和橡皮擦,她还以18卢比的价格购买了4支铅笔和6种橡皮擦。 。用数字和图形方式表示这种情况。
解决方案:
Let’s say cost of one pencil is Rs.x and cost of one eraser be Rs. y. Then the equations can be formulated as,
2x + 3y = 9,
4x + 6y = 18,
Let’s plot both of these on graph,
By putting them in intercept form,
![]()
![]()
The graph of both equations looks as follows,

Both of these lines have same equations, and thus they coincide.
问题2:板球队的教练以3900卢比的价格购买了3球拍和6球。后来,他以1300卢比的价格购买了另一把球拍和3个相同种类的球。用代数和几何方法表示这种情况。
解决方案:
Let’s say that cost of each bat is Rs. “x” and Balls is Rs. “y”.
3x + 6y = 3900
x + 3y = 1300
Let’s make graph of these equations, it can be made through any of the forms that were taught, but we will stick to intercept form
![]()
![]()
Now the graphs of both equations come out to be,

We can see that these lines intersect at one point.
解决方案的图形化方法
在这种方法中,我们绘制了两个方程,然后尝试找到交点。现在在绘制图形并找到可能的交点时,可能会发生三种情况
- 独特的解决方案
- 没有解决方案
- 无限多个解决方案
独特的解决方案
在两个变量中具有解决方案的一对线性方程式称为一致线性方程对。在这种情况下,两条线彼此相交。
例如:
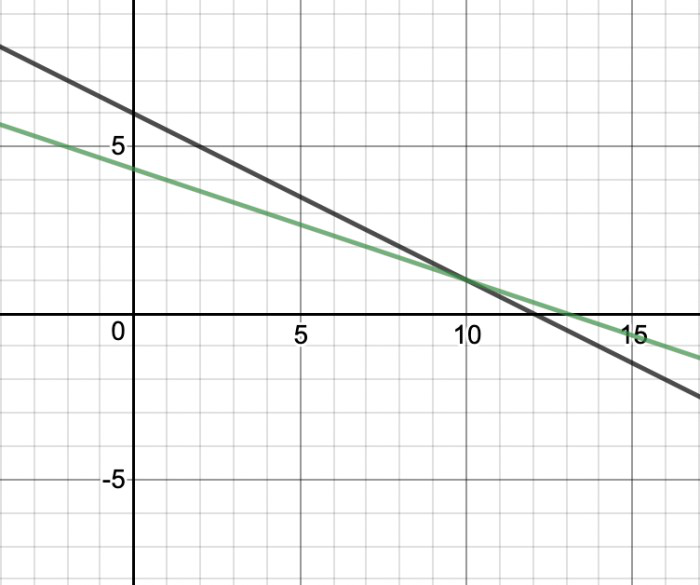
问题1:使用图形方法求解给定的方程对。
x + 3y = 6
2x – 3y = 12
解决方案:
We need to first plot both of them on to find the solution. Let’s bring them in intercept form,
![]()
![]()
Both of these equations can now be plotted on graph.

We can see on graph that both these lines intersect on (6,0) so this is the solution to this pair of linear equations.
问题2:拉吉尼去商店买裤子和裙子。当她的朋友问她买了多少条裙子时,她回答说:“裙子的数量是裤子的两倍,不到裤子的两倍。而且,裙子的数量比所购买裤子的数量少四倍。”帮助她的朋友找到Ragini购买了多少条裤子和裙子。
解决方案:
Let’s say there are “x” number of pants and “y” number of Skirts. Then following equations are formed.
y = 2x – 2
y = 4x – 4
Let’s draw graphs for both the equations,
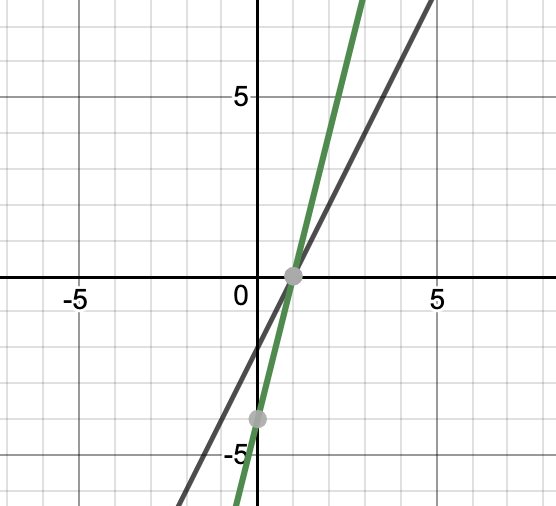
From graph, we can see that these lines intersect at (1,0). So this is the solution for this system of equations
x = 1 and y = 0
That means that Ragini brought 1 pant and 0 skirts.
没有解决方案
在两个变量中没有解决方案的一对线性方程称为不一致的线性方程对。在这种情况下,两条线是平行的,因此它们永不相交。
例如:
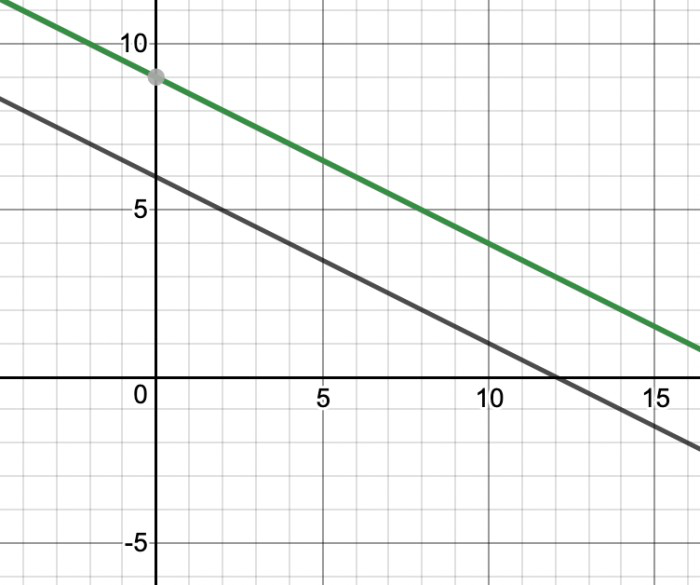
问题:在图形上针对给定的两个方程式绘制线性方程式,
7x- 9y = 4
7x- 9y = 12
解决方案:
The graph for the given two equations will look like,
As it is clear that the lines are not intersecting each other at any point, they are parallel in nature. Hence, there is no solution for the given linear equations.
无限多个解决方案
两个变量中具有无限多个解的一对线性方程称为从属线性方程对。在这种情况下,两条线彼此重合。
例如:

让我们概括以上情况,
a 1 x + b 1 y + c 1 = 0
a 2 x + b 2 y + c 2 = 0
这些等式是
- 相交是否

- 巧合的是,
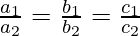
- 如果平行,

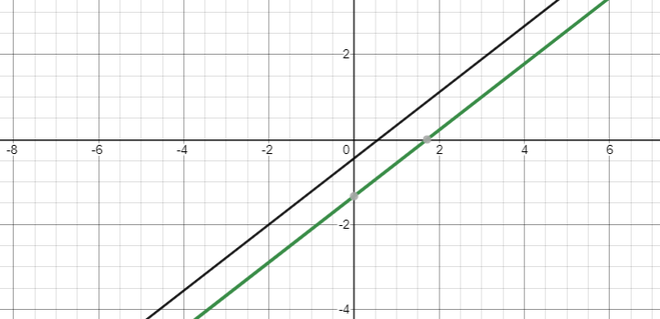
问题:在图形上绘制线性方程,还找到两个线性方程的解。
8x + 13y = 17
16x + 26y = 34
解决方案:

The two equations are not only parallel, they are overlapping each other, hence all the points are intersecting each other. Therefore, There exist infinitely many solutions for the given linear equations.