平均和瞬时变化率

平均变化率表示一个变量相对于另一变量的总变化的总变化。瞬时变化率或导数测量一个变量相对于另一个变量的特定的，无限微小的变化的特定变化率。函数的平均变化率可以用割线确定，瞬时变化率可以用切线确定。正如您将学到的，这些比率也可以使用一种称为演算的特殊类型的数学来确定。

变化率是多少?

变化率是一个变量相对于另一个变量的变化的变化。常见的变化率是速度，它衡量行驶距离相对于经过时间的变化。奥运金牌获得者Usain Bolt在100米冲刺中以44.72公里/小时的最高速度成为世界上跑得最快的人。平均而言，他的速度为37.58公里/小时，速度稍慢(不过非常令人印象深刻)。博尔特的最高速度就是瞬时变化率的一个例子，而他的平均速度就是平均变化率。

平均变化率

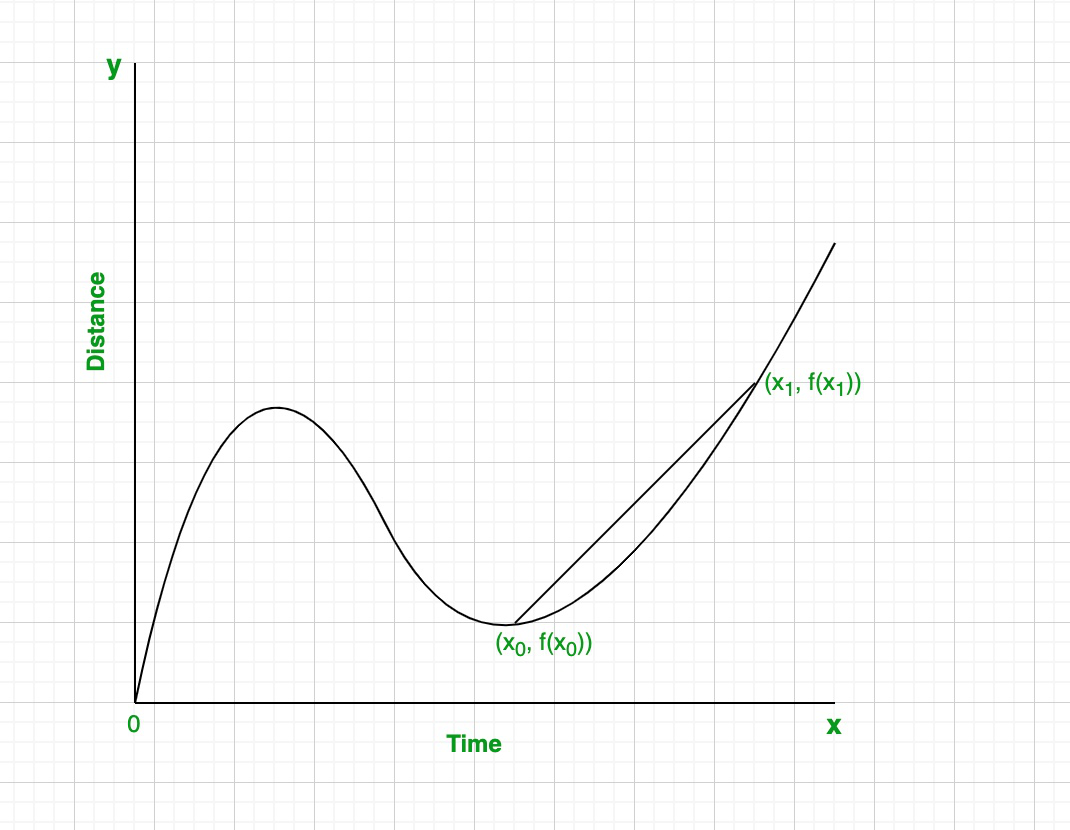
通过连接曲线上的两个点可以找到正割线。两点之间的割线斜率表示该间隔的平均变化率。

公式：

Average Rate of Change = Slope(m) = △y/△x = $\frac{y_{2} - y_{1}}{x_{2} - x_{1}}$ = $\frac{f(x_{1}) - f(x_{0})}{x_{1} - x_{0}}$

如何使用割线找到两点之间的平均变化率：

第1步：绘制一条连接这两个点的割线。

步骤2：使用两点的坐标来计算斜率。

Equation of slope:
Slope =$\frac{y_{2} - y_{1}}{x_{2} - x_{1}}$

The avarage change of the function over the given time interval[x₀, x₁]
Slope = $\frac{f(x_{1}) - f(x_{0})}{x_{1} - x_{0}}$

The slope of the secant line represents the average
rate of change of the graph in that interval.

一旦计算出割线的斜率，就可以使用该斜率写一个方程式来表示它。

For Example:
Equation of slope:
y – y₀ = m(x – x₀)

m = slope of secant line = 4/7
x₀ = 2
y₀ = 9
$y - 9 = \frac{4}{7}(x -2)$

Hence, the equation of the secant line between x = 2 and x = 9 is 
y – 9 = $\frac{4}{7}(x -2)$

衍生产品(瞬时变化率)

在一个点的切线通过绘制一条直线，在该点接触的曲线，而不跨过曲线找到。换句话说，该线在局部应仅接触一个点。一点处切线的斜率表示该点的瞬时变化率或导数。

公式：

Instantaneous Rate of Change = $f'(x_0) = \lim_{(x_0 \to x_1)} \frac{f(x_{1}) - f(x_{0})}{x_{1} - x_{0}}=\lim_{\triangle x \to 0} \frac{f(x_{0}-\triangle x) - f(x_{0})}{\triangle x}$

如何使用切线在一点上找到导数：

步骤1：在该点画一条切线。

步骤2：使用该线上任意两点的坐标来计算斜率。

Equation of slope:
Slope = $\frac{y_{2} - y_{1}}{x_{2} - x_{1}}$

The avarage change of the function over the given time interval x₀
Slope = $f'(x_0) = \lim_{(x_0 \to x_1)} \frac{f(x_{1}) - f(x_{0})}{x_{1} - x_{0}}=\lim_{\triangle x \to 0} \frac{f(x_{0}-\triangle x) - f(x_{0})}{\triangle x}$

The slope of the tangent line at a point represents
the instantaneous rate of change, or derivative, at that point.

一旦计算出切线的斜率，就可以编写一个方程式来表示它。

For Example: Equation of slope:
y – y₀ = m(x – x₀)

m = slope of tangent line = $\frac{5}{6}$
x₀ = 16
y₀ = 6
$y - 16 = \frac{5}{6}(x - 6)$

Hence, the equation of the tangent line at x = 16 is 
y – 16 = \frac{5}{6}(x – 6) 

导数符号

在18世纪初期，伟大的数学家艾萨克·牛顿( Isaac Newton)与戈特弗里德·威廉·莱布尼兹(Gottfried Wilhelm Leibniz)之间就谁是第一个发明微积分的问题发生了争议。这种说法被称为Prioritätsstreit ，或德语中的“优先权争议”。分歧对数学世界产生了持久的影响，给我们留下了两个标准的导数符号。拉格朗日符号是另一种常见的派生符号，由法国数学家和哲学家约瑟夫·路易斯·拉格朗日( Joseph-Louis Lagrange)建立。

如果我们采用函数y = f(x)，那么

1. Leibniz表示法：

“First derivative of y with respect to x”
⇒$\frac{dy}{dx}$

“Second derivative of y with respect to x”
⇒$\frac{dy^2}{d^2x}$

“First derivative of y with respect to x
at x = 2”
⇒$\frac{dy}{dx}|_{x=2}$

2.牛顿符号：

The number of dots above the function
variable represents how many times the
function has been differentiated.
“First derivative of y”
⇒$\dot y$
“Second derivative of y”
⇒$\ddot y$
“First derivative of y at x = 2”
⇒$\dot y (2)$

3.拉格朗日符号：

The number of apostrophes after the function
variable represents how many times the
function has been differentiated.
“First derivative of y”
⇒y’
“First derivative of y at x = 2”
⇒y'(2)
“Second derivative of y”
⇒y”

4.欧拉符号

“First derivative”
⇒ D_xf
“Second derivative”
⇒ D_x²f
Here, D represents the differential operator

样本问题

问题1.找到区间x = 4，x = 6的平均变化率。

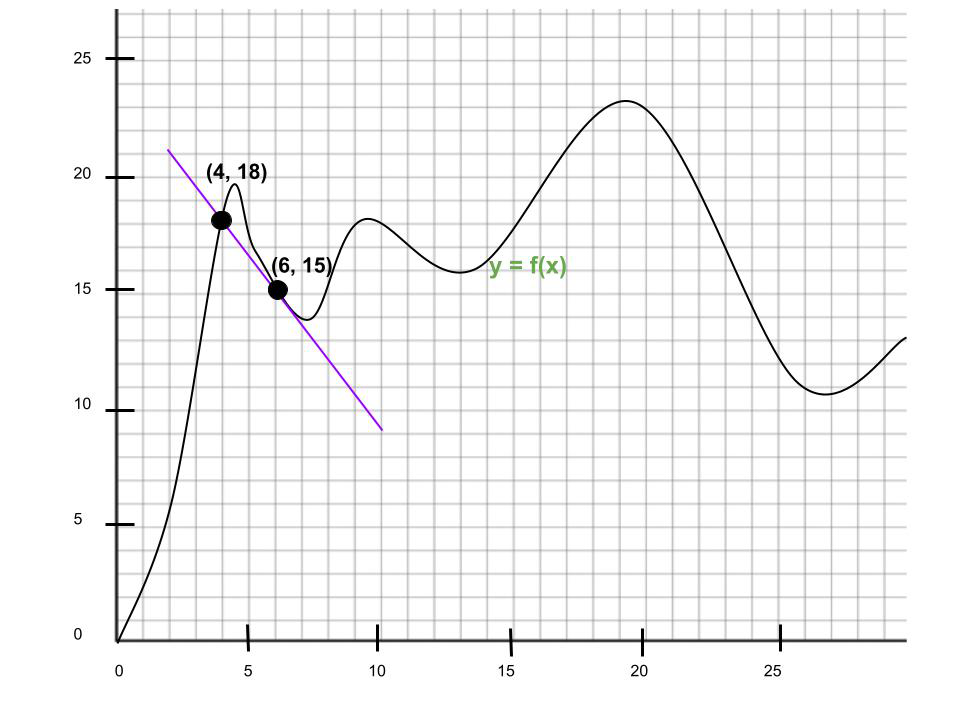
解决方案：

Point 1: (4, 18)

Point 2: (6, 15)

Slope = $\frac{y_{2} - y_{1}}{x_{2} - x_{1}}$

= $\frac{15 - 18}{6-4}$

= $-\frac{3}{2}$

Hence, the average rate of change = $- \frac{3}{2}$

问题2.写出x = 16处的切线方程。

解决方案：

Point of intersection: (16, 18)

Slope = $\frac{23 - 18}{18 - 16}$

= $\frac{5}{2}$

Equation of tangent line: 

 $y - 18 = \frac {5}{2}(x - 16)$ 

问题3.在x = 20时图的导数是什么?用Leibniz表示法表达您的答案。

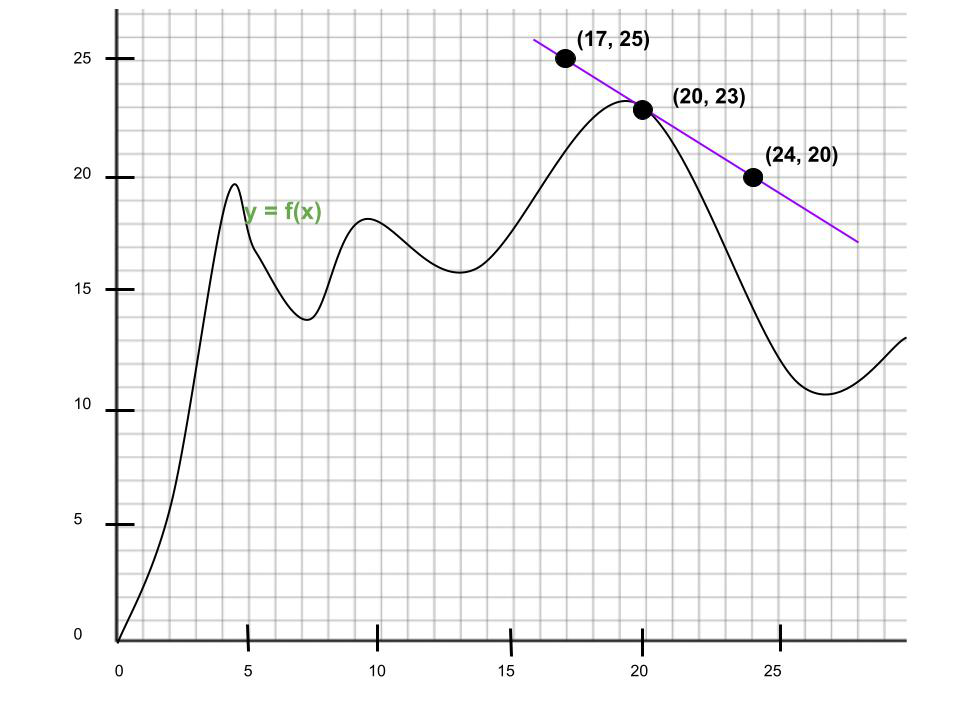
解决方案：

Given point of intersection: (20, 23)

Slope of tangent line = $\frac{20-25}{20-17}$

= $-\frac{5}{3}$

So, the Leibniz notation: $\frac {dy}{dx} | _{x=20} = -\frac{5}{3}$

问题4.在x = 4处找到图的导数。用牛顿符号表示答案。

解决方案：

Given point of intersection: (4, 18) 

Slope of tangent line = $\frac{25 - 18}{7-4}$ = $\frac{7}{3}$

So, the Newton’s notation$\dot y(4) = \frac{7}{3}$

问题5：求出区间x = 4，x = 25的平均变化率。这与x = 4时的导数相比如何?

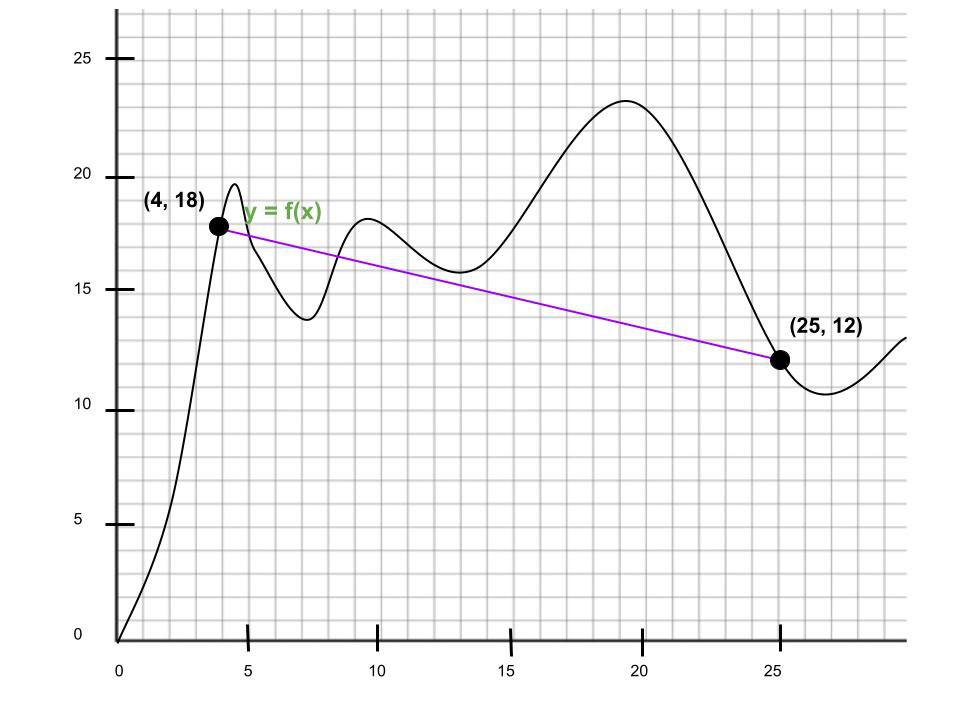
解决方案：

Slope of secant line = $\frac {12 - 18}{25-4}$

= $- \frac{6}{21}$

The average rate of change over x = 4, x = 25 is -6/21, 

which is less than the derivative of y at x = 4, which we found to

be 7/3. 

问题6：求出给定函数f(x)= 2x ² + 18在x = 9时的瞬时变化率?

解决方案：

Given: f(x) = 2x² + 18

f'(x) = 4x + 0

f'(x) = 4x 

Now we have to find the instantaneous rate of change at x = 9

f(9) = 4x

f(9) = 4(9)

f(9) = 36

问题7.求出给定函数f(x)= 4x ² + 12x + 8的瞬时变化率，其中x = 4?

解决方案：

Given: f(x) = 4x² + 12x + 8 

f'(x) = 8x + 12

Now we have to find the instantaneous rate of change at x = 4

f(4) = 8x + 12

f(4) = 8(4) + 12

f(4) = 44