作为变化率的导数

导数被认为是分析任何数量变化的数学方法。我们研究了计算不同类型函数的导数，例如三角函数、指数函数、多项式和隐函数。导数的计算主要有两种方法：基于极限的方法或使用不同的公式和规则，如乘积规则、商规则或链式规则。导数有不同的应用，这使得它们在处理现实世界中的物理现象时非常有用。让我们看看其中的一些应用程序。

变化率

导数是微积分中使用的基本原理。它们用于显示函数的特征，无论它们是增加还是减少等。想象我们有两个相互变化的量。假设这些量用“x”和“y”表示。它们通过某种规则相互关联

y = f(x)

那么这个函数的导数，即$\frac{dy}{dx}$是 y 相对于 x 的变化率，它表示 y 相对于 x 的变化。

示例：求边以 2 m/s 的速率增加的立方体的体积变化率。

解决方案：

Let’s say the length of the side of cube is “a”. The volume of cube is given by, V = a³. 

$\frac{dV}{da} = \frac{d(a^3)}{da}$

⇒ $\frac{dV}{dt} = 3a^2\frac{da}{dt}$

⇒$\frac{dV}{dt} = 3a^2(2)$

⇒$\frac{dV}{dt}$ = 6a² m³/s. 

增减函数

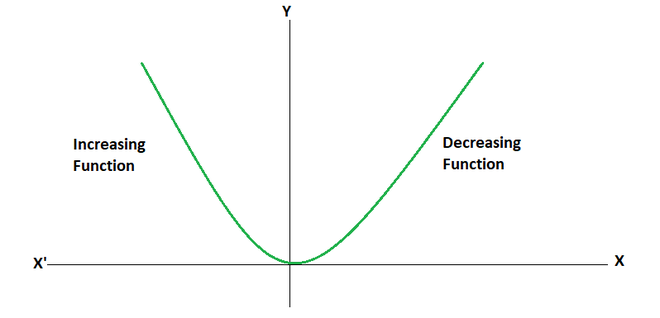
导数也用于找出函数是增加还是减少，或者它们都没有。下图显示了函数f(x) = x ² 。

注意图中，函数在 (-∞, 0) 区间内递减，在 (0,∞) 区间内递增。

In an interval I contained in the domain of real valued function “f”. Then, f is said to be, 

• Increasing on I, if x₁ < x₂ in I ⇒ f(x₁) ≤ f(x₂) for all x₁, x₂ ∈ I.
• Strictly Increasing on I, if x₁ < x₂ in I ⇒ f(x₁) < f(x₂) for all x₁, x₂ ∈ I.

• Decreasing on I, if x₁ < x₂ in I ⇒ f(x₁) ≥ f(x₂) for all x₁, x₂ ∈ I.
• Strictly Decreasing on I, if x₁ < x₂ in I ⇒ f(x₁) > f(x₂) for all x₁, x₂ ∈ I.

现在我们知道了增减函数的定义。让我们看看如何识别在一个区间内增加或减少的函数。

Let’s say f is continuous on [a, b] and differentiable on the open interval (a, b). Then, 

1. f is increasing in (a, b) if f'(x) > 0 in the interval [a, b].
2. f is decreasing in (a, b) if f'(x) < 0 in the given interval.
3. f is constant if f'(x) = 0.

示例问题

问题 1：假设我们有一个半径在增加的圆。求 r = 4cm 时面积随半径的变化率。

解决方案：

Let’s say “A” is the area of the circle and “r” be the radius of the circle.

A = πr²

Differentiating it with respect to radius. 

$\frac{dA}{dr} = \frac{d(\pi r^2)}{dr}$

⇒ $\frac{dA}{dr} = 2\pi r$

At r = 4. 

$\frac{dA}{dr} = 8\pi$

问题 2：假设我们有一个矩形，它的边每秒都在变化。长度以 3 m/s 的速度增加，而宽度以 8 m/s 的速度增加。计算当长 = 8m 和宽 = 5m 时矩形面积增加的速率。

解决方案：

Let, x be the length of the rectangle and y be the breadth of rectangle. 

$\frac{dx}{dt} = 3$ And $\frac{dy}{dt} = 8$

The area of rectangle is given by, 

A = xy

Differentiating the equation w.r.t time. 

$\frac{dA}{dt} = \frac{d(xy)}{dt}$

⇒ $\frac{dA}{dt} = x\frac{dy}{dt} + y\frac{dx}{dt}$

⇒ $\frac{dA}{dt} = x\frac{dy}{dt} + y\frac{dx}{dt}$

⇒$\frac{dA}{dt} = 8(\frac{dy}{dt}) + 5(\frac{dx}{dt})$

⇒ $\frac{dA}{dt} = 8(8) + 5(3)$

⇒ $\frac{dA}{dt}$ = 64 + 15

问题 3：对于给定的曲线，找到 y 的变化率值为零的点。

y = x ² + x

解决方案：

y = x² + x

$\frac{dy}{dx} = \frac{d(x^2 + x)}{dx}$

⇒$\frac{dy}{dx} = 2x + 1$

This rate of change must be zero, 

2x + 1 = 0 

⇒ x = $\frac{-1}{2}$

Thus, at x = $\frac{-1}{2}$ the rate of change is zero. 

问题 4：证明上面讨论的函数f(x) = x ²在 (0, ∞) 区间内递增。

解决方案：

According to above definition, a function in increasing in any interval if its derivative is greater than zero in that interval. 

f(x) = x²

Differentiating with respect to x, 

f'(x) = 2x 

For the given interval (0,∞) f'(x) > 0. 

Thus, the function is increasing in the given interval. 

问题 5：找出函数f(x) = x ² + 5x + 6 增加或减少的区间。

解决方案：

Given f(x) = x² + 5x + 6

f'(x) = 2x + 5

We need to study the sign of the derivative to find the intervals where this function is increasing or decreasing. 

f'(x) < 0 

⇒ 2x + 5 < 0 

⇒ x < $\frac{-5}{2}$

f'(x) > 0 

⇒ 2x + 5 > 0 

⇒ x > $\frac{-5}{2}$

Thus, the function is increasing in ($\frac{-5}{2}$, ∞) and is decreasing in (-∞, $\frac{-5}{2}$).