行列式的次要和辅因子

矩阵是实数(或其他合适的实体)的数组，排列成行和列，其中实体是指矩阵中存在的元素。下图演示了矩阵，其中水平分隔的元素被称为矩阵的行，垂直分隔的元素被称为矩阵的列。

众所周知，矩阵是按行和列排列的，下面的矩阵有3行和3列，所以矩阵的顺序是3×3。

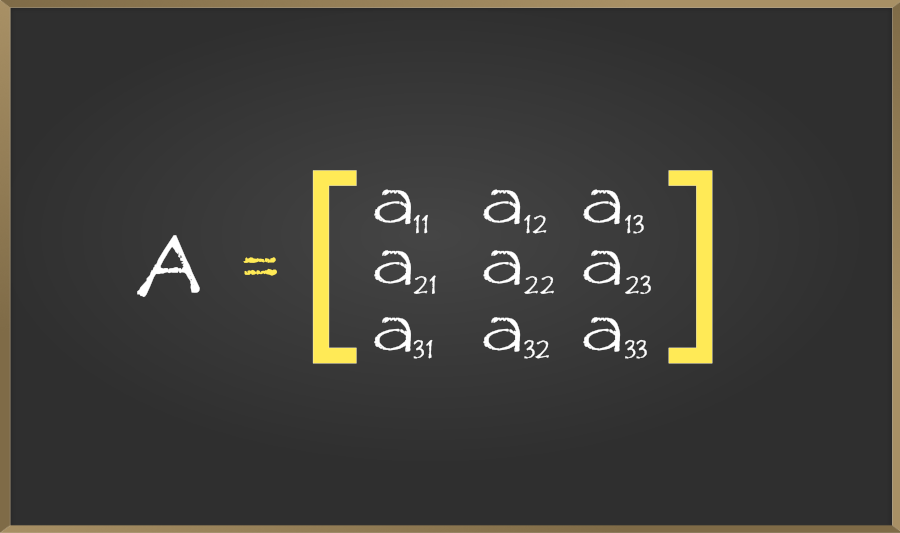
任何四个元件的a，b，c和d被布置在两行和两垂直条之间的两个列中，如下所示，这是所谓的第二阶或二阶行列式的行列式形式。如下所示，展示了行列式和行列式的扩展。

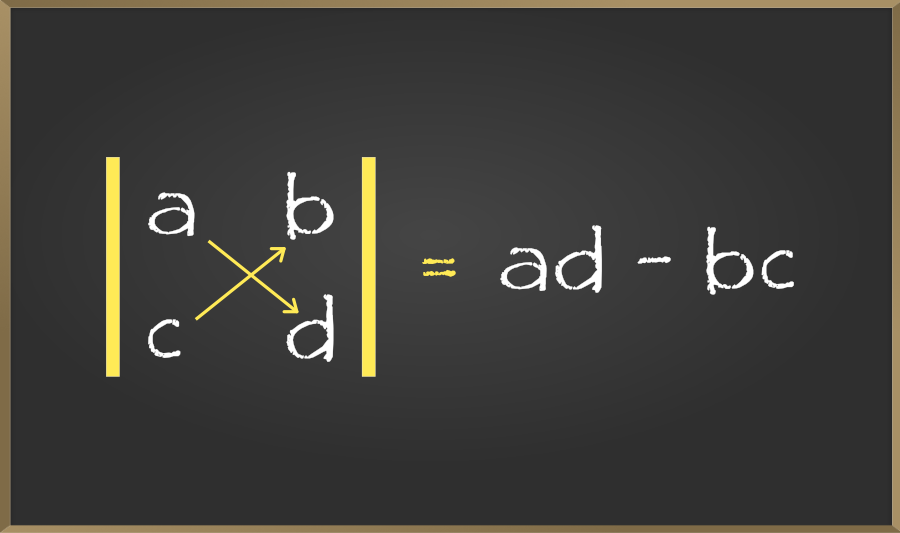
矩阵的行列式

行列式对于求解线性方程式，捕获线性变换如何改变面积或体积以及改变积分中的变量很有用。行列式可以看做一个函数，其输入是一个方矩阵，其输出是一个数字。在下面的文章中，我们将全面讨论未成年人和辅助因素。用简单的语言，我们可以说，对于每个小矩阵A，我们可以将一个数字(实数或复数)关联起来，这称为方矩阵A的行列式。

矩阵的行列式可以很容易地表示为det(A)或| A |

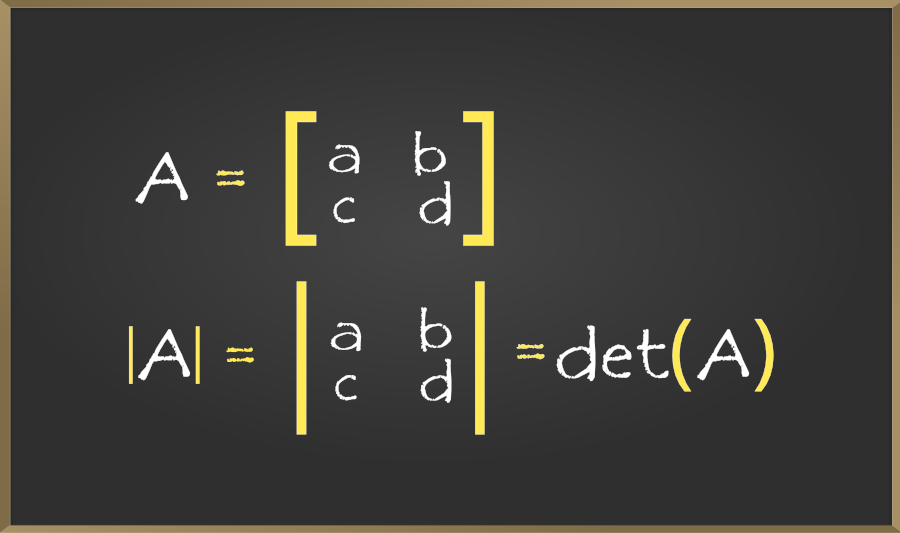
现在，让我们跳到未成年人和辅助因素这一主题。

因此，首先让我们讨论未成年人。

笔记：

• 本文中完成的问题已出现在去年的不同问题论文中。
• i代表行列式的行，而j代表行列式的列。
• 我强调第ij^个术语，以便您可以清楚看到而不会造成任何混淆。
• 在下面的文章中，您将看到该问题，该问题的解决方案通过图像进行了演示。

矩阵的次要

次要元素a _ij 行列式的，是通过删除^第i行和^第j列所获得的行列式，其中元件的_IJ 说谎。次要元素a _ij 用M _ij表示

计算矩阵次要的步骤

步骤1：隐藏第i矩阵A的^第i行和^第j列，其中，所述元件的_IJ所在。

步骤2：现在，使用步骤1删除行和列后，计算矩阵的行列式。

矩阵次要样本问题

问题1：如果矩阵A为

然后，写下₂₂岁的未成年人_。

解决方案：

In this question, we have to find out the minor of a₂₂, the element present at a₂₂ is 0. As we learn from our definition of a minor we have to delete the i^th row and j^th columns at which our asked element is present. Below image is demonstrating how to delete the i^th row and j^th column

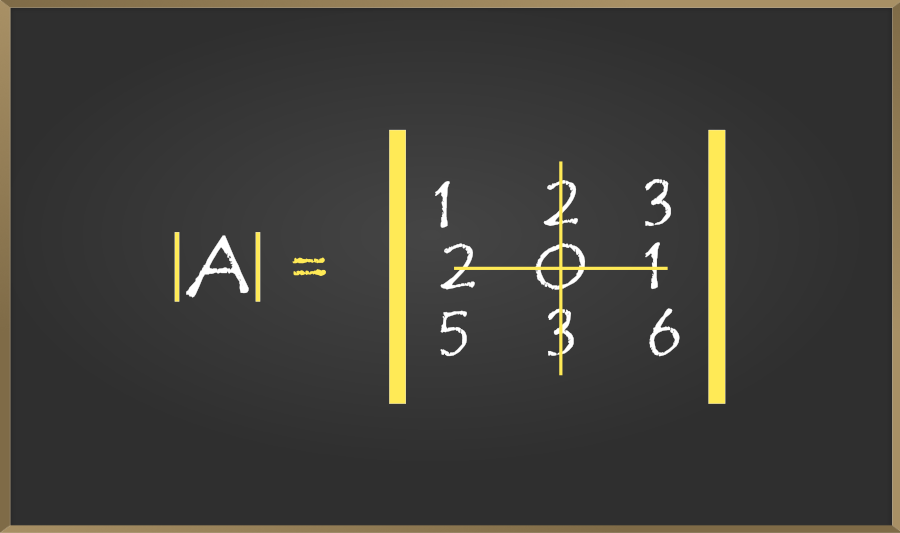
After deletion, we write our left element as it is and do cross multiplication.

Now after deleting i^th row and j^th column we had to expand the determinant, so we get (8 – 15) which on solving gives -7, which is our required answer.

Note: Always remember, after multiplication of left diagonal element always put -ve sign then do the multiplication of right diagonal elements and solve them out.

问题2：如果矩阵A为

然后找出_32岁的未成年人_。

解决方案：

In the above question we have asked to find out the minor of a₃₂ element which is 1. So as we did in this above problem same procedure we will follow. So firstly we have to delete the i^th row and j^th column at which our element is present.

So we had canceled the i^th row and j^th column at which our element is present. So write the elements which are left as it is.

Then do the cross multiplication and solve:

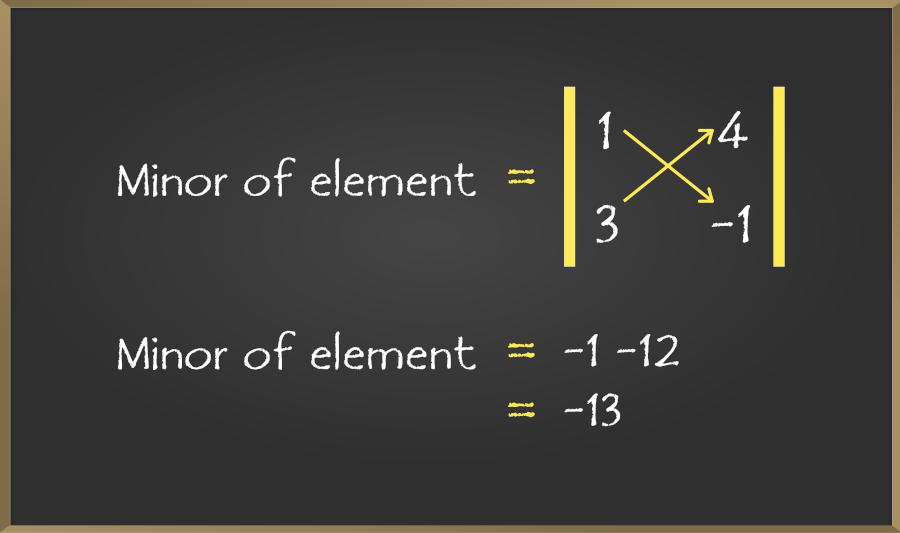
通过遵循与上述问题相同的过程，我们也通过在引言中讨论的行列式扩展来解决了该问题。

矩阵的辅因子

行列式元素a _ij的辅因子，用A _ij或C _ij表示，定义为A _ij =(-1) ^{i + j} M _ij ，其中M _ij是元素a _ij的次要元素

查找辅助因子的公式

A _ij =(-1) ^{i + j} M _ij

矩阵的辅助因子的样本问题

问题1：如果矩阵A为

将元素的辅因子写成_32。

解决方案：

As asked in question we have to find the co factor of element a32 which means our row (i) = 3 and column (j) = 2 so we have row and column as we do to find the minor by deleting the rows and column at which asked element exist we do the same in this question to and then put that in our formula -> A_ij = (-1)^i+j  M_ij

So after putting in the formula of finding cofactor and doing expansion of determinant we get (-1) (5 – 16) which on solving gives the answer 11, this is our required answer.

问题2：如果下面给出行列式元素_ij的元素_ij ，则写出₃₂的值。 _32岁

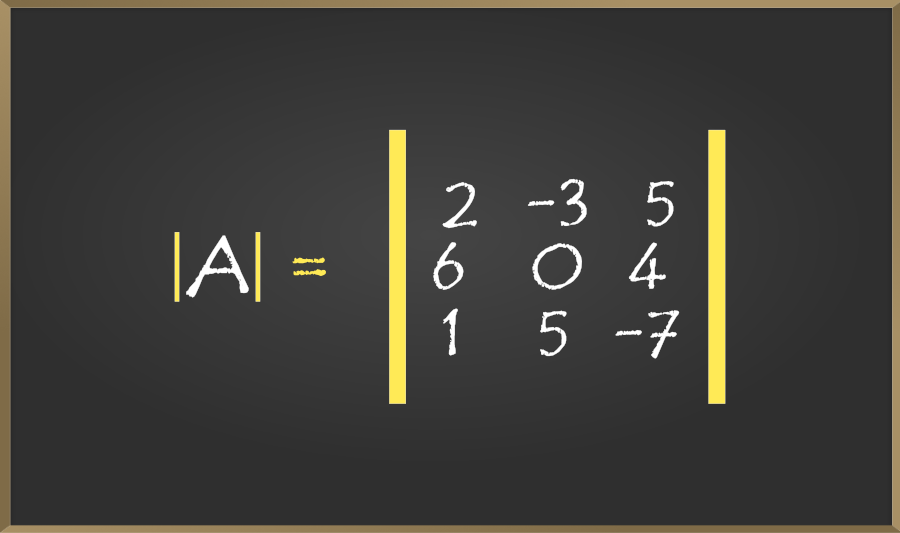
解决方案：

In the question, we are having determinant. So we have row and column given in the question.

Here, a₃₂ = 3+2 = 5

Given, A_ij is the cofactor of the element a_ij of A . So now we can solve this question by putting the values in the formula of cofactor as discussed in above question.

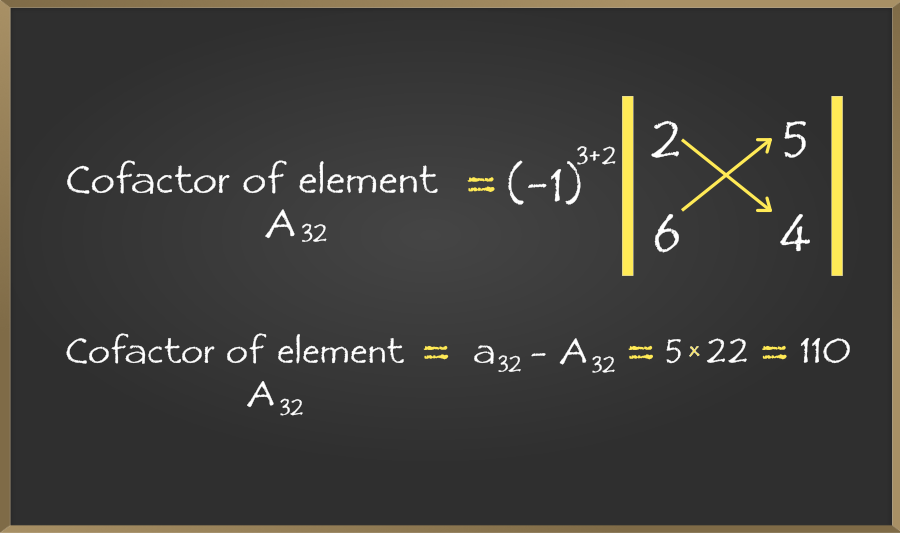
So, 110 is our required answer.