第12类RD Sharma解–第19章不定积分–练习19.9 |套装1

评估以下积分：

问题1.∫(log⁡x)/ x dx

解决方案：

Given that, I = ∫(log⁡x)/x dx

 Let us considered log⁡x = t

Now differentiating both side we get,

d(log⁡x) = dt

1/x dx = dt

dx = xdt

Then, put log⁡x = t and dx = xdt, we get

I = ∫ t/x × (x)dt

= ∫ tdt

= t²/2 + c

= (log⁡x)²/2 + c

Hence, I = (log⁡x)²/2 + c

问题2.∫(log⁡(1 + 1 / x))/(x(1 + x))dx

解决方案：

Given that I = ∫(log⁡(1 + 1/x))/(x(1 + x)) dx   ……(i)

Let us considered log⁡(1 + 1/x) = t then

On differentiating both side we get,

 d[log⁡(1 + 1/x)]=dt

1/(1 + 1/x) × (-1)/x²dx = dt

1/((x + 1)/x) × (-1)/x²dx = dt

(-x)/(x² (x + 1)) dx = -dt

dx/(x(x + 1)) = -dt

Now, on putting log⁡(1 + 1/x) = t and dx/(x(x + 1)) = -dt in equation (i), we get

I = ∫t × -dt

= -t²/2 + c

= -1/2 [log⁡(1 + 1/x)]² + c

Hence, I = -1/2 [log⁡(1 + 1/x)]² + c

问题3.∫(((1 +√x) ² )/√xdx

解决方案：

Given that I = ∫((1 + √x)²)/√x dx

 Let us considered (1 + √x) = t then, 

On differentiating both side we get,

d(1 + √x) = dt

1/(2√x) dx = dt

dx = dt × 2√x

Now on putting (1 + √x) = t and dx = dt × 2√x, we get

I = ∫t²/√x × dt × 2√x

= 2∫t²dt

= 2 × t³/3 + c

= 2/3[1 + √x]³ + c

Hence, I = 2/3(1 + √x)³ + c

问题4.∫√(1 + e ^x )e ^x d

解决方案：

Given that I = ∫√(1 + e^x) e^x dx  ……(i)

Let us considered 1 + e^x = t then, 

On differentiating both side we get,

d(1 + e^x) = dt

e^x dx = dt

dx = dt/e^x 

Now on putting 1 + e^x = t and dx = dt/e^x in equation (i), we get

I = ∫√t × e^x × dt/e^x

= ∫ t^1/2 dt

= 2/3t^3/2 + c

= 2/3 (1 + e^x)^3/2+c

问题5.∫∛(cos ² x)sin⁡xdx

解决方案：

Given that I = ∫∛(cos²x) sin⁡x dx    ……(i)

Let us considered cos⁡x = t then,

On differentiating both side we get,

d(cos⁡x) = dt

-sin⁡xdx = dt

dx = -dt/(sin⁡x))

Now on putting cos⁡x = t and dx = -dt/(sin⁡x) in equation (i), we get

I = ∫∛(t²) sin⁡x × (-dt)/(sin⁡x)

= -∫t^2/3 sin⁡x dt/(sin⁡x)

= -∫ t^2/3 dt

= -3/5 × t^5/3

Hence, I = -3/5(cos⁡x)^5/3 + c

问题6.∫E^的x /(1 + E ^X)2 DX

解决方案：

Given that I = ∫e^x/(1 + e^x)² dx   …….(i)

Let us considered 1 + e^x = t then,

On differentiating both side we get,

d(1 + e^x) = dt

e^x dx = dt

dx = dt/e^x 

Now on putting 1 + e^x = t and dx = dt/e^x in equation (i), we get

I = ∫e^x/t² × dt/e^x 

= ∫dt/t²

= ∫t^-2 dt

= -t^-1 + c

= -1/t + c

= -1/(1 + e^x) + c

 Hence, I = -1/(1 + e^x) + c

问题^{7.∫cot3⁡x}余割^2⁡xDX

解决方案：

Given that I = ∫cot³⁡x cosec²⁡x dx   …….(i)

Let us considered cot⁡x = t then,

On differentiating both side we get,

d(cotx) = dt

 -cosec²x dx = dt

dx = -dt/cosec²x

Now on putting cot⁡x = t and dx = -dt/(cosec²⁡x) in equation (i), we get

I = ∫ t³ cosec²x × (-dt)/(cosec²⁡x)

= -∫ t³ dt

= -t⁴/4 + c

= -(cot⁴⁡x)/4 + c

Hence, I = -(cot⁴⁡x)/4 + c

问题8。 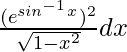$∫\frac{(e^{sin^{-1} x})^2}{\sqrt{1-x^2}} dx$

解决方案：

Given that I =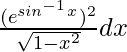$∫\frac{(e^{sin^{-1} x})^2}{\sqrt{1-x^2}} dx$[Tex] [/Tex] …….(i)

Let us considered sin^-1⁡x = t then,

On differentiating both side we get,

d(sin^-1x) = dt

1/√(1 – x²)dx = dt

dx = √(1 – x²) dt)

Now on putting sin^-1x = t and dx = √(1 – x²) dt in equation (i), we get

I = ∫ (e^t)²/√(1 – x²) × √(1 – x²) dt

= ∫e^2t dt

= e^2t/2 + c

= $\frac{e^{sin^{-1}x}}{2}$ + c

Hence, I = $\frac{e^{sin^{-1}x}}{2}$ + c

问题9.∫(1 +sin⁡x)/√(x-cos⁡x)dx

解决方案：

Given that I = ∫(1 + sin⁡x)/√(x – cos⁡x) dx    ……..(i)

Let us considered x – cos⁡x = t, then 

On differentiating both side we get,

d(x – cos⁡x) = dt

 [1 – (-sin⁡x)]dx = dt

 (1 + sin⁡x)dx = dt

Now on putting x – cos⁡x = t and (1 + sin⁡x)dx = dt in equation (i), we get

I = ∫ dt/√t

= ∫ t^-1/2 dt

= 2t^1/2 + c

= 2(x – cos⁡x)^1/2 + c

Hence, I = 2√(x – cos⁡x) + c

问题10。∫1/(√(1 – x ² )(sin ^-1 x) ² )dx

解决方案：

Given that I = ∫1/(√(1 – x²) (sin^-1⁡x)²) dx   …..(i)

Let us considered sin^-1⁡x = t then,

On differentiating both side we get,

 d(sin^-1⁡x) = dt

1/√(1 – x² ) dx = dt

Now on putting sin^-1⁡x = t and 1/√(1 – x²) dx = dt in equation (i), we get

I = ∫dt/t²  

= ∫t^-2 dt

= -t^-1 + c

= (-1)/t + c

= (-1)/(sin^-1⁡x) + c

Hence, I = (-1)/(sin^-1⁡x) + c

问题11.∫(cot⁡x)/√(sin⁡x)dx

解决方案：

Given that I = ∫(cot⁡x)/√(sin⁡x) dx    …….(i)

Let us considered sin⁡x = t then,

On differentiating both side we get,

d(sin⁡x) = dt

cos⁡xdx = dt

Now, I = ∫(cot⁡x)/√(sin⁡x) dx

 = ∫(cos⁡x)/(sin⁡x√(sin⁡x)) dx

 = ∫ cos⁡x/(sin⁡x)^3/2dx

 = ∫ cos⁡x/(sin⁡x)^3/2 dx   …….(ii)

Now on putting sin⁡x = t and cos⁡xdx = dt in equation (ii), we get

I = ∫ dt/t^3/2 

= ∫ t^-3/2 dt

= -2t^-1/2 + c

= -2/√t + c

= -2/√(sin⁡x) + c

I = -2/√sin⁡x + c

问题12.∫(tan⁡x)/√(cos⁡x)dx

解决方案：

Given that I = ∫(tan⁡x)/√(cos⁡x) dx

I = ∫sin⁡x/cos⁡x√(cos⁡x) dx

= ∫ sin⁡x/(cos⁡x)^3/2 dx

= ∫sin⁡x/(cos⁡x)^3/2 dx   ……..(i)

Let us considered cos⁡x = t then,

On differentiating both side we get,

d(cos⁡x) = dt

-sin⁡xdx = dt

sin⁡xdx = -dt

Now on putting cos⁡x = t and sin⁡xdx = -dt in equation (i), we get

I = ∫(-dt)/t^-3/2 

= -∫t^-3/2 dt

= -[-2t^-1/2] + c

= 2/t^1/2 + c

= 2/√(cos⁡x) + c)

Hence, I = 2/√(cos⁡x) + c

问题^{13∫cos3×/√(sin⁡x)DX}

解决方案：

Given that I = ∫cos³⁡x/√(sin⁡x) dx

= ∫(cos²xcos⁡x)/√(sin⁡x) dx

= ∫((1 – sin²⁡x)cos⁡x)/√(sin⁡x) dx

= ∫((1 – sin²x))/√(sin⁡x) cos⁡xdx ……(i)

Let us considered  sin⁡x = t then,

On differentiating both side we get,

d(sin⁡x) = dt

cos⁡xdx = dt

Now on putting sin⁡x = t and cos⁡xdx = dt in equation (i), we get

I = ∫(1 – t²)/√t dt

= ∫(t^-1/2-t² x t^-1/2)dt

=∫(t^-1/2 – t^3/2)dt

= 2t^1/2 – 2/5 t^5/2 + c

= 2(sin⁡x)^1/2 – 2/5(sin⁡x)^5/2 + c

Hence, I = 2√(sin⁡x) – 2/5(sin⁡x)^5/2 + c

问题14.∫(sin ³ x)/√(cos⁡x)dx

解决方案：

Given that I = ∫(sin³⁡x)/√(cos⁡x) dx

= ∫(sin²⁡xsin⁡x)/√(cos⁡x) dx

=∫((1 – cos²⁡x))/√(cos⁡x) sin⁡xdx …….(i)

Let us considered cos⁡x = t then,

On differentiating both side we get,

d(cos⁡x) = dt

-sin⁡xdx = dt

sin⁡xdx = -dt

Now on putting cos⁡x = t and sin⁡xdx = -dt in equation (i), we get

I = ∫((1 – t²))/√t × -dt

= ∫(t² – 1)/√t dt

= ∫(t²/t^1/2 – 1/t^1/2)dx

= ∫(t^2-1/2 – t^-1/2)dt

= ∫(t^3/2 – t^1/2)dt

= 2/5 t^5/2 – 2t^1/2 + c

= 2/5 cos^5/2⁡x – 2cos^1/2⁡x + c

Hence, I = 2/5 cos^5/2x – 2√(cos⁡x) + c

问题15.∫1/(√(tan ^-1 x)(1 + x ² ))dx

解决方案：

Given that I = ∫1/(√(tan^-1x) (1 + x²)) dx  …..(i)

Let us considered tan^-1⁡x = t, then    

On differentiating both side we get,

d(tan^-1⁡x) = dt

1/(1 + x²) dx = dt

Now on putting tan^-1⁡x = t and 1/(1 + x²) dx = dt in equation (i), we get

I = ∫1/√t dt

= ∫t^-1/2 dt

= 2t^1/2 + c

= 2√tan^-1⁡x + c

Hence, I = 2√tan^-1⁡x + c

问题16.∫√(tan⁡x)/(sin⁡xcos⁡x)dx

解决方案：

Given that I = ∫√(tan⁡x)/(sin⁡xcos⁡x) dx

= ∫(√(tan⁡x)×cos⁡x)/(sin⁡xcos⁡x×cos⁡x) dx

= ∫√(tan⁡x)/(tan⁡xcos²⁡x) dx

= ∫(sec²⁡xdx)/√(tan⁡x) dx

Let us considered tan⁡x = t, then

On differentiating both side we get,

sec²⁡xdx = dt

Now

I = ∫ dt/√t

= 2√t + c

Hence, I = 2√tan⁡x + c

问题17.1 / x×(log⁡x) ² dx

解决方案：

Given that I = ∫1/x × (log⁡x)² dx   …..(i)

Let us considered log⁡x = t then,

On differentiating both side we get,

d(log⁡x) = dt

1/x dx = dt

Now on putting log⁡x = t and 1/x dx = dt in equation (i), we get

I = ∫t² dt

= t³/3 + c

= (log⁡x)³/3 + c

Hence, I = (log⁡x)³/3 + c

问题^{18∫sin5×cos⁡xDX}

解决方案：

Given that I = ∫sin⁵⁡x cos⁡x dx  ……(i)

Let us considered sin⁡x = t then,

On differentiating both side we get,

d(sin⁡x) = dt

cos⁡xdx = dt

Now on putting sin⁡x = t and cos⁡xdx = dt in equation (i), we get

I = ∫ t⁵ dt

= t⁶/6 + c

= (sin⁶⁡x)/6 + c

Hencec, I = 1/6 (sin⁶⁡x) + c

问题^{19∫tan3/2⁡x}秒^2⁡xDX

解决方案：

Given that I = ∫tan^3/2⁡xsec²⁡xdx  ……(i)

Let us considered tan⁡x = t then,

On differentiating both side we get,

d(tan⁡x) = dt

sec²⁡xdx = dt

Now on putting tan⁡x = t and sec²xdx = dt in equation (i), we get

I = ∫ t^3/2 dt

= 2/5 t^5/2 + c

= 2/5(tan⁡x)^5/2 + c

Hence, I = 2/5 tan^5/2⁡x + c

问题20.∫(x ³ )/(x ² +1) ² dx

解决方案：

Given that I = ∫(x³)/(x² + 1)²dx …….(i)

Let us considered 1 + x² = t then,

On differentiating both side we get,

d(1 + x²) = dt

2xdx = dt

xdx = dt/2

Now on putting 1 + x² = t and xdx = dt/2 in equation (i),we get 

I = ∫x²/t³ × dt/2

= 1/2∫(t – 1)/t³ dt         [1 + x² = t]

= 1/2∫[(t/t³ – 1/t³)dt]

= 1/2∫(t^-2 – t^-3)dt

= 1/2 [-1t^-1 – t^-2/(-2)] + c

= 1/2 [-1/t + 1/(2t²)] + c

= -1/2t + 1/(4t²) + c

= -1/2(1 + x²) + 1/(4(1 + x²)²) + c

= (-2(1 + x²) + 1)/(4(1 + x²)²) + c

= (-2 – 2x² + 1)/(4(1 + x²)²) + c

= (-2x² – 1)/(4(1 + x²)²) + c

= -(1 + 2x² )/(4(x² + 1)²) + c

Henec, I = -(1 + 2x²)/(4(x² + 1)²) + c

问题21.∫(4x + 2)√(x ² + x + 1)dx

解决方案：

Given that I = ∫(4x + 2)√(x² + x + 1) dx

Let us considered x² + x + 1 = t then,

On differentiating both side we get,

(2x + 1)dx = dt

Now,

I = ∫ (4x + 2)√(x² + x + 1) dx

= ∫2√t dt

= 2∫√t dt

= 2t^3/2/(3/2) + c

Hence, I = 4/3 (x² + x + 1)^3/2 + c

问题22.∫(4x + 3)/√(2x ² + 3x + 1)dx

解决方案：

Given that l = ∫(4x + 3)/√(2x² + 3x + 1) dx  ……(i)

Let us considered 2x² + 3x + 1 = t then,

On differentiating both side we get,

d(2x² + 3x + 1) = dt

(4x + 3)dx = dt

Now on putting 2x² + 3x + 1 = t and (4x + 3)dx = dt in equation (i), we get

I = ∫dt/√t

= ∫t^-1/2 dt

= 2t^1/2 + c

= 2√t + c

Hence, I = 2√(2x² + 3x + 1) + c

问题23.∫1/(1 +√x)dx

解决方案：

Given that I = ∫1/(1 + √x) dx   …….(i)

Let us considered x = t² then,

On differentiating both side we get,

dx = d(t²)

dx = 2tdt

Now on putting x = t² and dx = 2tdt in equation (i), we get

I = ∫2t/(1 + √(t²)) dt

= ∫2t/(1 + t) dt

= 2∫t/(1 + t) dt

= 2∫(1 + t – 1)/(1 + t) dt

= 2⌋[(1 + t)/(1 + t) – 1/(1 + t)]dt

= 2∫dt – 2∫1/(1 + t) dt

= 2t – 2log⁡|(1 + t)| + c

= 2√x – 2log⁡|(1 + √x)| + c

Hence, I = 2√x – 2log⁡|(1 + √x)| + c

问题24。 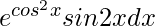$∫e^{cos^{2}x} sin⁡2xdx$

解决方案：

Given that I = $∫e^{cos^{2}x} sin⁡2xdx$ …….(i)

Let us considered cos²x = t then,

On differentiating both side we get,

d(cos²⁡x) = dt

-2cos⁡x sin⁡x dx = dt

-sin⁡2x dx = dt

sin⁡2x dx = -dt

Now on putting cos²⁡x = t and sin⁡2x dx = -dt in equation (i), we get

I = ∫e^t(-dt)

= -e^t + c

= –$e^{cos^{2}x}$ + c

Hence, I = –$e^{cos^{2}x}$ + c