方向余弦和方向比率

通常，对于三维几何，我们依赖于三维笛卡尔平面。向量也可以用来描述直线和它们与轴所成的角度。我们应该如何描述一条穿过原点并与不同轴成角度的线?我们使用线的余弦比定义它们。在处理三维几何(在许多应用程序，例如游戏设计中使用)时，需要描述3D空间中直线的重要性。

直线的方向余弦和方向比率

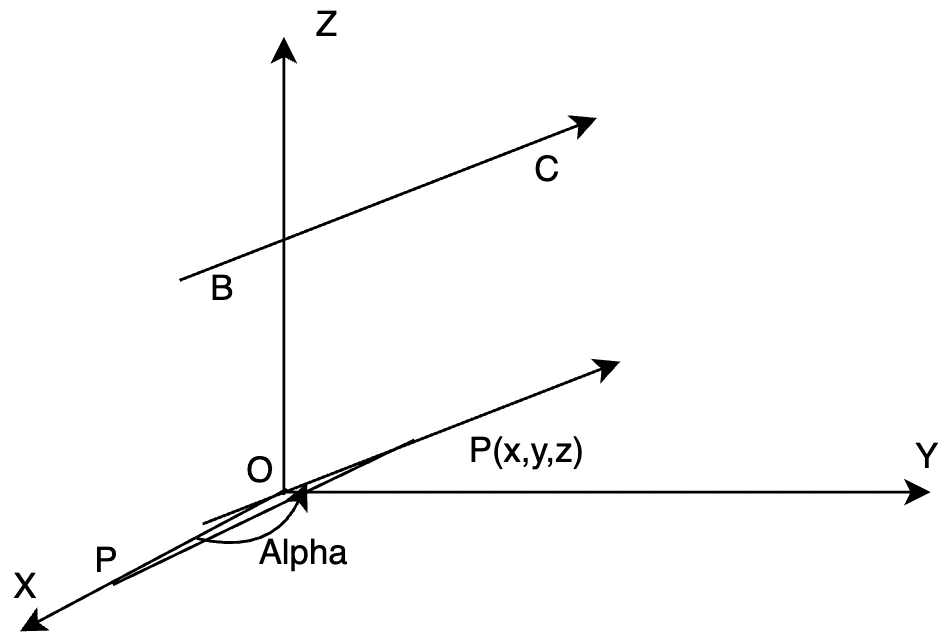
假设一条直线OA穿过原点，并分别与x，y和z轴形成角度α，β和γ，称为方向角。这些角度cos(α)，cos(β)和cos(γ)的余弦称为有向线OA的有向余弦。下图显示了穿过原点的OA线及其与坐标轴的所有角度。

Note: The line can extend in both the directions, and there can be two sets of angles that it makes with coordinate axes. The line makes α, β, and γ and their supplements $\pi - \alpha, \pi -\beta$, and $\pi - \gamma$ with the coordinate axes. Now the signs of the directional cosines will be reversed. So, to have unique directional cosines, we choose to make it a directed line. 

假设直线的方向余弦为l，m和n。

方向比

与线的方向余弦成比例的数字称为线的方向比率。我们假设l，m和n为线的方向余弦。假设a，b和c是线的方向比。然后，

l = k × a, m = k × b and n = k × c

$\frac{l}{a} = \frac{m}{b} = \frac{n}{c} = k$

Where k is any constant. 

现在我们知道了

l ² + m ² + n ² = 1

⇒k^{图2(a 2} + B ² + C ²⁾⁼ 1

⇒k = $\pm \frac{1}{a^2 + b^2 + c^2}$

所以，现在一条线的方向余弦是

l = $\pm \frac{a}{a^2 + b^2 + c^2}$ ，m = $\pm \frac{b}{a^2 + b^2 + c^2}$和n = $\pm \frac{c}{a^2 + b^2 + c^2}$

方向余弦之间的关系

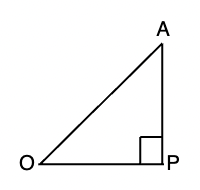
假设我们有一条线BC，它的方向余弦为l，m和n。通过与该线平行的原点绘制一条线，然后让A(x，y，z)在该线上成为一点。我们从A到x轴绘制一个垂直线。

令OA = r。

$cos(\alpha) = \frac{OA}{OP} = \frac{x}{r}$

So, x = lr and similarly, y = mr and z = nr. 

x² + y² + z² = r²(l² + m² + n²) 

We know that distance of (x, y,z) from origin is r. 

x² + y² + z² = r²

Thus,

l² + m² + n²  = 1

穿过两个点的线的方向余弦

想象一下，我们有两个点A(x ₁ ，y ₁ ，z ₁ )和B(x ₂ ，y ₂ ，z ₂ )，一条线穿过它们。我们的目标是确定穿过这些点的线的方向余弦。令l，m和n为线AB的方向余弦，并假设它们与x，y和z轴成的角度分别为α，β和γ。

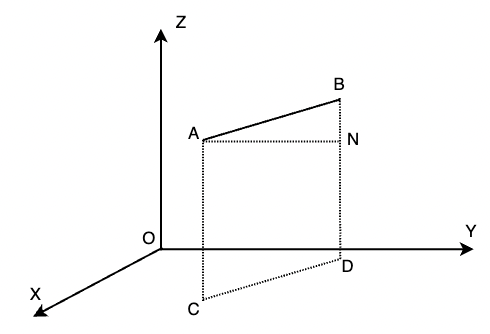
在上图中，从线AB到XY平面绘制了垂直线，并且它们在CD处到达XY平面。应该从A到与BD和N相交的BD绘制另一个垂直线。因此，现在在三角形ANB中，∠ABN是γ。

$cos(\gamma) = \frac{BN}{AB} = \frac{z_2 - z_1}{AB}$

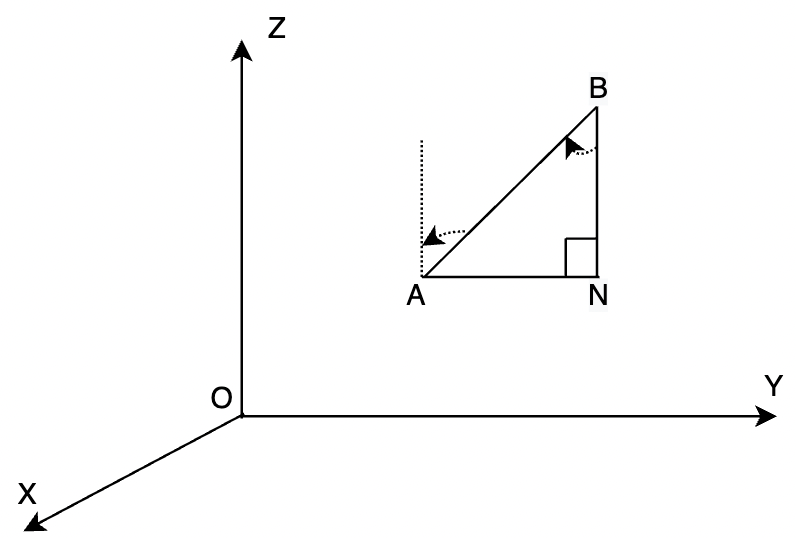
用同样的方式

$cos(\alpha) = \frac{x_2 - x_1}{AB}$和$cos(\beta) = \frac{y_2 - y_1}{AB}$

因此，连接AB的线的方向余弦为，

$\frac{x_2 - x_1}{AB}, \frac{y_2 - y_1}{AB}, \frac{z_2 - z_1}{AB}$

在这里，AB = $\sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2 + (z_2 - z_1)^2}$

让我们看看基于这些概念的一些示例问题，

样本问题

问题1：找到x，y和z轴的方向余弦。

解决方案：

The x-axis makes an angle of 90°, 90° and 0° with the coordinate axes. We know that the direction cosines are basically cosines of the angle the line makes with different axes. 

l = cos(0°) m = cos(90°) and n = cos(90°)

Thus, 

l = 1, m = n = 0  

Similarly, for y-axis, 

l = 0, m = 1 and n = 0 

and for z-axis 

l = 0, m = 0 and n = 1. 

问题2：找到分别与x，y和z轴成30°，60°和90°的直线的方向余弦。

解决方案：

Directional cosines for the given line are, 

l = cos(30°) ; m = cos(60°) and n = cos(90°) 

⇒ l = $\frac{\sqrt{3}}{2}$ ; m = $\frac{1}{2}$ and n = 0

问题3：假设我们有一条线的比率为4、2，-4。计算这条线的方向余弦。

解决方案：

Given direction ratios: 4, 2, and -4. 

The formula for directional cosines from direction ratios is given by, 

l =$\frac{a}{\sqrt{a^2 + b^2 + c^2}}$, m =$\frac{b}{\sqrt{a^2 + b^2 + c^2}}$ and n = $\frac{c}{\sqrt{a^2 + b^2 + c^2}}$

Here, a = 4, b = 2 and c =-4. 

l = $\frac{4}{\sqrt{4^2 + 2^2 + (-4)^2}}$, m =$\frac{2}{\sqrt{4^2 + 2^2 + (-4)^2}}$  and n = $\frac{-4}{\sqrt{4^2 + 2^2 + (-4)^2}}$

l = \frac{4}{\sqrt{36}} 

m = $\frac{2}{\sqrt{36}} = \frac{2}{6} = \frac{1}{3}$

n = $\frac{2}{\sqrt{4^2 + 2^2 + (-4)^2}} = \frac{2}{\sqrt{36}} = \frac{2}{6}$

问题4：假设我们有一条线的方向比率为0、1、0。计算该线的方向余弦值。

解决方案：

Given direction ratios: 0, 1, and 0. 

The formula for directional cosines from direction ratios is given by, 

l =$\frac{a}{\sqrt{a^2 + b^2 + c^2}}$, m =$\frac{b}{\sqrt{a^2 + b^2 + c^2}}$ and n = $\frac{c}{\sqrt{a^2 + b^2 + c^2}}$

Here, a = 0, b = 1 and c =0. 

l = $\frac{0}{\sqrt{0^2 + 1^2 + 0^2}}$, m =$\frac{1}{\sqrt{0^2 + 1^2 + (0)^2}}$  and n = $\frac{0}{\sqrt{0^2 + 1^2 + 0^2}}$

l = 0

m = 1

n = 0

问题5：找到通过(1、1、1)和(3、3、3)的线的方向余弦。

解决方案：

Let’s say these points are A(1,1,1) and B(3,3,3).  We know for any two points on the line AB, the direction cosines are given by, 

$\frac{x_2 - x_1}{AB}, \frac{y_2 - y_1}{AB}, \frac{z_2 - z_1}{AB}$

Here, AB = $\sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2 + (z_2 - z_1)^2}$

Here, (x₁,y₁,z₁) = (1,1,1) and (x₂,y₂,z₂) = (3,3,3) 

AB = $\sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2 + (z_2 - z_1)^2}$

      = $\sqrt{(3-1)^2 + (3 - 1)^2 + (3 -1)^2}$

      = $\sqrt{4+ 4 + 4}$

      = $\sqrt{12}$

      = 2√3

$\frac{3-1}{AB}, \frac{3 - 1}{AB}, \frac{3 - 1}{AB}$

⇒ $\frac{3-1}{2\sqrt{3}}, \frac{3 - 1}{2\sqrt{3}}, \frac{3 - 1}{2\sqrt{3}}$

⇒$\frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}$