在本文中,我们将探索隐式微分的应用,以找到反三角函数的导数。但是在前进之前,让我们回顾一下隐式微分和逆三角学的概念。
反三角学
三角函数的反函数是三角比的反函数,即sin,cos,tan,cot,sec,cosec。这些功能广泛用于物理,数学,工程和其他研究领域。就像加法和减法是彼此的反函数一样,三角函数的反函数也是如此。
sin θ = x
⇒ θ = sin−1x
反三角函数的表示
它们通过在前缀中添加弧线或在功率上加上-1来表示。
Inverse sine can be written in two ways:
- sin-1 x
- arcsin x
Same goes for cos and tan.
Note: Don’t confuse sin-1 x with (sin x)-1. They are different. Writing sin-1 x is a way to write inverse sine whereas (sin x)-1 means 1/sin x.
隐分化
隐式微分是一种利用链规则来区分隐式定义的函数的方法。显式地找到函数然后进行区分通常并不容易。相反,我们可以完全微分f(x,y),然后求解方程的其余部分以找到f’(x)的值。即使可以明确求解原始方程式,总的来说,由全微分得出的公式也更简单易用。让我们区分一些反三角函数。
示例1:区分正弦-1 (x)?
解决方案:
Let,
y = sin−1 (x)
Taking sine on both sides of equation gives,
![]()
By the property of inverse trigonometry we know, ![]()
![]()
Now differentiating both sides wrt to x,
![]()
![]()
![]()
We can simplify it more by using the below observation:
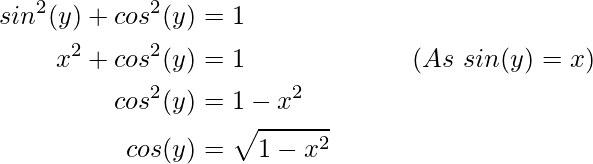
Substituting the value, we get
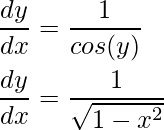
示例2:区分cos -1 (x)?
解决方案:
Let,
y = cos−1 (x)
Taking cosine on both sides of equation gives,
![]()
By the property of inverse trigonometry we know, ![]()
![]()
Now differentiating both sides wrt to x,
![]()
![]()
![]()
We can simplify it more by using the below observation:

Substituting the value, we get
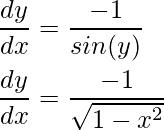
示例3:区分tan -1 (x)?
解决方案:
Let,
y = tan−1 (x)
Taking tan on both sides of equation gives,
![]()
By the property of inverse trigonometry we know,![]()
![]()
Now differentiating both sides wrt to x,
![]()
![]()
![]()
We can simplify it more by using the below observation:

Substituting the value, we get
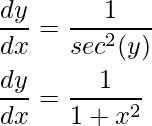
反三角函数微分的一些高级示例
示例1:y = cos -1 (-2x 2 )。在y = 1/2处找到dy / dx?
解决方案:
方法1(使用隐式微分)
Given,
y = cos−1 (−2x2)
⇒ cos y = −2x2
Differentiating both sides wrt x
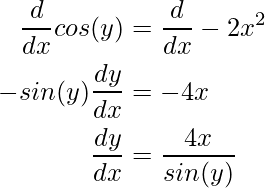
Simplifying
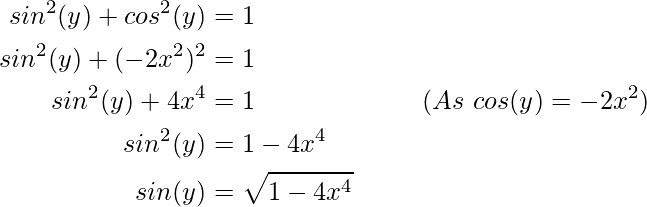
Putting the obtained value we get,

方法2(使用链规则,因为我们知道arccos x的微分)
Given,
y = cos−1 (−2x2)
Differentiating both sides wrt x

示例2:区分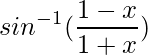 ?
?
Let,
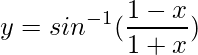
Differentiating both sides wrt x
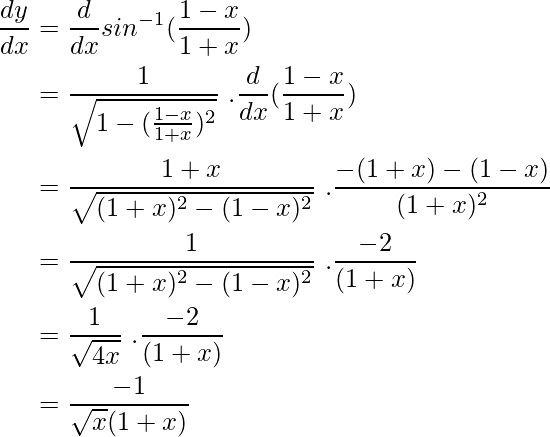
逆三角函数的导数表
|
Function |
Derivative |
|---|---|